Exercises: 1.3 Circles

1.

Leanne is sailing and is currently [latex]3[/latex] miles west and [latex]5[/latex] miles south of the harbor. She heads directly toward an island that is [latex]8[/latex] miles west and [latex]7[/latex] miles north of the harbor. How far is Leanne from the island?

2.

Dominic is [latex]100[/latex] meters east and [latex]250[/latex] meters north of Kristen. He is walking directly toward a tree that is [latex]220[/latex] meters east and [latex]90[/latex] meters north of Kristen. How far is Dominic from the tree?

3.

4.

5.

6.

7.

[latex](1,1),(4,5)[/latex]

8.

[latex](-1,1),(5,9)[/latex]

9.

[latex](2,-3),(-2,-1)[/latex]

10.

[latex](5,-4),(-1,1)[/latex]

11.

[latex](3,5),(-2,5)[/latex]

12.

[latex](-2,-5),(-2,3)[/latex]

13.

Sketch a triangle with vertices [latex](10,1),(3,1),(5,9)[/latex] and find its perimeter. Round your answer to tenths.

14.

Sketch a triangle with vertices [latex](-1,5), (8,-7), (4,1)[/latex] and find its perimeter. Round your answer to tenths.

15.

1. Write an expression for the distance between the points [latex](x,y)[/latex] and [latex](-3,4).[/latex]
2. Write an equation that says “the distance between the points [latex](x,y)[/latex] and [latex](-3,4)[/latex] is [latex]5[/latex] units.”

16.

1. Write an expression for the distance between the points [latex](-6,-1)[/latex] and [latex](h,k).[/latex]
2. Write an equation that says “the point [latex](-6,-1)[/latex] is [latex]3[/latex] units from the point [latex](h,k)[/latex]“

17.

[latex]\sqrt{(x-4)^{2}+(y+1)^{2}}=3[/latex]

18.

[latex]\sqrt{(-2-h)^{2}+(5-k)^{2}}=l[/latex]

19.

How long is the diagonal of a square whose side is [latex]6[/latex] centimeters?

20.

How long is the side of a cube whose volume is [latex]80[/latex] cubic feet?

21.

What is the area of a circle whose radius is [latex]5[/latex] inches?

22.

What is the circumference of a circle whose radius is [latex]5[/latex] meters?

23.

1. [latex]\dfrac{\sqrt{3}}{2} \rightarrow 0.866025[/latex]
2. [latex]\dfrac{\sqrt{16}}{3} \rightarrow 1.333333[/latex]
3. [latex]\dfrac{3\pi}{2} \rightarrow 4.712390[/latex]
4. [latex]\dfrac{5}{7} \rightarrow \overline{0.714285}[/latex]

24.

1. [latex]\dfrac{\pi}{6} \rightarrow 0.52536[/latex]
2. [latex]\dfrac{5}{32} \rightarrow 0.15625[/latex]
3. [latex]\dfrac{\sqrt{196}}{32} \rightarrow 0.4375[/latex]
4. [latex]\dfrac{\sqrt{8}}{4} \rightarrow 0.707107[/latex]

25.

1. Complete the table of values for the equation [latex]x^2 + y^2 = 25[/latex].
   

   [latex]x[/latex]	[latex]-5[/latex]	[latex]-4[/latex]	[latex]-3[/latex]	[latex]-2[/latex]	[latex]-1[/latex]	[latex]0[/latex]	[latex]1[/latex]	[latex]2[/latex]	[latex]3[/latex]	[latex]4[/latex]	[latex]5[/latex]
   [latex]y[/latex]

2. Plot the points and graph the equation.

26.

1. Complete the table of values for the equation [latex]x^2 + y^2 = 1[/latex].
   

   [latex]x[/latex]	[latex]-1[/latex]	[latex]-0.8[/latex]	[latex]-0.6[/latex]	[latex]0[/latex]	[latex]0.6[/latex]	[latex]0.8[/latex]	[latex]1[/latex]
   [latex]y[/latex]

2. Plot the points and graph the equation.

27.

1. Sketch a graph of all points in the plane that lie [latex]6[/latex] units from the origin.
2. Write an equation for your graph.

28.

1. Sketch a graph of all points in the plane that lie [latex]\sqrt{12}[/latex] units from the origin.
2. Write an equation for your graph.

29.

1. Sketch a graph of all points in the plane that lie less than [latex]3[/latex] units from the origin.
2. Write an inequality for your graph.

30.

1. Sketch a graph of all points in the plane that lie more than [latex]5[/latex] units from the origin.
2. Write an inequality for your graph.

31.

1. Explain why the solutions of the equation [latex]x^2 +y^2 = 16[/latex] must have [latex]-4 \leq x \leq 4.[/latex]
2. What does part (a) tell you about the graph of the equation?

32.

1. Explain why the solutions of the equation [latex]x^2 +y^2 = 100[/latex] must have [latex]-10 \leq x \leq  10[/latex]
2. What does part (a) tell you about the graph of the equation?

33.

The point [latex](1,-3)[/latex] lies on a circle centered at the origin. What is the radius of the circle?

34.

The point [latex](-2,\sqrt{6})[/latex]lies on a circle centered at the origin. What is the radius of the circle?

35.

[latex]x^2 + y^2 = 36[/latex]

36.

[latex]x^2 + y^2 = 16[/latex]

37.

[latex]4x^2 +4y^2 = 16[/latex]

38.

[latex]2x^2 + 2y^2 = 18[/latex]

39.

Give the coordinates of two points on the circle in Problem 35 that have [latex]y=-4[/latex]. Plot those points on your graph.

40.

Give the coordinates of two points on the circle in Problem 35 that have [latex]x=-2[/latex]. Plot those points on your graph.

41.

42.

43.

A circular herb garden has diameter [latex]40[/latex] feet and is divided into [latex]8[/latex] equal sectors.

1. What is the central angle of each sector?
2. What is the length of the circular edge of each sector?
3. What is the area of each sector?

44.

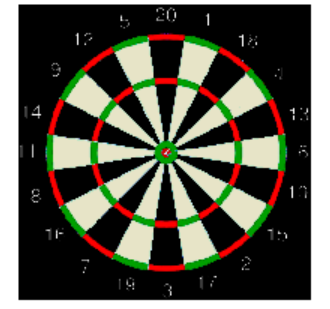
A dart board is [latex]18[/latex] inches in diameter, divided into [latex]20[/latex] sectors of equal size. (For this exercise, we will ignore the bulls-eye and the fact that the sectors are further subdivided.)

1. What is the central angle of each sector?
2. What is the length of the circular edge of each sector?
3. What is the area of each sector?

45.

46.

47.

48.

49.

50.

51.

South America stretches across [latex]30^o[/latex] of longitude at the equator, from Quito in Ecuador to the east coast of Brazil. (The figure at right shows a view of the earth from above the North Pole.) The radius of the earth is about [latex]3960[/latex] miles. How wide is South America at the equator?

52.

The radius of the earth is about [latex]3960[/latex] miles. What distance will you cover if you travel north by one degree of latitude? (See the figure at right.) There is [latex]180^o[/latex] of latitude from the South Pole to the North Pole.

53.

The moon is about [latex]240,000[/latex] miles from earth. It moves in a roughly circular orbit, completing one revolution in [latex]28[/latex] days.

1. How far does the moon move around the earth in one day?
2. What is the speed of the moon relative to the earth, in miles per hour?

54.

The earth is about [latex]93,000,000[/latex] miles from the sun, and its orbit is approximately circular.

1. How far does the earth move around the sun in one month?
2. What is the speed of the earth relative to the sun, in miles per day? (Assume a month has [latex]30[/latex] days.)

55.

1. Use the distance formula to write an equation for the circle of radius [latex]6[/latex] centered at the point [latex](3,-2).[/latex]
2. Use the distance formula to derive an equation for the circle of radius [latex]r[/latex] centered at the point [latex](h,k).[/latex]

56.

Find the coordinates of the indicated points on each circle.

1.  [latex](x-4)^2 + (y-5)^2 = 9)[/latex]
2.  [latex](x+3)^2 + (y-1)^2 = 25[/latex]