78 Exploring the Arts Chapter 18: Elements of Dance
Learning Objectives
- Recall the Elements of Dance
- Distinguish between the Elements of Dance
- Analyze, identify, and describe the Elements of Dance
| “A dance, as a work of art, must be constructed as well as a beautiful building…. A dance must have a beginning, development, and climax – just as a building has foundations, walls, and roof.” -Ted Shawn |
What are the Elements of Dance?
The Elements of Dance are the basic building blocks of dance that help us identify and describe movement, assisting in the ability to analyze, interpret and speak/write about dance as an artistic practice. When viewing dance, we want to put into words what we are witnessing by analyzing its most important qualities. The elements of the dance provide us with the tools to do so.
In dance, the body can be in constant motion and even arrive at points of stillness. However, even in stillness, the dancers are inherently aware of themselves. No matter the case, all forms of dance can be broken down into their primary elements: BODY, ENERGY, SPACE, and TIME. To easily remember the dance elements, we use the acronym: B. E. S. T., which stands for BODY, ENERGY, SPACE, and TIME. Dance can be seen as the use of the BODY with different kinds of ENERGY moving through SPACE and unfolding in TIME.
Let’s take a quick look at the elements of dance before we dig in further.
Watch This
Randy Barron, Teaching Artist on the Kennedy Center’s National Roster, made this video to explain the Elements of Dance:
Body
The body is the dancer’s instrument of expression. When an audience looks at dance, they see the dancer’s body and what is moving. The dance could be made up of a variety of actions and still poses. It could use the whole body or emphasize one part of the body. Exploring body shapes and movement actions increases our awareness of movement possibilities.

Body Shapes
The choreographer who is designing a dance may look at their dancers as sculpture. They choose shapes for the dancers to make with their bodies. These can be curved, straight, angular, twisted, wide, narrow, symmetrical, or asymmetrical. These shapes can be geometric designs, such as circles or diagonals. They could make literal shapes such as tree branches or bird wings. They can also make conceptual shapes (abstract) such as friendship, courage, or sadness. Sometimes a choreographer emphasizes the negative space or the empty area around the dancers’ bodies instead of just the positive space the dancer occupies. Look at the positive and negative spaces in Fig 2.

Body Moves/Actions
Dance movements or actions fall into two main categories:
Locomotor: (traveling moves) walk, run, jump, hop, skip, leap, gallop, crawl, roll, etc.
Nonlocomotor: (moves that stay in place) melt, stretch, bend, twist, swing, turn, shake, stomp, etc.
Below is an example of body movements and shapes by Modern Dance choreographer Paul Taylor.
Watch This
Body Parts
Each part of the body (head, shoulders, elbows, hips, knees, feet, eyes, etc.) can move alone (isolated) or in combination. In the classical Indian dance form Bharatanatyam, dancers stomp their feet in a percussive rhythm. At the same time, the dancer performs hand gestures, known as mudras, codified hand gestures that are important in the storytelling aspect of Bharatnatyam to communicate words, concepts, or feelings.
Observe in the video below, how the dancer alternately emphasizes her feet and legs with her hand and arm gestures. In Classical Indian dance forms, facial expressions and hand gestures play an important role in storytelling.
Watch This
Excerpt from Pushpanjali, choreographer Savitha Sastry performs a Classical Indian dance solo called Bharatanatyam. Observe how the dancer alternately emphasizes their feet and legs with hand and arm gestures.
In the next video, dancers are participating in the GAGA technique developed by Isralei choreographer Ohad Naharin. In this movement language, dancers are directed to listen to their inner sensations to elicit physical responses; movement. Notice how the dancers are integrating the entire body to create fluid and successive movement.
Check Your Understanding
Energy
An exploration of “how” a movement is done rather than “what” it is, gives us a richer sense of dance as an expressive art. A dancer can walk, reach for an imaginary object and turn, making these movements look completely different by changing the use of Energy. For example, anger could be shown with a loud quick walk, a sharp reach, and a strong twisting turn. Happiness could be depicted by using a delicate gliding walk, a gentle reach out, and a smooth light turn. Energy is what brings the dancer’s intent or emotion to the audience. The element of Energy is sometimes called Efforts or Movement Qualities.
Dancer and movement analyst Rudolf Laban broke it down into four Efforts, each of which is a pair of opposites:
- Space (direct or indirect use of space) When the dancer is paying attention to the use of space, they can be direct, single-focused, and targeted in their use of space. Conversely, they can be indirect, multi-focused, and aware of many things in the space around them.
- Weight or force (strong or light use of weight) The dancer can emphasize the effort or use of force by fighting against it, throwing their weight and strength into movements. The opposite is using a yielding, light sense of weightlessness in their movements.
- Time (sudden or sustained use of time) Not to be confused with tempo, the dancer’s use of time can be reflected in their movement. It can appear hurried, as though fighting against time. Conversely, the dancer can have a relaxed attitude toward time as though they have all the time in the world.
- Flow (bound or free use of the flow of movement) When the dancer’s flow is bound up, they can appear to be careful and cautious, only allowing small amounts of flow. The opposite is when the dancer appears to throw the movement around without inhibition, letting the movement feel carefree.
Another way we can define energy is by looking at the Movement Qualities. Movement Qualities are energy released during various time spans to portray distinct qualities. There are six dynamic Movement Qualities.
- Sustained (slow, smooth, continuous)
- Percussive (sharp, choppy, jagged)
- Swinging (swaying, to and fro, pendulum-like)
- Suspended (a moment of stillness, the high point, a balance)
- Collapsed (fall, release, relax)
- Vibratory (shake, wiggle, tremble)
Notice the kinds of Energy the dancers are displaying in the examples below.
In the first video the dancers are using Efforts of direct, strong, sudden and bound movements. In terms of Movement Qualities the dancers are using percussive, vibratory, and moments of collapse.
Watch This
Hip Hop dance crew Kaba Modern uses the Efforts of direct, strong, sudden, and bound movements. In terms of Movement Qualities, the dancers use percussive, vibratory, and moments of collapse.
In the National Opera of Ukraine’s preclude from Chopiniana, the dancers are using Efforts of light and free. The Movement Qualities are sustained and suspended.
Check Your Understanding
Space
Let’s look at where the dance takes place. Is the dance expansive, using lots of space, or is it more intimate, using primarily personal space? An exploration of space increases our awareness of the visual design aspects of movement.
- Personal Space: The space around the dancer’s body can also be called near space. A dance primarily in personal space can give a feeling of introspection or intimacy.
- Negative Space/Positive Space: Sometimes, a choreographer emphasizes the negative space or the empty area around the dancers’ bodies instead of just the positive space the dancer occupies. Look at the positive and negative space in the photograph below.
- General Space: The defined space where the dancer can move can be a small room, a large stage, or even an outdoor setting.
- Levels: Dancers use a variety of levels: high, middle, or low. High movements can reach upward using jumps, leaps, or when lifting each other. A middle level move is generally a move that takes place between the height of the dancer’s shoulders and knees. Low level moves can include sitting, kneeling, sinking to the ground, rolling, or crawling.

Fig 5. Français : Épreuve gélatino-argentique 1925 24,5 x 21 cm 18 May 2017 Mathildemultiple. CC Attribution-Share Alike 4.0 International license. - Directions: While dances made for the camera often have the performers facing forward as they dance, they can also change directions by turning, going to the back, right, left, up, or down.
- Pathways or Floor Patterns: Where the dancer goes through space is often an important design element. They can travel in a circle, figure eight, spiral, zig-zag, straight lines, and combinations of lines.
- Range: Movements or shapes can be near reach, mid-ranged, or far reach. Range is associated with one’s kinesphere. Kinesphere is the immediate area surrounding the body and is described as a three-dimensional volume of space. Imagine a bubble around the dancer’s body, with their arms and limbs extended to their fullest extent in every possible direction without moving from a fixed spot, this is considered a personal kinesphere. Movement occurs in a person’s kinesphere and includes near reach (movement that is close to the body, small or condensed), mid-reach (movement that is neither near not far, but comfortably in the middle ), and far reach (large and expansive movement).

Fig 5. Français : Épreuve gélatino-argentique 1925 24,5 x 21 cm 18 May 2017 Mathildemultiple. CC Attribution-Share Alike 4.0 International license. Fig 5. Français : Épreuve gélatino-argentique 1925 24,5 x 21 cm 18 May 2017 Mathildemultiple. CC Attribution-Share Alike 4.0 International license.
- Relationship: Dancers can explore the relationship between different body parts; the relationship of one dancer to another dancer or a group of dancers; or the relationship to a prop or to objects in the dance space.

Fig. 6. Egyptian Modern Dance Theatre in performance “devil hope.” 27 September 2016, 21:43:59 Esraa abd el khalla. CC Attribution-Share Alike 4.0 International license.
Watch This
Excerpt from George Balanchine’s ballet Apollo. Notice the interlocking of circles of the dancers’ arms and the straight lines made by the dancers’ legs.
In this next video, notice various floor patterns such as circular pathways and straight lines that are made by the group of dancers. Observe the dancers’ use of gestures that go from near to far reach from personal space to filling the general space. The choreography also uses levels from low to high.
Check Your Understanding
Time
Dance is a time art; movement develops and reveals itself in time. Adding a rhythmic sense to movement helps transform ordinary movement into dance and informs when the dancer moves.
- Pulse: The basic pulse or underlying beat
- Speed (tempo): Fast, moderate, slow
- Rhythm Pattern: A grouping of long or short beats, accents or silences
- Natural Rhythm: Timing which comes from the rhythms of the breath, the heartbeat, or natural sources like the wind or the ocean.
- Syncopation: Accents the off-beat in a musical phrase.
Compare the different uses of Time in the two videos below.
In the first video, the dancers have no musical accompaniment and use their breath to initiate movement and cue each other for the timing. Their movement is also slow to moderate tempo and imitates the natural rhythm of the ocean.
Watch This
Excerpt from Modern Dance choreographer Doris Humphrey’s Water Study. In this video, the dancers have no musical accompaniment and use their breath to initiate movement and cue each other for the timing. Their movement is also slow to moderate tempo and imitates the natural rhythm of the ocean.
Promo clip of Step Afrika!, the dancers are creating rhythm patterns with body percussion. There is an emphasis on syncopation and varying tempos with accents.
Summary
All dance forms share foundational concepts known as the Elements of Dance. The Elements of Dance are overarching concepts and terminology that are useful when observing, creating, analyzing, and discussing dance. Dance can be broken down into its primary elements : Body, Energy, Space, and Time. It can be easily recalled through the acronym B.E.S.T.
The Body is the mobile instrument of the dancer and helps inform us what is moving. The Body category includes shapes, actions, whole body, and part body movements. Energy is how the body moves. When speaking about energy, we can refer to effort or movement qualities. Space is where movement occurs and includes personal and general space, levels, directions, pathways and floor patterns, various sizes of movements, range of movement, and relationships. Time is when the dancers move. The Time category includes pulse, speed, rhythmic patterns, natural rhythm, and syncopation.
As an observer of dance, it can be easy to allow our biases to influence how we perceive dance. By using dance vocabulary and stating what we observe, we can be more objective in our discussions of dance. Using the Elements of Dance, we can view dance through an unbiased lens to consider its structural elements to deepen our understanding and appreciation of dance as an art form.
Check Your Understanding
1. Try making shapes that depict literal and abstract concepts. Some examples of literal shapes might be a flower, a seashell, or a rainbow. Some abstract shapes might be circles, diamonds, or even concepts such as friendship, heroism, or depression.
2. Make a short (10 second) dance phrase and perform it twice with two different types of energy.
3. On paper, draw a map with a continuous pathway without lines overlapping. After mapping your pathway, try adding locomotor movement on various levels that compliments your pathway design.
4. Make a sentence introducing yourself and your favorite food. For example: “Cissy Whipp likes chips and guacamole.” or “Vanessa Kanamoto likes grilled shrimp.” Now try clapping the rhythm your sentence makes. (Notice how the two examples have very different rhythms.) Create a movement pattern that matches the rhythm pattern of your sentence. Practice until you can repeat it four times in a row.
Check Your Understanding
Resources:
The Elements of Dance website from Perpich Center for Arts Education in
partnership with University of MN Dance Program https://www.elementsofdance.org/
[latexpage]
Learning Objectives
By the end of this section, you will be able to:
- Identify the mathematical relationships between the various properties of gases
- Use the ideal gas law, and related gas laws, to compute the values of various gas properties under specified conditions
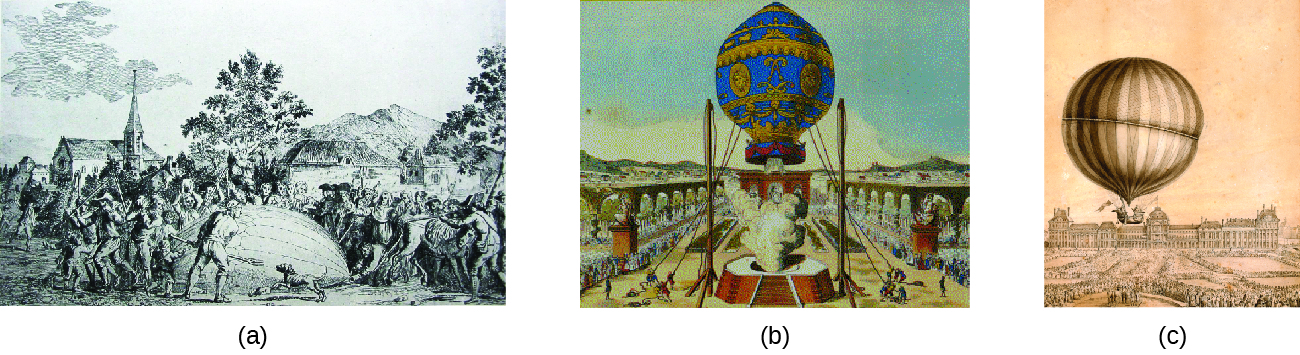
During the seventeenth and especially eighteenth centuries, driven both by a desire to understand nature and a quest to make balloons in which they could fly ((Figure)), a number of scientists established the relationships between the macroscopic physical properties of gases, that is, pressure, volume, temperature, and amount of gas. Although their measurements were not precise by today’s standards, they were able to determine the mathematical relationships between pairs of these variables (e.g., pressure and temperature, pressure and volume) that hold for an ideal gas—a hypothetical construct that real gases approximate under certain conditions. Eventually, these individual laws were combined into a single equation—the ideal gas law—that relates gas quantities for gases and is quite accurate for low pressures and moderate temperatures. We will consider the key developments in individual relationships (for pedagogical reasons not quite in historical order), then put them together in the ideal gas law.
Pressure and Temperature: Amontons’s Law
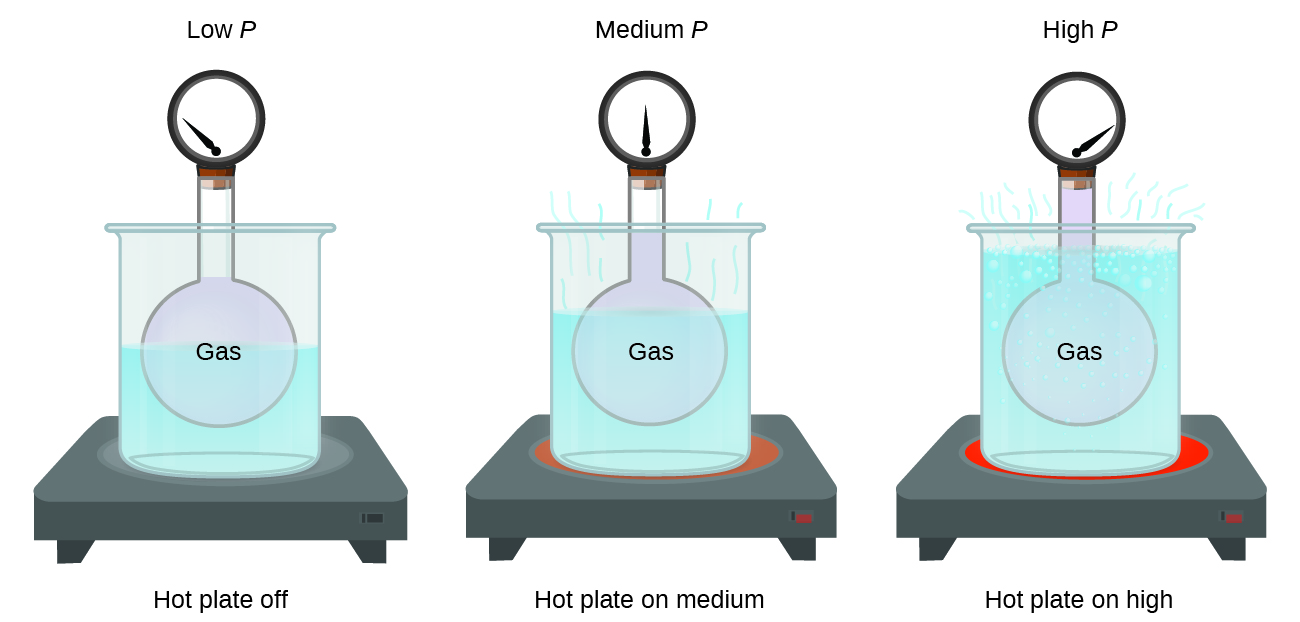
Imagine filling a rigid container attached to a pressure gauge with gas and then sealing the container so that no gas may escape. If the container is cooled, the gas inside likewise gets colder and its pressure is observed to decrease. Since the container is rigid and tightly sealed, both the volume and number of moles of gas remain constant. If we heat the sphere, the gas inside gets hotter ((Figure)) and the pressure increases.
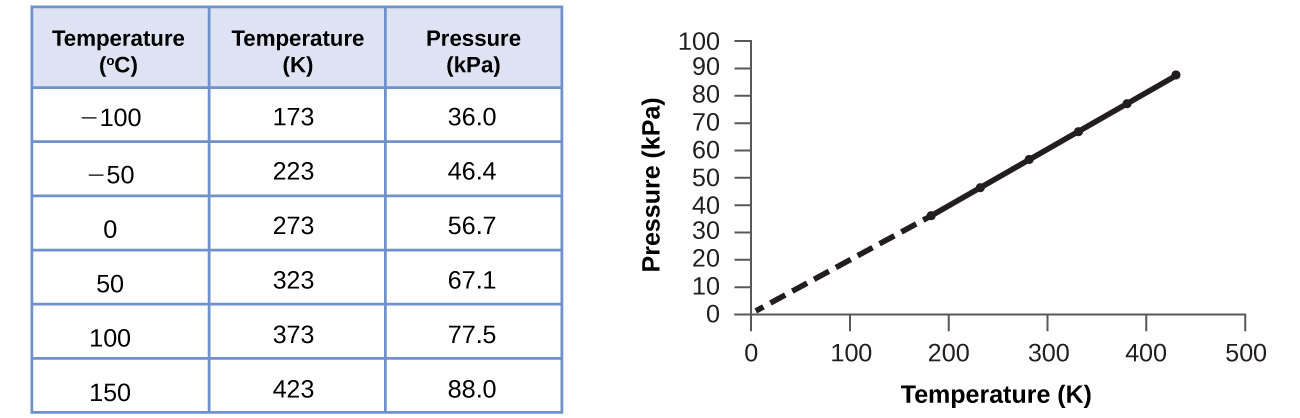
This relationship between temperature and pressure is observed for any sample of gas confined to a constant volume. An example of experimental pressure-temperature data is shown for a sample of air under these conditions in (Figure). We find that temperature and pressure are linearly related, and if the temperature is on the kelvin scale, then P and T are directly proportional (again, when volume and moles of gas are held constant); if the temperature on the kelvin scale increases by a certain factor, the gas pressure increases by the same factor.
Guillaume Amontons was the first to empirically establish the relationship between the pressure and the temperature of a gas (~1700), and Joseph Louis Gay-Lussac determined the relationship more precisely (~1800). Because of this, the P-T relationship for gases is known as either Amontons’s law or Gay-Lussac’s law. Under either name, it states that the pressure of a given amount of gas is directly proportional to its temperature on the kelvin scale when the volume is held constant. Mathematically, this can be written:
where ∝ means “is proportional to,” and k is a proportionality constant that depends on the identity, amount, and volume of the gas.
For a confined, constant volume of gas, the ratio \(\frac{P}{T}\) is therefore constant (i.e., \(\frac{P}{T}\phantom{\rule{0.2em}{0ex}}=k\)). If the gas is initially in “Condition 1” (with P = P1 and T = T1), and then changes to “Condition 2” (with P = P2 and T = T2), we have that \(\frac{{P}_{1}}{{T}_{1}}\phantom{\rule{0.2em}{0ex}}=k\) and \(\frac{{P}_{2}}{{T}_{2}}\phantom{\rule{0.2em}{0ex}}=k,\) which reduces to \(\frac{{P}_{1}}{{T}_{1}}\phantom{\rule{0.2em}{0ex}}=\phantom{\rule{0.2em}{0ex}}\frac{{P}_{2}}{{T}_{2}}.\) This equation is useful for pressure-temperature calculations for a confined gas at constant volume. Note that temperatures must be on the kelvin scale for any gas law calculations (0 on the kelvin scale and the lowest possible temperature is called absolute zero). (Also note that there are at least three ways we can describe how the pressure of a gas changes as its temperature changes: We can use a table of values, a graph, or a mathematical equation.)
Predicting Change in Pressure with Temperature A can of hair spray is used until it is empty except for the propellant, isobutane gas.
(a) On the can is the warning “Store only at temperatures below 120 °F (48.8 °C). Do not incinerate.” Why?
(b) The gas in the can is initially at 24 °C and 360 kPa, and the can has a volume of 350 mL. If the can is left in a car that reaches 50 °C on a hot day, what is the new pressure in the can?
Solution (a) The can contains an amount of isobutane gas at a constant volume, so if the temperature is increased by heating, the pressure will increase proportionately. High temperature could lead to high pressure, causing the can to burst. (Also, isobutane is combustible, so incineration could cause the can to explode.)
(b) We are looking for a pressure change due to a temperature change at constant volume, so we will use Amontons’s/Gay-Lussac’s law. Taking P1 and T1 as the initial values, T2 as the temperature where the pressure is unknown and P2 as the unknown pressure, and converting °C to K, we have:
Rearranging and solving gives: \({P}_{2}=\phantom{\rule{0.2em}{0ex}}\frac{360\phantom{\rule{0.2em}{0ex}}\text{kPa}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}323\phantom{\rule{0.2em}{0ex}}\overline{)\text{K}}}{297\phantom{\rule{0.2em}{0ex}}\overline{)\text{K}}}\phantom{\rule{0.2em}{0ex}}=390\phantom{\rule{0.2em}{0ex}}\text{kPa}\)
Check Your Learning A sample of nitrogen, N2, occupies 45.0 mL at 27 °C and 600 torr. What pressure will it have if cooled to –73 °C while the volume remains constant?
400 torr
Volume and Temperature: Charles’s Law
If we fill a balloon with air and seal it, the balloon contains a specific amount of air at atmospheric pressure, let’s say 1 atm. If we put the balloon in a refrigerator, the gas inside gets cold and the balloon shrinks (although both the amount of gas and its pressure remain constant). If we make the balloon very cold, it will shrink a great deal, and it expands again when it warms up.
This video shows how cooling and heating a gas causes its volume to decrease or increase, respectively.
These examples of the effect of temperature on the volume of a given amount of a confined gas at constant pressure are true in general: The volume increases as the temperature increases, and decreases as the temperature decreases. Volume-temperature data for a 1-mole sample of methane gas at 1 atm are listed and graphed in (Figure).
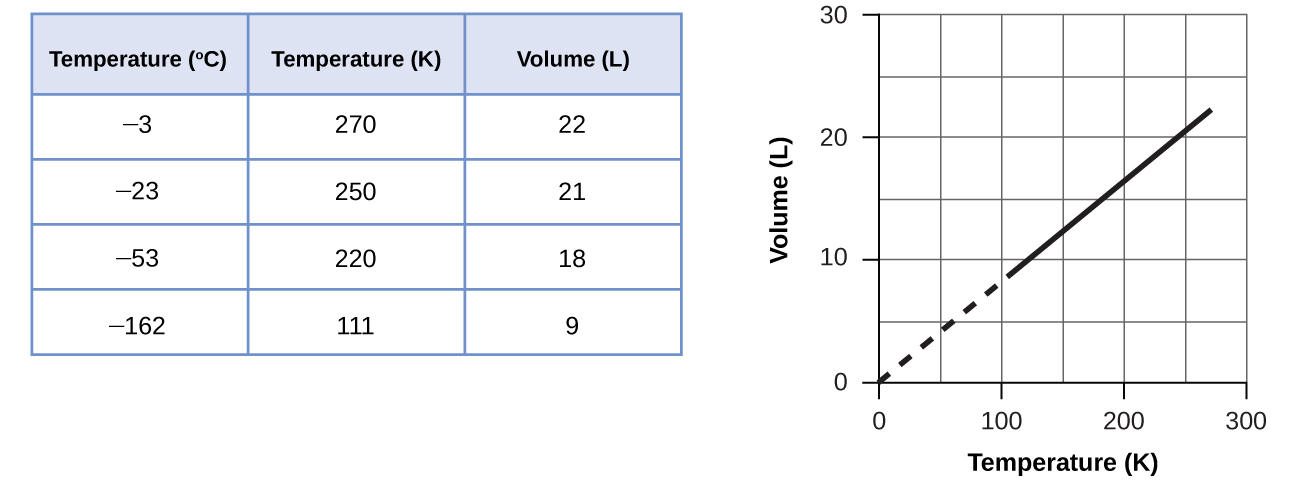
The relationship between the volume and temperature of a given amount of gas at constant pressure is known as Charles’s law in recognition of the French scientist and balloon flight pioneer Jacques Alexandre César Charles. Charles’s law states that the volume of a given amount of gas is directly proportional to its temperature on the kelvin scale when the pressure is held constant.
Mathematically, this can be written as:
with k being a proportionality constant that depends on the amount and pressure of the gas.
For a confined, constant pressure gas sample, \(\frac{V}{T}\) is constant (i.e., the ratio = k), and as seen with the P-T relationship, this leads to another form of Charles’s law: \(\frac{{V}_{1}}{{T}_{1}}\phantom{\rule{0.2em}{0ex}}=\phantom{\rule{0.2em}{0ex}}\frac{{V}_{2}}{{T}_{2}}.\)
Predicting Change in Volume with Temperature A sample of carbon dioxide, CO2, occupies 0.300 L at 10 °C and 750 torr. What volume will the gas have at 30 °C and 750 torr?
Solution Because we are looking for the volume change caused by a temperature change at constant pressure, this is a job for Charles’s law. Taking V1 and T1 as the initial values, T2 as the temperature at which the volume is unknown and V2 as the unknown volume, and converting °C into K we have:
Rearranging and solving gives: \({V}_{2}=\phantom{\rule{0.2em}{0ex}}\frac{0.300\phantom{\rule{0.2em}{0ex}}\text{L}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\text{303}\phantom{\rule{0.2em}{0ex}}\overline{)\text{K}}}{283\phantom{\rule{0.2em}{0ex}}\overline{)\text{K}}}\phantom{\rule{0.2em}{0ex}}=0.321\phantom{\rule{0.2em}{0ex}}\text{L}\)
This answer supports our expectation from Charles’s law, namely, that raising the gas temperature (from 283 K to 303 K) at a constant pressure will yield an increase in its volume (from 0.300 L to 0.321 L).
Check Your Learning A sample of oxygen, O2, occupies 32.2 mL at 30 °C and 452 torr. What volume will it occupy at –70 °C and the same pressure?
21.6 mL
Measuring Temperature with a Volume Change Temperature is sometimes measured with a gas thermometer by observing the change in the volume of the gas as the temperature changes at constant pressure. The hydrogen in a particular hydrogen gas thermometer has a volume of 150.0 cm3 when immersed in a mixture of ice and water (0.00 °C). When immersed in boiling liquid ammonia, the volume of the hydrogen, at the same pressure, is 131.7 cm3. Find the temperature of boiling ammonia on the kelvin and Celsius scales.
Solution A volume change caused by a temperature change at constant pressure means we should use Charles’s law. Taking V1 and T1 as the initial values, T2 as the temperature at which the volume is unknown and V2 as the unknown volume, and converting °C into K we have:
Rearrangement gives \({T}_{2}=\phantom{\rule{0.2em}{0ex}}\frac{131.7\phantom{\rule{0.2em}{0ex}}{\overline{)\text{cm}}}^{3}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}273.15\phantom{\rule{0.2em}{0ex}}\text{K}}{150.0\phantom{\rule{0.2em}{0ex}}{\overline{)\text{cm}}}^{3}}\phantom{\rule{0.2em}{0ex}}=239.8\phantom{\rule{0.2em}{0ex}}\text{K}\)
Subtracting 273.15 from 239.8 K, we find that the temperature of the boiling ammonia on the Celsius scale is –33.4 °C.
Check Your Learning What is the volume of a sample of ethane at 467 K and 1.1 atm if it occupies 405 mL at 298 K and 1.1 atm?
635 mL
Volume and Pressure: Boyle’s Law
If we partially fill an airtight syringe with air, the syringe contains a specific amount of air at constant temperature, say 25 °C. If we slowly push in the plunger while keeping temperature constant, the gas in the syringe is compressed into a smaller volume and its pressure increases; if we pull out the plunger, the volume increases and the pressure decreases. This example of the effect of volume on the pressure of a given amount of a confined gas is true in general. Decreasing the volume of a contained gas will increase its pressure, and increasing its volume will decrease its pressure. In fact, if the volume increases by a certain factor, the pressure decreases by the same factor, and vice versa. Volume-pressure data for an air sample at room temperature are graphed in (Figure).
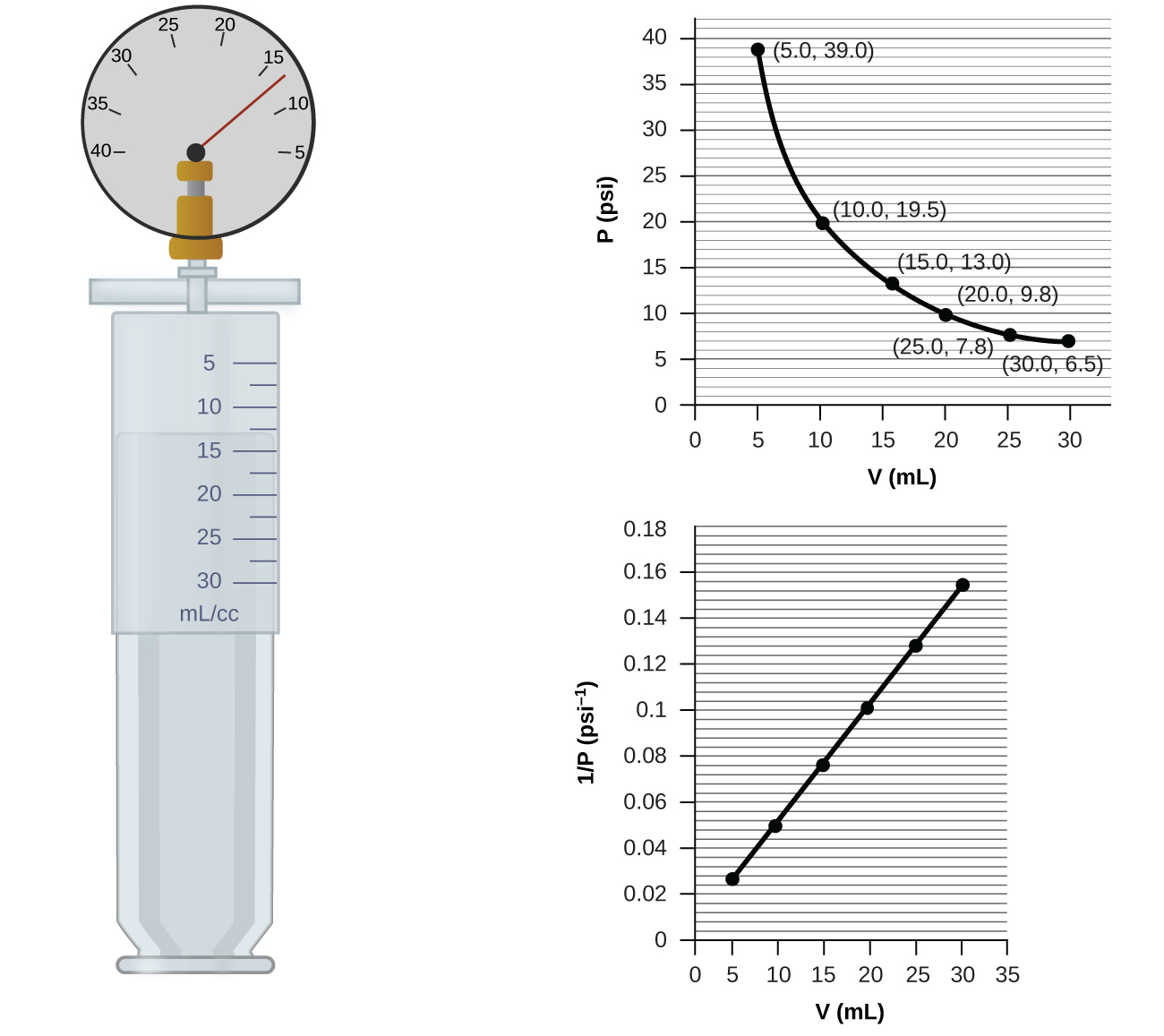
Unlike the P-T and V-T relationships, pressure and volume are not directly proportional to each other. Instead, P and V exhibit inverse proportionality: Increasing the pressure results in a decrease of the volume of the gas. Mathematically this can be written:
with k being a constant. Graphically, this relationship is shown by the straight line that results when plotting the inverse of the pressure \(\left(\frac{1}{P}\right)\) versus the volume (V), or the inverse of volume \(\left(\frac{1}{V}\right)\) versus the pressure (P). Graphs with curved lines are difficult to read accurately at low or high values of the variables, and they are more difficult to use in fitting theoretical equations and parameters to experimental data. For those reasons, scientists often try to find a way to “linearize” their data. If we plot P versus V, we obtain a hyperbola (see (Figure)).

The relationship between the volume and pressure of a given amount of gas at constant temperature was first published by the English natural philosopher Robert Boyle over 300 years ago. It is summarized in the statement now known as Boyle’s law: The volume of a given amount of gas held at constant temperature is inversely proportional to the pressure under which it is measured.
Volume of a Gas Sample The sample of gas in (Figure) has a volume of 15.0 mL at a pressure of 13.0 psi. Determine the pressure of the gas at a volume of 7.5 mL, using:
(a) the P-V graph in (Figure)
(b) the \(\frac{1}{P}\) vs. V graph in (Figure)
(c) the Boyle’s law equation
Comment on the likely accuracy of each method.
Solution (a) Estimating from the P-V graph gives a value for P somewhere around 27 psi.
(b) Estimating from the \(\frac{1}{P}\) versus V graph give a value of about 26 psi.
(c) From Boyle’s law, we know that the product of pressure and volume (PV) for a given sample of gas at a constant temperature is always equal to the same value. Therefore we have P1V1 = k and P2V2 = k which means that P1V1 = P2V2.
Using P1 and V1 as the known values 13.0 psi and 15.0 mL, P2 as the pressure at which the volume is unknown, and V2 as the unknown volume, we have:
Solving:
It was more difficult to estimate well from the P-V graph, so (a) is likely more inaccurate than (b) or (c). The calculation will be as accurate as the equation and measurements allow.
Check Your LearningThe sample of gas in (Figure) has a volume of 30.0 mL at a pressure of 6.5 psi. Determine the volume of the gas at a pressure of 11.0 psi, using:
(a) the P-V graph in (Figure)
(b) the \(\frac{1}{P}\) vs. V graph in (Figure)
(c) the Boyle’s law equation
Comment on the likely accuracy of each method.
(a) about 17–18 mL; (b) ~18 mL; (c) 17.7 mL; it was more difficult to estimate well from the P-V graph, so (a) is likely more inaccurate than (b); the calculation will be as accurate as the equation and measurements allow
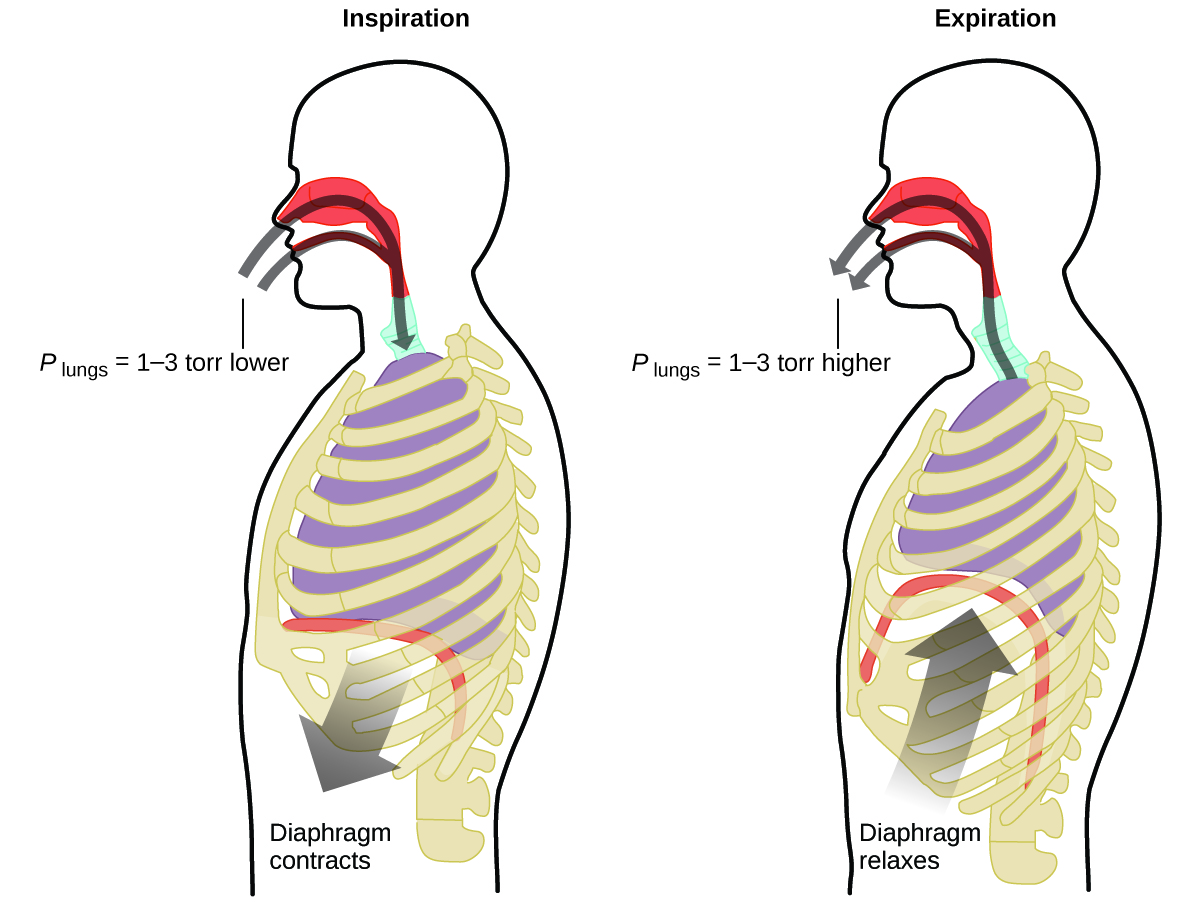
What do you do about 20 times per minute for your whole life, without break, and often without even being aware of it? The answer, of course, is respiration, or breathing. How does it work? It turns out that the gas laws apply here. Your lungs take in gas that your body needs (oxygen) and get rid of waste gas (carbon dioxide). Lungs are made of spongy, stretchy tissue that expands and contracts while you breathe. When you inhale, your diaphragm and intercostal muscles (the muscles between your ribs) contract, expanding your chest cavity and making your lung volume larger. The increase in volume leads to a decrease in pressure (Boyle’s law). This causes air to flow into the lungs (from high pressure to low pressure). When you exhale, the process reverses: Your diaphragm and rib muscles relax, your chest cavity contracts, and your lung volume decreases, causing the pressure to increase (Boyle’s law again), and air flows out of the lungs (from high pressure to low pressure). You then breathe in and out again, and again, repeating this Boyle’s law cycle for the rest of your life ((Figure)).
Moles of Gas and Volume: Avogadro’s Law
The Italian scientist Amedeo Avogadro advanced a hypothesis in 1811 to account for the behavior of gases, stating that equal volumes of all gases, measured under the same conditions of temperature and pressure, contain the same number of molecules. Over time, this relationship was supported by many experimental observations as expressed by Avogadro’s law: For a confined gas, the volume (V) and number of moles (n) are directly proportional if the pressure and temperature both remain constant.
In equation form, this is written as:
Mathematical relationships can also be determined for the other variable pairs, such as P versus n, and n versus T.
Visit this interactive PhET simulation to investigate the relationships between pressure, volume, temperature, and amount of gas. Use the simulation to examine the effect of changing one parameter on another while holding the other parameters constant (as described in the preceding sections on the various gas laws).
The Ideal Gas Law
To this point, four separate laws have been discussed that relate pressure, volume, temperature, and the number of moles of the gas:
- Boyle’s law: PV = constant at constant T and n
- Amontons’s law: \(\frac{P}{T}\) = constant at constant V and n
- Charles’s law: \(\frac{V}{T}\) = constant at constant P and n
- Avogadro’s law: \(\frac{V}{n}\) = constant at constant P and T
Combining these four laws yields the ideal gas law, a relation between the pressure, volume, temperature, and number of moles of a gas:
where P is the pressure of a gas, V is its volume, n is the number of moles of the gas, T is its temperature on the kelvin scale, and R is a constant called the ideal gas constant or the universal gas constant. The units used to express pressure, volume, and temperature will determine the proper form of the gas constant as required by dimensional analysis, the most commonly encountered values being 0.08206 L atm mol–1 K–1 and 8.314 kPa L mol–1 K–1.
Gases whose properties of P, V, and T are accurately described by the ideal gas law (or the other gas laws) are said to exhibit ideal behavior or to approximate the traits of an ideal gas. An ideal gas is a hypothetical construct that may be used along with kinetic molecular theory to effectively explain the gas laws as will be described in a later module of this chapter. Although all the calculations presented in this module assume ideal behavior, this assumption is only reasonable for gases under conditions of relatively low pressure and high temperature. In the final module of this chapter, a modified gas law will be introduced that accounts for the non-ideal behavior observed for many gases at relatively high pressures and low temperatures.
The ideal gas equation contains five terms, the gas constant R and the variable properties P, V, n, and T. Specifying any four of these terms will permit use of the ideal gas law to calculate the fifth term as demonstrated in the following example exercises.
Using the Ideal Gas Law Methane, CH4, is being considered for use as an alternative automotive fuel to replace gasoline. One gallon of gasoline could be replaced by 655 g of CH4. What is the volume of this much methane at 25 °C and 745 torr?
Solution We must rearrange PV = nRT to solve for V: \(V=\phantom{\rule{0.2em}{0ex}}\frac{nRT}{P}\)
If we choose to use R = 0.08206 L atm mol–1 K–1, then the amount must be in moles, temperature must be in kelvin, and pressure must be in atm.
Converting into the “right” units:
It would require 1020 L (269 gal) of gaseous methane at about 1 atm of pressure to replace 1 gal of gasoline. It requires a large container to hold enough methane at 1 atm to replace several gallons of gasoline.
Check Your Learning Calculate the pressure in bar of 2520 moles of hydrogen gas stored at 27 °C in the 180-L storage tank of a modern hydrogen-powered car.
350 bar
If the number of moles of an ideal gas are kept constant under two different sets of conditions, a useful mathematical relationship called the combined gas law is obtained: \(\frac{{P}_{1}{V}_{1}}{{T}_{1}}\phantom{\rule{0.2em}{0ex}}=\phantom{\rule{0.2em}{0ex}}\frac{{P}_{2}{V}_{2}}{{T}_{2}}\) using units of atm, L, and K. Both sets of conditions are equal to the product of n \(×\) R (where n = the number of moles of the gas and R is the ideal gas law constant).
Using the Combined Gas Law When filled with air, a typical scuba tank with a volume of 13.2 L has a pressure of 153 atm ((Figure)). If the water temperature is 27 °C, how many liters of air will such a tank provide to a diver’s lungs at a depth of approximately 70 feet in the ocean where the pressure is 3.13 atm?

Letting 1 represent the air in the scuba tank and 2 represent the air in the lungs, and noting that body temperature (the temperature the air will be in the lungs) is 37 °C, we have:
Solving for V2:
(Note: Be advised that this particular example is one in which the assumption of ideal gas behavior is not very reasonable, since it involves gases at relatively high pressures and low temperatures. Despite this limitation, the calculated volume can be viewed as a good “ballpark” estimate.)
Check Your Learning A sample of ammonia is found to occupy 0.250 L under laboratory conditions of 27 °C and 0.850 atm. Find the volume of this sample at 0 °C and 1.00 atm.
0.193 L
Whether scuba diving at the Great Barrier Reef in Australia (shown in (Figure)) or in the Caribbean, divers must understand how pressure affects a number of issues related to their comfort and safety.

Pressure increases with ocean depth, and the pressure changes most rapidly as divers reach the surface. The pressure a diver experiences is the sum of all pressures above the diver (from the water and the air). Most pressure measurements are given in units of atmospheres, expressed as “atmospheres absolute” or ATA in the diving community: Every 33 feet of salt water represents 1 ATA of pressure in addition to 1 ATA of pressure from the atmosphere at sea level. As a diver descends, the increase in pressure causes the body’s air pockets in the ears and lungs to compress; on the ascent, the decrease in pressure causes these air pockets to expand, potentially rupturing eardrums or bursting the lungs. Divers must therefore undergo equalization by adding air to body airspaces on the descent by breathing normally and adding air to the mask by breathing out of the nose or adding air to the ears and sinuses by equalization techniques; the corollary is also true on ascent, divers must release air from the body to maintain equalization. Buoyancy, or the ability to control whether a diver sinks or floats, is controlled by the buoyancy compensator (BCD). If a diver is ascending, the air in his BCD expands because of lower pressure according to Boyle’s law (decreasing the pressure of gases increases the volume). The expanding air increases the buoyancy of the diver, and she or he begins to ascend. The diver must vent air from the BCD or risk an uncontrolled ascent that could rupture the lungs. In descending, the increased pressure causes the air in the BCD to compress and the diver sinks much more quickly; the diver must add air to the BCD or risk an uncontrolled descent, facing much higher pressures near the ocean floor. The pressure also impacts how long a diver can stay underwater before ascending. The deeper a diver dives, the more compressed the air that is breathed because of increased pressure: If a diver dives 33 feet, the pressure is 2 ATA and the air would be compressed to one-half of its original volume. The diver uses up available air twice as fast as at the surface.
Standard Conditions of Temperature and Pressure
We have seen that the volume of a given quantity of gas and the number of molecules (moles) in a given volume of gas vary with changes in pressure and temperature. Chemists sometimes make comparisons against a standard temperature and pressure (STP) for reporting properties of gases: 273.15 K and 1 atm (101.325 kPa).1 At STP, one mole of an ideal gas has a volume of about 22.4 L—this is referred to as the standard molar volume ((Figure)).
Key Concepts and Summary
The behavior of gases can be described by several laws based on experimental observations of their properties. The pressure of a given amount of gas is directly proportional to its absolute temperature, provided that the volume does not change (Amontons’s law). The volume of a given gas sample is directly proportional to its absolute temperature at constant pressure (Charles’s law). The volume of a given amount of gas is inversely proportional to its pressure when temperature is held constant (Boyle’s law). Under the same conditions of temperature and pressure, equal volumes of all gases contain the same number of molecules (Avogadro’s law).
The equations describing these laws are special cases of the ideal gas law, PV = nRT, where P is the pressure of the gas, V is its volume, n is the number of moles of the gas, T is its kelvin temperature, and R is the ideal (universal) gas constant.
Key Equations
- PV = nRT
Chemistry End of Chapter Exercises
Sometimes leaving a bicycle in the sun on a hot day will cause a blowout. Why?
Explain how the volume of the bubbles exhausted by a scuba diver ((Figure)) change as they rise to the surface, assuming that they remain intact.
As the bubbles rise, the pressure decreases, so their volume increases as suggested by Boyle’s law.
One way to state Boyle’s law is “All other things being equal, the pressure of a gas is inversely proportional to its volume.” (a) What is the meaning of the term “inversely proportional?” (b) What are the “other things” that must be equal?
An alternate way to state Avogadro’s law is “All other things being equal, the number of molecules in a gas is directly proportional to the volume of the gas.” (a) What is the meaning of the term “directly proportional?” (b) What are the “other things” that must be equal?
(a) The number of particles in the gas increases as the volume increases. (b) temperature, pressure
How would the graph in (Figure) change if the number of moles of gas in the sample used to determine the curve were doubled?
How would the graph in (Figure) change if the number of moles of gas in the sample used to determine the curve were doubled?
The curve would be farther to the right and higher up, but the same basic shape.
In addition to the data found in (Figure), what other information do we need to find the mass of the sample of air used to determine the graph?
Determine the volume of 1 mol of CH4 gas at 150 K and 1 atm, using (Figure).
About 12.5 L
Determine the pressure of the gas in the syringe shown in (Figure) when its volume is 12.5 mL, using:
(a) the appropriate graph
(b) Boyle’s law
A spray can is used until it is empty except for the propellant gas, which has a pressure of 1344 torr at 23 °C. If the can is thrown into a fire (T = 475 °C), what will be the pressure in the hot can?
3.40 \(×\) 103 torr
What is the temperature of an 11.2-L sample of carbon monoxide, CO, at 744 torr if it occupies 13.3 L at 55 °C and 744 torr?
A 2.50-L volume of hydrogen measured at –196 °C is warmed to 100 °C. Calculate the volume of the gas at the higher temperature, assuming no change in pressure.
12.1 L
A balloon inflated with three breaths of air has a volume of 1.7 L. At the same temperature and pressure, what is the volume of the balloon if five more same-sized breaths are added to the balloon?
A weather balloon contains 8.80 moles of helium at a pressure of 0.992 atm and a temperature of 25 °C at ground level. What is the volume of the balloon under these conditions?

217 L
The volume of an automobile air bag was 66.8 L when inflated at 25 °C with 77.8 g of nitrogen gas. What was the pressure in the bag in kPa?
How many moles of gaseous boron trifluoride, BF3, are contained in a 4.3410-L bulb at 788.0 K if the pressure is 1.220 atm? How many grams of BF3?
8.190 \(×\) 10–2 mol; 5.553 g
Iodine, I2, is a solid at room temperature but sublimes (converts from a solid into a gas) when warmed. What is the temperature in a 73.3-mL bulb that contains 0.292 g of I2 vapor at a pressure of 0.462 atm?
How many grams of gas are present in each of the following cases?
(a) 0.100 L of CO2 at 307 torr and 26 °C
(b) 8.75 L of C2H4, at 378.3 kPa and 483 K
(c) 221 mL of Ar at 0.23 torr and –54 °C
(a) 7.24 \(×\) 10–2 g; (b) 23.1 g; (c) 1.5 \(×\) 10–4 g
A high altitude balloon is filled with 1.41 \(×\) 104 L of hydrogen at a temperature of 21 °C and a pressure of 745 torr. What is the volume of the balloon at a height of 20 km, where the temperature is –48 °C and the pressure is 63.1 torr?
A cylinder of medical oxygen has a volume of 35.4 L, and contains O2 at a pressure of 151 atm and a temperature of 25 °C. What volume of O2 does this correspond to at normal body conditions, that is, 1 atm and 37 °C?
5561 L
A large scuba tank ((Figure)) with a volume of 18 L is rated for a pressure of 220 bar. The tank is filled at 20 °C and contains enough air to supply 1860 L of air to a diver at a pressure of 2.37 atm (a depth of 45 feet). Was the tank filled to capacity at 20 °C?
A 20.0-L cylinder containing 11.34 kg of butane, C4H10, was opened to the atmosphere. Calculate the mass of the gas remaining in the cylinder if it were opened and the gas escaped until the pressure in the cylinder was equal to the atmospheric pressure, 0.983 atm, and a temperature of 27 °C.
46.4 g
While resting, the average 70-kg human male consumes 14 L of pure O2 per hour at 25 °C and 100 kPa. How many moles of O2 are consumed by a 70 kg man while resting for 1.0 h?
For a given amount of gas showing ideal behavior, draw labeled graphs of:
(a) the variation of P with V
(b) the variation of V with T
(c) the variation of P with T
(d) the variation of \(\frac{1}{P}\) with V
For a gas exhibiting ideal behavior:

A liter of methane gas, CH4, at STP contains more atoms of hydrogen than does a liter of pure hydrogen gas, H2, at STP. Using Avogadro’s law as a starting point, explain why.
The effect of chlorofluorocarbons (such as CCl2F2) on the depletion of the ozone layer is well known. The use of substitutes, such as CH3CH2F(g), for the chlorofluorocarbons, has largely corrected the problem. Calculate the volume occupied by 10.0 g of each of these compounds at STP:
(a) CCl2F2(g)
(b) CH3CH2F(g)
(a) 1.85 L CCl2F2; (b) 4.66 L CH3CH2F
As 1 g of the radioactive element radium decays over 1 year, it produces 1.16 \(×\) 1018 alpha particles (helium nuclei). Each alpha particle becomes an atom of helium gas. What is the pressure in pascal of the helium gas produced if it occupies a volume of 125 mL at a temperature of 25 °C?
A balloon with a volume of 100.21 L at 21 °C and 0.981 atm is released and just barely clears the top of Mount Crumpet in British Columbia. If the final volume of the balloon is 144.53 L at a temperature of 5.24 °C, what is the pressure experienced by the balloon as it clears Mount Crumpet?
0.644 atm
If the temperature of a fixed amount of a gas is doubled at constant volume, what happens to the pressure?
If the volume of a fixed amount of a gas is tripled at constant temperature, what happens to the pressure?
The pressure decreases by a factor of 3.
Footnotes
- 1The IUPAC definition of standard pressure was changed from 1 atm to 1 bar (100 kPa) in 1982, but the prior definition remains in use by many literature resources and will be used in this text.
Glossary
- absolute zero
- temperature at which the volume of a gas would be zero according to Charles’s law.
- Amontons’s law
- (also, Gay-Lussac’s law) pressure of a given number of moles of gas is directly proportional to its kelvin temperature when the volume is held constant
- Avogadro’s law
- volume of a gas at constant temperature and pressure is proportional to the number of gas molecules
- Boyle’s law
- volume of a given number of moles of gas held at constant temperature is inversely proportional to the pressure under which it is measured
- Charles’s law
- volume of a given number of moles of gas is directly proportional to its kelvin temperature when the pressure is held constant
- ideal gas
- hypothetical gas whose physical properties are perfectly described by the gas laws
- ideal gas constant (R)
- constant derived from the ideal gas equation R = 0.08206 L atm mol–1 K–1 or 8.314 L kPa mol–1 K–1
- ideal gas law
- relation between the pressure, volume, amount, and temperature of a gas under conditions derived by combination of the simple gas laws
- standard conditions of temperature and pressure (STP)
- 273.15 K (0 °C) and 1 atm (101.325 kPa)
- standard molar volume
- volume of 1 mole of gas at STP, approximately 22.4 L for gases behaving ideally
[latexpage]
Learning Objectives
By the end of this section, you will be able to:
- Use the ideal gas law to compute gas densities and molar masses
- Perform stoichiometric calculations involving gaseous substances
- State Dalton’s law of partial pressures and use it in calculations involving gaseous mixtures
The study of the chemical behavior of gases was part of the basis of perhaps the most fundamental chemical revolution in history. French nobleman Antoine Lavoisier, widely regarded as the “father of modern chemistry,” changed chemistry from a qualitative to a quantitative science through his work with gases. He discovered the law of conservation of matter, discovered the role of oxygen in combustion reactions, determined the composition of air, explained respiration in terms of chemical reactions, and more. He was a casualty of the French Revolution, guillotined in 1794. Of his death, mathematician and astronomer Joseph-Louis Lagrange said, “It took the mob only a moment to remove his head; a century will not suffice to reproduce it.”1
As described in an earlier chapter of this text, we can turn to chemical stoichiometry for answers to many of the questions that ask “How much?” The essential property involved in such use of stoichiometry is the amount of substance, typically measured in moles (n). For gases, molar amount can be derived from convenient experimental measurements of pressure, temperature, and volume. Therefore, these measurements are useful in assessing the stoichiometry of pure gases, gas mixtures, and chemical reactions involving gases. This section will not introduce any new material or ideas, but will provide examples of applications and ways to integrate concepts we have already discussed.
Gas Density and Molar Mass
The ideal gas law described previously in this chapter relates the properties of pressure P, volume V, temperature T, and molar amount n. This law is universal, relating these properties in identical fashion regardless of the chemical identity of the gas:
The density d of a gas, on the other hand, is determined by its identity. As described in another chapter of this text, the density of a substance is a characteristic property that may be used to identify the substance.
Rearranging the ideal gas equation to isolate V and substituting into the density equation yields
The ratio m/n is the definition of molar mass, ℳ:
The density equation can then be written
This relation may be used for calculating the densities of gases of known identities at specified values of pressure and temperature as demonstrated in (Figure).
Measuring Gas Density What is the density of molecular nitrogen gas at STP?
Solution The molar mass of molecular nitrogen, N2, is 28.01 g/mol. Substituting this value along with standard temperature and pressure into the gas density equation yields
Check Your Learning What is the density of molecular hydrogen gas at 17.0 °C and a pressure of 760 torr?
d = 0.0847 g/L
When the identity of a gas is unknown, measurements of the mass, pressure, volume, and temperature of a sample can be used to calculate the molar mass of the gas (a useful property for identification purposes). Combining the ideal gas equation
and the definition of molarity
yields the following equation:
Determining the molar mass of a gas via this approach is demonstrated in (Figure).
Determining the Molecular Formula of a Gas from its Molar Mass and Empirical Formula Cyclopropane, a gas once used with oxygen as a general anesthetic, is composed of 85.7% carbon and 14.3% hydrogen by mass. Find the empirical formula. If 1.56 g of cyclopropane occupies a volume of 1.00 L at 0.984 atm and 50 °C, what is the molecular formula for cyclopropane?
Solution First determine the empirical formula of the gas. Assume 100 g and convert the percentage of each element into grams. Determine the number of moles of carbon and hydrogen in the 100-g sample of cyclopropane. Divide by the smallest number of moles to relate the number of moles of carbon to the number of moles of hydrogen. In the last step, realize that the smallest whole number ratio is the empirical formula:
Empirical formula is CH2 [empirical mass (EM) of 14.03 g/empirical unit].
Next, use the provided values for mass, pressure, temperature and volume to compute the molar mass of the gas:
Comparing the molar mass to the empirical formula mass shows how many empirical formula units make up a molecule:
The molecular formula is thus derived from the empirical formula by multiplying each of its subscripts by three:
Check Your LearningAcetylene, a fuel used welding torches, is composed of 92.3% C and 7.7% H by mass. Find the empirical formula. If 1.10 g of acetylene occupies of volume of 1.00 L at 1.15 atm and 59.5 °C, what is the molecular formula for acetylene?
Empirical formula, CH; Molecular formula, C2H2
Determining the Molar Mass of a Volatile Liquid The approximate molar mass of a volatile liquid can be determined by:
- Heating a sample of the liquid in a flask with a tiny hole at the top, which converts the liquid into gas that may escape through the hole
- Removing the flask from heat at the instant when the last bit of liquid becomes gas, at which time the flask will be filled with only gaseous sample at ambient pressure
- Sealing the flask and permitting the gaseous sample to condense to liquid, and then weighing the flask to determine the sample’s mass (see (Figure))
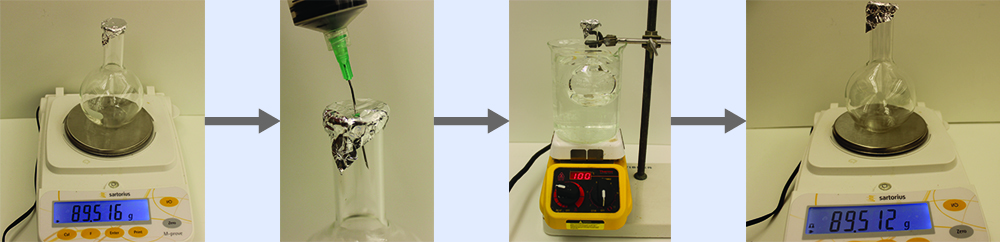
Using this procedure, a sample of chloroform gas weighing 0.494 g is collected in a flask with a volume of 129 cm3 at 99.6 °C when the atmospheric pressure is 742.1 mm Hg. What is the approximate molar mass of chloroform?
Solution Since \(\text{ℳ}=\phantom{\rule{0.2em}{0ex}}\frac{m}{n}\) and \(n=\phantom{\rule{0.2em}{0ex}}\frac{PV}{RT},\) substituting and rearranging gives \(\text{ℳ}=\phantom{\rule{0.2em}{0ex}}\frac{mRT}{PV},\)
then
Check Your Learning A sample of phosphorus that weighs 3.243 \(×\) 10−2 g exerts a pressure of 31.89 kPa in a 56.0-mL bulb at 550 °C. What are the molar mass and molecular formula of phosphorus vapor?
124 g/mol P4
The Pressure of a Mixture of Gases: Dalton’s Law
Unless they chemically react with each other, the individual gases in a mixture of gases do not affect each other’s pressure. Each individual gas in a mixture exerts the same pressure that it would exert if it were present alone in the container ((Figure)). The pressure exerted by each individual gas in a mixture is called its partial pressure. This observation is summarized by Dalton’s law of partial pressures: The total pressure of a mixture of ideal gases is equal to the sum of the partial pressures of the component gases:
In the equation PTotal is the total pressure of a mixture of gases, PA is the partial pressure of gas A; PB is the partial pressure of gas B; PC is the partial pressure of gas C; and so on.
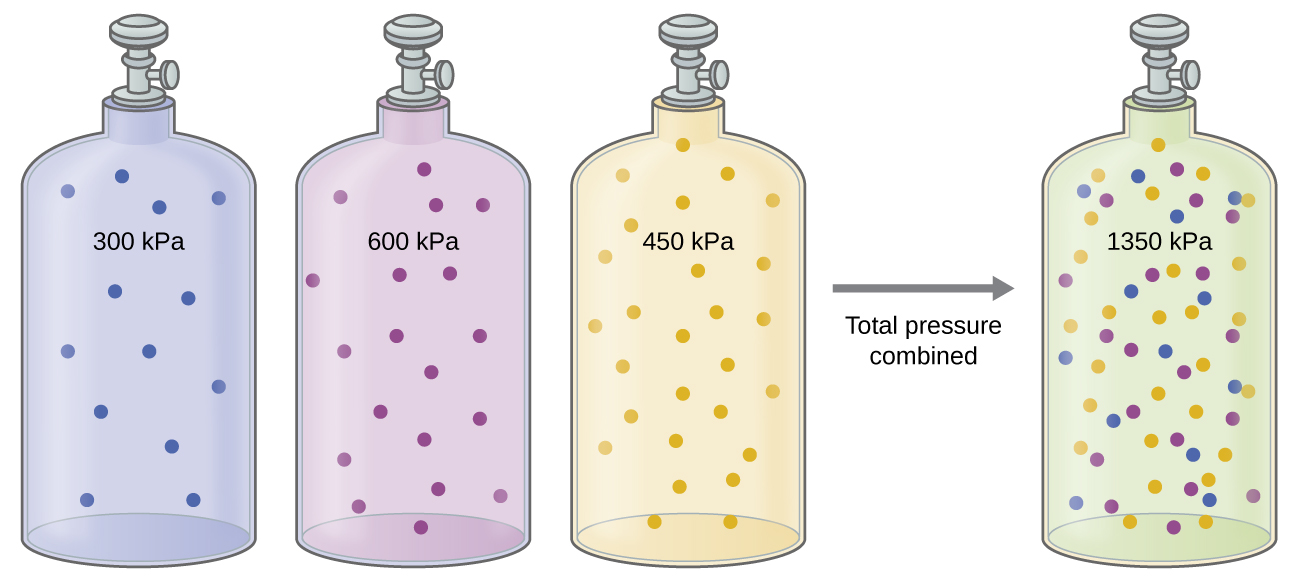
The partial pressure of gas A is related to the total pressure of the gas mixture via its mole fraction (X), a unit of concentration defined as the number of moles of a component of a solution divided by the total number of moles of all components:
where PA, XA, and nA are the partial pressure, mole fraction, and number of moles of gas A, respectively, and nTotal is the number of moles of all components in the mixture.
The Pressure of a Mixture of Gases A 10.0-L vessel contains 2.50 \(×\) 10−3 mol of H2, 1.00 \(×\) 10−3 mol of He, and 3.00 \(×\) 10−4 mol of Ne at 35 °C.
(a) What are the partial pressures of each of the gases?
(b) What is the total pressure in atmospheres?
SolutionThe gases behave independently, so the partial pressure of each gas can be determined from the ideal gas equation, using \(P=\phantom{\rule{0.2em}{0ex}}\frac{nRT}{V}\):
The total pressure is given by the sum of the partial pressures:
Check Your Learning A 5.73-L flask at 25 °C contains 0.0388 mol of N2, 0.147 mol of CO, and 0.0803 mol of H2. What is the total pressure in the flask in atmospheres?
1.137 atm
Here is another example of this concept, but dealing with mole fraction calculations.
The Pressure of a Mixture of Gases A gas mixture used for anesthesia contains 2.83 mol oxygen, O2, and 8.41 mol nitrous oxide, N2O. The total pressure of the mixture is 192 kPa.
(a) What are the mole fractions of O2 and N2O?
(b) What are the partial pressures of O2 and N2O?
Solution The mole fraction is given by \({X}_{A}=\phantom{\rule{0.2em}{0ex}}\frac{{n}_{A}}{{n}_{Total}}\) and the partial pressure is PA = XA \(×\) PTotal.
For O2,
and \({P}_{{O}_{2}}={X}_{{O}_{2}}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{P}_{Total}=0.252\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\text{192 kPa}=\text{48.4 kPa}\)
For N2O,
and
\({P}_{{N}_{2}}={X}_{{N}_{2}}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{P}_{\mathrm{Total}}=0.748\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\text{192 kPa}=\text{143.6 kPa}\)
Check Your Learning What is the pressure of a mixture of 0.200 g of H2, 1.00 g of N2, and 0.820 g of Ar in a container with a volume of 2.00 L at 20 °C?
1.87 atm
Collection of Gases over Water
A simple way to collect gases that do not react with water is to capture them in a bottle that has been filled with water and inverted into a dish filled with water. The pressure of the gas inside the bottle can be made equal to the air pressure outside by raising or lowering the bottle. When the water level is the same both inside and outside the bottle ((Figure)), the pressure of the gas is equal to the atmospheric pressure, which can be measured with a barometer.
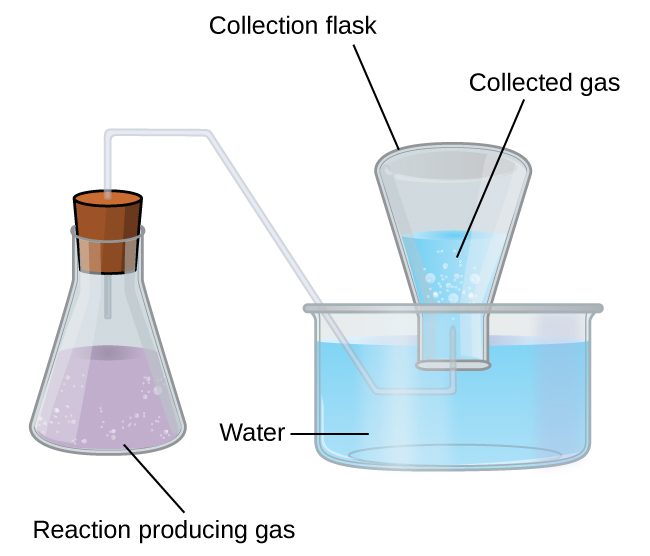
However, there is another factor we must consider when we measure the pressure of the gas by this method. Water evaporates and there is always gaseous water (water vapor) above a sample of liquid water. As a gas is collected over water, it becomes saturated with water vapor and the total pressure of the mixture equals the partial pressure of the gas plus the partial pressure of the water vapor. The pressure of the pure gas is therefore equal to the total pressure minus the pressure of the water vapor—this is referred to as the “dry” gas pressure, that is, the pressure of the gas only, without water vapor. The vapor pressure of water, which is the pressure exerted by water vapor in equilibrium with liquid water in a closed container, depends on the temperature ((Figure)); more detailed information on the temperature dependence of water vapor can be found in (Figure), and vapor pressure will be discussed in more detail in the chapter on liquids.
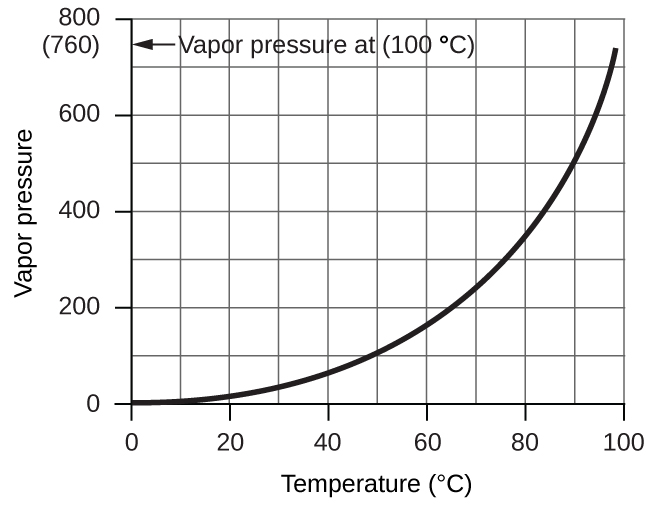
| Vapor Pressure of Ice and Water in Various Temperatures at Sea Level | |||||||
|---|---|---|---|---|---|---|---|
| Temperature (°C) | Pressure (torr) | Temperature (°C) | Pressure (torr) | Temperature (°C) | Pressure (torr) | ||
| –10 | 1.95 | 18 | 15.5 | 30 | 31.8 | ||
| –5 | 3.0 | 19 | 16.5 | 35 | 42.2 | ||
| –2 | 3.9 | 20 | 17.5 | 40 | 55.3 | ||
| 0 | 4.6 | 21 | 18.7 | 50 | 92.5 | ||
| 2 | 5.3 | 22 | 19.8 | 60 | 149.4 | ||
| 4 | 6.1 | 23 | 21.1 | 70 | 233.7 | ||
| 6 | 7.0 | 24 | 22.4 | 80 | 355.1 | ||
| 8 | 8.0 | 25 | 23.8 | 90 | 525.8 | ||
| 10 | 9.2 | 26 | 25.2 | 95 | 633.9 | ||
| 12 | 10.5 | 27 | 26.7 | 99 | 733.2 | ||
| 14 | 12.0 | 28 | 28.3 | 100.0 | 760.0 | ||
| 16 | 13.6 | 29 | 30.0 | 101.0 | 787.6 | ||
Pressure of a Gas Collected Over Water If 0.200 L of argon is collected over water at a temperature of 26 °C and a pressure of 750 torr in a system like that shown in (Figure), what is the partial pressure of argon?
Solution According to Dalton’s law, the total pressure in the bottle (750 torr) is the sum of the partial pressure of argon and the partial pressure of gaseous water:
Rearranging this equation to solve for the pressure of argon gives:
The pressure of water vapor above a sample of liquid water at 26 °C is 25.2 torr (Appendix E), so:
Check Your Learning A sample of oxygen collected over water at a temperature of 29.0 °C and a pressure of 764 torr has a volume of 0.560 L. What volume would the dry oxygen have under the same conditions of temperature and pressure?
0.583 L
Chemical Stoichiometry and Gases
Chemical stoichiometry describes the quantitative relationships between reactants and products in chemical reactions.
We have previously measured quantities of reactants and products using masses for solids and volumes in conjunction with the molarity for solutions; now we can also use gas volumes to indicate quantities. If we know the volume, pressure, and temperature of a gas, we can use the ideal gas equation to calculate how many moles of the gas are present. If we know how many moles of a gas are involved, we can calculate the volume of a gas at any temperature and pressure.
Avogadro’s Law Revisited
Sometimes we can take advantage of a simplifying feature of the stoichiometry of gases that solids and solutions do not exhibit: All gases that show ideal behavior contain the same number of molecules in the same volume (at the same temperature and pressure). Thus, the ratios of volumes of gases involved in a chemical reaction are given by the coefficients in the equation for the reaction, provided that the gas volumes are measured at the same temperature and pressure.
We can extend Avogadro’s law (that the volume of a gas is directly proportional to the number of moles of the gas) to chemical reactions with gases: Gases combine, or react, in definite and simple proportions by volume, provided that all gas volumes are measured at the same temperature and pressure. For example, since nitrogen and hydrogen gases react to produce ammonia gas according to \({\text{N}}_{2}\left(g\right)+3{\text{H}}_{2}\left(g\right)\phantom{\rule{0.4em}{0ex}}⟶\phantom{\rule{0.4em}{0ex}}2{\text{NH}}_{3}\left(g\right),\) a given volume of nitrogen gas reacts with three times that volume of hydrogen gas to produce two times that volume of ammonia gas, if pressure and temperature remain constant.
The explanation for this is illustrated in (Figure). According to Avogadro’s law, equal volumes of gaseous N2, H2, and NH3, at the same temperature and pressure, contain the same number of molecules. Because one molecule of N2 reacts with three molecules of H2 to produce two molecules of NH3, the volume of H2 required is three times the volume of N2, and the volume of NH3 produced is two times the volume of N2.<!-- no-selfclose -->
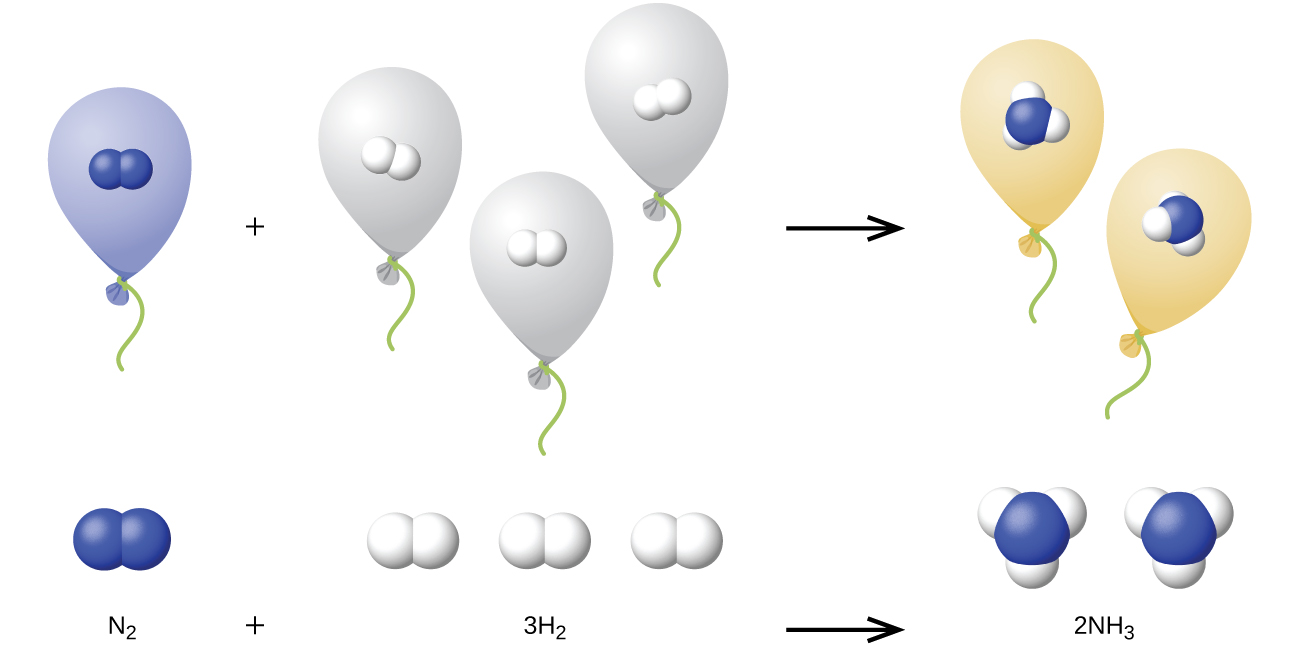
Reaction of Gases Propane, C3H8(g), is used in gas grills to provide the heat for cooking. What volume of O2(g) measured at 25 °C and 760 torr is required to react with 2.7 L of propane measured under the same conditions of temperature and pressure? Assume that the propane undergoes complete combustion.
Solution The ratio of the volumes of C3H8 and O2 will be equal to the ratio of their coefficients in the balanced equation for the reaction:
From the equation, we see that one volume of C3H8 will react with five volumes of O2:
A volume of 13.5 L of O2 will be required to react with 2.7 L of C3H8.
Check Your Learning An acetylene tank for an oxyacetylene welding torch provides 9340 L of acetylene gas, C2H2, at 0 °C and 1 atm. How many tanks of oxygen, each providing 7.00 \(×\) 103 L of O2 at 0 °C and 1 atm, will be required to burn the acetylene?
3.34 tanks (2.34 \(×\) 104 L)
Volumes of Reacting Gases Ammonia is an important fertilizer and industrial chemical. Suppose that a volume of 683 billion cubic feet of gaseous ammonia, measured at 25 °C and 1 atm, was manufactured. What volume of H2(g), measured under the same conditions, was required to prepare this amount of ammonia by reaction with N2?
Solution Because equal volumes of H2 and NH3 contain equal numbers of molecules and each three molecules of H2 that react produce two molecules of NH3, the ratio of the volumes of H2 and NH3 will be equal to 3:2. Two volumes of NH3, in this case in units of billion ft3, will be formed from three volumes of H2:
The manufacture of 683 billion ft3 of NH3 required 1020 billion ft3 of H2. (At 25 °C and 1 atm, this is the volume of a cube with an edge length of approximately 1.9 miles.)
Check Your Learning What volume of O2(g) measured at 25 °C and 760 torr is required to react with 17.0 L of ethylene, C2H4(g), measured under the same conditions of temperature and pressure? The products are CO2 and water vapor.
51.0 L
Volume of Gaseous Product What volume of hydrogen at 27 °C and 723 torr may be prepared by the reaction of 8.88 g of gallium with an excess of hydrochloric acid?
Solution
Convert the provided mass of the limiting reactant, Ga, to moles of hydrogen produced:
Convert the provided temperature and pressure values to appropriate units (K and atm, respectively), and then use the molar amount of hydrogen gas and the ideal gas equation to calculate the volume of gas:
Check Your Learning Sulfur dioxide is an intermediate in the preparation of sulfuric acid. What volume of SO2 at 343 °C and 1.21 atm is produced by burning l.00 kg of sulfur in excess oxygen?
1.30 \(×\) 103 L
The thin skin of our atmosphere keeps the earth from being an ice planet and makes it habitable. In fact, this is due to less than 0.5% of the air molecules. Of the energy from the sun that reaches the earth, almost \(\frac{1}{3}\) is reflected back into space, with the rest absorbed by the atmosphere and the surface of the earth. Some of the energy that the earth absorbs is re-emitted as infrared (IR) radiation, a portion of which passes back out through the atmosphere into space. Most of this IR radiation, however, is absorbed by certain atmospheric gases, effectively trapping heat within the atmosphere in a phenomenon known as the greenhouse effect. This effect maintains global temperatures within the range needed to sustain life on earth. Without our atmosphere, the earth's average temperature would be lower by more than 30 °C (nearly 60 °F). The major greenhouse gases (GHGs) are water vapor, carbon dioxide, methane, and ozone. Since the Industrial Revolution, human activity has been increasing the concentrations of GHGs, which have changed the energy balance and are significantly altering the earth’s climate ((Figure)).

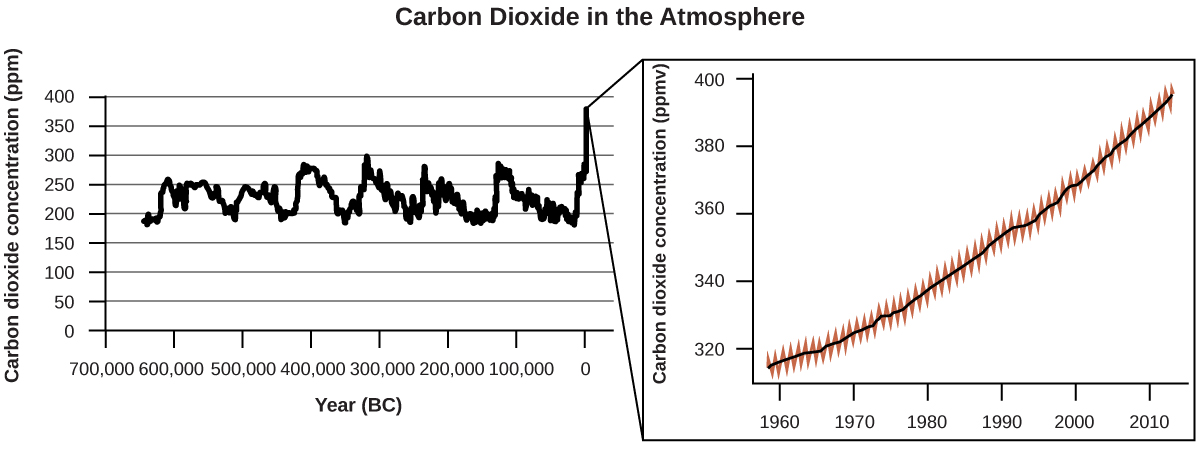
There is strong evidence from multiple sources that higher atmospheric levels of CO2 are caused by human activity, with fossil fuel burning accounting for about \(\frac{3}{4}\) of the recent increase in CO2. Reliable data from ice cores reveals that CO2 concentration in the atmosphere is at the highest level in the past 800,000 years; other evidence indicates that it may be at its highest level in 20 million years. In recent years, the CO2 concentration has increased from preindustrial levels of ~280 ppm to more than 400 ppm today ((Figure)).
Click here to see a 2-minute video explaining greenhouse gases and global warming.
Atmospheric and climate scientist Susan Solomon ((Figure)) is the author of one of The New York Times books of the year (The Coldest March, 2001), one of Time magazine’s 100 most influential people in the world (2008), and a working group leader of the Intergovernmental Panel on Climate Change (IPCC), which was the recipient of the 2007 Nobel Peace Prize. She helped determine and explain the cause of the formation of the ozone hole over Antarctica, and has authored many important papers on climate change. She has been awarded the top scientific honors in the US and France (the National Medal of Science and the Grande Medaille, respectively), and is a member of the National Academy of Sciences, the Royal Society, the French Academy of Sciences, and the European Academy of Sciences. Formerly a professor at the University of Colorado, she is now at MIT, and continues to work at NOAA.
For more information, watch this video about Susan Solomon.

Key Concepts and Summary
The ideal gas law can be used to derive a number of convenient equations relating directly measured quantities to properties of interest for gaseous substances and mixtures. Appropriate rearrangement of the ideal gas equation may be made to permit the calculation of gas densities and molar masses. Dalton’s law of partial pressures may be used to relate measured gas pressures for gaseous mixtures to their compositions. Avogadro’s law may be used in stoichiometric computations for chemical reactions involving gaseous reactants or products.
Key Equations
- PTotal = PA + PB + PC + … = ƩiPi
- PA = XA PTotal
- \({X}_{A}=\phantom{\rule{0.2em}{0ex}}\frac{{n}_{A}}{{n}_{Total}}\)
Chemistry End of Chapter Exercises
What is the density of laughing gas, dinitrogen monoxide, N2O, at a temperature of 325 K and a pressure of 113.0 kPa?
Calculate the density of Freon 12, CF2Cl2, at 30.0 °C and 0.954 atm.
4.64 g L−1
Which is denser at the same temperature and pressure, dry air or air saturated with water vapor? Explain.
A cylinder of O2(g) used in breathing by patients with emphysema has a volume of 3.00 L at a pressure of 10.0 atm. If the temperature of the cylinder is 28.0 °C, what mass of oxygen is in the cylinder?
38.8 g
What is the molar mass of a gas if 0.0494 g of the gas occupies a volume of 0.100 L at a temperature 26 °C and a pressure of 307 torr?
What is the molar mass of a gas if 0.281 g of the gas occupies a volume of 125 mL at a temperature 126 °C and a pressure of 777 torr?
72.0 g mol−1
How could you show experimentally that the molecular formula of propene is C3H6, not CH2?
The density of a certain gaseous fluoride of phosphorus is 3.93 g/L at STP. Calculate the molar mass of this fluoride and determine its molecular formula.
88.1 g mol−1; PF3
Consider this question: What is the molecular formula of a compound that contains 39% C, 45% N, and 16% H if 0.157 g of the compound occupies 125 mL with a pressure of 99.5 kPa at 22 °C?
(a) Outline the steps necessary to answer the question.
(b) Answer the question.
A 36.0–L cylinder of a gas used for calibration of blood gas analyzers in medical laboratories contains 350 g CO2, 805 g O2, and 4,880 g N2. At 25 degrees C, what is the pressure in the cylinder in atmospheres?
141 atm
A cylinder of a gas mixture used for calibration of blood gas analyzers in medical laboratories contains 5.0% CO2, 12.0% O2, and the remainder N2 at a total pressure of 146 atm. What is the partial pressure of each component of this gas? (The percentages given indicate the percent of the total pressure that is due to each component.)
A sample of gas isolated from unrefined petroleum contains 90.0% CH4, 8.9% C2H6, and 1.1% C3H8 at a total pressure of 307.2 kPa. What is the partial pressure of each component of this gas? (The percentages given indicate the percent of the total pressure that is due to each component.)
CH4: 276 kPa; C2H6: 27 kPa; C3H8: 3.4 kPa
A mixture of 0.200 g of H2, 1.00 g of N2, and 0.820 g of Ar is stored in a closed container at STP. Find the volume of the container, assuming that the gases exhibit ideal behavior.
Most mixtures of hydrogen gas with oxygen gas are explosive. However, a mixture that contains less than 3.0 % O2 is not. If enough O2 is added to a cylinder of H2 at 33.2 atm to bring the total pressure to 34.5 atm, is the mixture explosive?
Yes
A commercial mercury vapor analyzer can detect, in air, concentrations of gaseous Hg atoms (which are poisonous) as low as 2 \(×\) 10−6 mg/L of air. At this concentration, what is the partial pressure of gaseous mercury if the atmospheric pressure is 733 torr at 26 °C?
A sample of carbon monoxide was collected over water at a total pressure of 756 torr and a temperature of 18 °C. What is the pressure of the carbon monoxide? (See (Figure) for the vapor pressure of water.)
740 torr
In an experiment in a general chemistry laboratory, a student collected a sample of a gas over water. The volume of the gas was 265 mL at a pressure of 753 torr and a temperature of 27 °C. The mass of the gas was 0.472 g. What was the molar mass of the gas?
Joseph Priestley first prepared pure oxygen by heating mercuric oxide, HgO:
\(2\text{HgO}\left(s\right)\phantom{\rule{0.2em}{0ex}}⟶\phantom{\rule{0.2em}{0ex}}2\text{Hg}\left(l\right)+{\text{O}}_{2}\left(g\right)\)
(a) Outline the steps necessary to answer the following question: What volume of O2 at 23 °C and 0.975 atm is produced by the decomposition of 5.36 g of HgO?
(b) Answer the question.
(a) Determine the moles of HgO that decompose; using the chemical equation, determine the moles of O2 produced by decomposition of this amount of HgO; and determine the volume of O2 from the moles of O2, temperature, and pressure. (b) 0.308 L
Cavendish prepared hydrogen in 1766 by the novel method of passing steam through a red-hot gun barrel:
\(4{\text{H}}_{2}\text{O}\left(g\right)+3\text{Fe}\left(s\right)\phantom{\rule{0.2em}{0ex}}⟶\phantom{\rule{0.2em}{0ex}}{\text{Fe}}_{3}{\text{O}}_{4}\left(s\right)+4{\text{H}}_{2}\left(g\right)\)
(a) Outline the steps necessary to answer the following question: What volume of H2 at a pressure of 745 torr and a temperature of 20 °C can be prepared from the reaction of 15.O g of H2O?
(b) Answer the question.
The chlorofluorocarbon CCl2F2 can be recycled into a different compound by reaction with hydrogen to produce CH2F2(g), a compound useful in chemical manufacturing:
\({\text{CCl}}_{2}{\text{F}}_{2}\left(g\right)+4{\text{H}}_{2}\left(g\right)\phantom{\rule{0.2em}{0ex}}⟶\phantom{\rule{0.2em}{0ex}}{\text{CH}}_{2}{\text{F}}_{2}\left(g\right)+2\text{HCl}\left(g\right)\)
(a) Outline the steps necessary to answer the following question: What volume of hydrogen at 225 atm and 35.5 °C would be required to react with 1 ton (1.000 \(×\) 103 kg) of CCl2F2?
(b) Answer the question.
(a) Determine the molar mass of CCl2F2. From the balanced equation, calculate the moles of H2 needed for the complete reaction. From the ideal gas law, convert moles of H2 into volume. (b) 3.72 \(×\) 103 L
Automobile air bags are inflated with nitrogen gas, which is formed by the decomposition of solid sodium azide (NaN3). The other product is sodium metal. Calculate the volume of nitrogen gas at 27 °C and 756 torr formed by the decomposition of 125 g of sodium azide.
Lime, CaO, is produced by heating calcium carbonate, CaCO3; carbon dioxide is the other product.
(a) Outline the steps necessary to answer the following question: What volume of carbon dioxide at 875° and 0.966 atm is produced by the decomposition of 1 ton (1.000 \(×\) 103 kg) of calcium carbonate?
(b) Answer the question.
(a) Balance the equation. Determine the grams of CO2 produced and the number of moles. From the ideal gas law, determine the volume of gas. (b) 7.43 \(×\) 105 L
Before small batteries were available, carbide lamps were used for bicycle lights. Acetylene gas, C2H2, and solid calcium hydroxide were formed by the reaction of calcium carbide, CaC2, with water. The ignition of the acetylene gas provided the light. Currently, the same lamps are used by some cavers, and calcium carbide is used to produce acetylene for carbide cannons.
(a) Outline the steps necessary to answer the following question: What volume of C2H2 at 1.005 atm and 12.2 °C is formed by the reaction of 15.48 g of CaC2 with water?
(b) Answer the question.
Calculate the volume of oxygen required to burn 12.00 L of ethane gas, C2H6, to produce carbon dioxide and water, if the volumes of C2H6 and O2 are measured under the same conditions of temperature and pressure.
42.00 L
What volume of O2 at STP is required to oxidize 8.0 L of NO at STP to NO2? What volume of NO2 is produced at STP?
Consider the following questions:
(a) What is the total volume of the CO2(g) and H2O(g) at 600 °C and 0.888 atm produced by the combustion of 1.00 L of C2H6(g) measured at STP?
(b) What is the partial pressure of H2O in the product gases?
(a) 18.0 L; (b) 0.533 atm
Methanol, CH3OH, is produced industrially by the following reaction:
\(\text{CO}\left(g\right)+2{\text{H}}_{2}\left(g\right)\phantom{\rule{0.2em}{0ex}}\stackrel{\phantom{\rule{2em}{0ex}}\text{copper catalyst 300 °C, 300 atm}\phantom{\rule{2em}{0ex}}}{\to }\phantom{\rule{0.2em}{0ex}}{\text{CH}}_{3}\text{OH}\left(g\right)\)
Assuming that the gases behave as ideal gases, find the ratio of the total volume of the reactants to the final volume.
What volume of oxygen at 423.0 K and a pressure of 127.4 kPa is produced by the decomposition of 129.7 g of BaO2 to BaO and O2?
10.57 L O2
A 2.50-L sample of a colorless gas at STP decomposed to give 2.50 L of N2 and 1.25 L of O2 at STP. What is the colorless gas?
Ethanol, C2H5OH, is produced industrially from ethylene, C2H4, by the following sequence of reactions:
\(3{\text{C}}_{2}{\text{H}}_{4}+2{\text{H}}_{2}{\text{SO}}_{4}\phantom{\rule{0.2em}{0ex}}⟶\phantom{\rule{0.2em}{0ex}}{\text{C}}_{2}{\text{H}}_{5}{\text{HSO}}_{4}+{\left({\text{C}}_{2}{\text{H}}_{5}\right)}_{2}{\text{SO}}_{4}\)
\({\text{C}}_{2}{\text{H}}_{5}{\text{HSO}}_{4}+{\left({\text{C}}_{2}{\text{H}}_{5}\right)}_{2}{\text{SO}}_{4}+3{\text{H}}_{2}\text{O}\phantom{\rule{0.2em}{0ex}}⟶\phantom{\rule{0.2em}{0ex}}3{\text{C}}_{2}{\text{H}}_{5}\text{OH}+2{\text{H}}_{2}{\text{SO}}_{4}\)
What volume of ethylene at STP is required to produce 1.000 metric ton (1000 kg) of ethanol if the overall yield of ethanol is 90.1%?
5.40 \(×\) 105 L
One molecule of hemoglobin will combine with four molecules of oxygen. If 1.0 g of hemoglobin combines with 1.53 mL of oxygen at body temperature (37 °C) and a pressure of 743 torr, what is the molar mass of hemoglobin?
A sample of a compound of xenon and fluorine was confined in a bulb with a pressure of 18 torr. Hydrogen was added to the bulb until the pressure was 72 torr. Passage of an electric spark through the mixture produced Xe and HF. After the HF was removed by reaction with solid KOH, the final pressure of xenon and unreacted hydrogen in the bulb was 36 torr. What is the empirical formula of the xenon fluoride in the original sample? (Note: Xenon fluorides contain only one xenon atom per molecule.)
XeF2
One method of analyzing amino acids is the van Slyke method. The characteristic amino groups (−NH2) in protein material are allowed to react with nitrous acid, HNO2, to form N2 gas. From the volume of the gas, the amount of amino acid can be determined. A 0.0604-g sample of a biological sample containing glycine, CH2(NH2)COOH, was analyzed by the van Slyke method and yielded 3.70 mL of N2 collected over water at a pressure of 735 torr and 29 °C. What was the percentage of glycine in the sample?
\({\text{CH}}_{2}\left({\text{NH}}_{2}\right){\text{CO}}_{2}\text{H}+{\text{HNO}}_{2}\phantom{\rule{0.2em}{0ex}}⟶\phantom{\rule{0.2em}{0ex}}{\text{CH}}_{2}\left(\text{OH}\right){\text{CO}}_{2}\text{H}+{\text{H}}_{2}\text{O}+{\text{N}}_{2}\)
Footnotes
- 1“Quotations by Joseph-Louis Lagrange,” last modified February 2006, accessed February 10, 2015, http://www-history.mcs.st-andrews.ac.uk/Quotations/Lagrange.html
Glossary
- Dalton’s law of partial pressures
- total pressure of a mixture of ideal gases is equal to the sum of the partial pressures of the component gases.
- mole fraction (X)
- concentration unit defined as the ratio of the molar amount of a mixture component to the total number of moles of all mixture components
- partial pressure
- pressure exerted by an individual gas in a mixture
- vapor pressure of water
- pressure exerted by water vapor in equilibrium with liquid water in a closed container at a specific temperature
[latexpage]
Learning Objectives
By the end of this section, you will be able to:
- Use the ideal gas law to compute gas densities and molar masses
- Perform stoichiometric calculations involving gaseous substances
- State Dalton’s law of partial pressures and use it in calculations involving gaseous mixtures
The study of the chemical behavior of gases was part of the basis of perhaps the most fundamental chemical revolution in history. French nobleman Antoine Lavoisier, widely regarded as the “father of modern chemistry,” changed chemistry from a qualitative to a quantitative science through his work with gases. He discovered the law of conservation of matter, discovered the role of oxygen in combustion reactions, determined the composition of air, explained respiration in terms of chemical reactions, and more. He was a casualty of the French Revolution, guillotined in 1794. Of his death, mathematician and astronomer Joseph-Louis Lagrange said, “It took the mob only a moment to remove his head; a century will not suffice to reproduce it.”1
As described in an earlier chapter of this text, we can turn to chemical stoichiometry for answers to many of the questions that ask “How much?” The essential property involved in such use of stoichiometry is the amount of substance, typically measured in moles (n). For gases, molar amount can be derived from convenient experimental measurements of pressure, temperature, and volume. Therefore, these measurements are useful in assessing the stoichiometry of pure gases, gas mixtures, and chemical reactions involving gases. This section will not introduce any new material or ideas, but will provide examples of applications and ways to integrate concepts we have already discussed.
Gas Density and Molar Mass
The ideal gas law described previously in this chapter relates the properties of pressure P, volume V, temperature T, and molar amount n. This law is universal, relating these properties in identical fashion regardless of the chemical identity of the gas:
The density d of a gas, on the other hand, is determined by its identity. As described in another chapter of this text, the density of a substance is a characteristic property that may be used to identify the substance.
Rearranging the ideal gas equation to isolate V and substituting into the density equation yields
The ratio m/n is the definition of molar mass, ℳ:
The density equation can then be written
This relation may be used for calculating the densities of gases of known identities at specified values of pressure and temperature as demonstrated in (Figure).
Measuring Gas Density What is the density of molecular nitrogen gas at STP?
Solution The molar mass of molecular nitrogen, N2, is 28.01 g/mol. Substituting this value along with standard temperature and pressure into the gas density equation yields
Check Your Learning What is the density of molecular hydrogen gas at 17.0 °C and a pressure of 760 torr?
d = 0.0847 g/L
When the identity of a gas is unknown, measurements of the mass, pressure, volume, and temperature of a sample can be used to calculate the molar mass of the gas (a useful property for identification purposes). Combining the ideal gas equation
and the definition of molarity
yields the following equation:
Determining the molar mass of a gas via this approach is demonstrated in (Figure).
Determining the Molecular Formula of a Gas from its Molar Mass and Empirical Formula Cyclopropane, a gas once used with oxygen as a general anesthetic, is composed of 85.7% carbon and 14.3% hydrogen by mass. Find the empirical formula. If 1.56 g of cyclopropane occupies a volume of 1.00 L at 0.984 atm and 50 °C, what is the molecular formula for cyclopropane?
Solution First determine the empirical formula of the gas. Assume 100 g and convert the percentage of each element into grams. Determine the number of moles of carbon and hydrogen in the 100-g sample of cyclopropane. Divide by the smallest number of moles to relate the number of moles of carbon to the number of moles of hydrogen. In the last step, realize that the smallest whole number ratio is the empirical formula:
Empirical formula is CH2 [empirical mass (EM) of 14.03 g/empirical unit].
Next, use the provided values for mass, pressure, temperature and volume to compute the molar mass of the gas:
Comparing the molar mass to the empirical formula mass shows how many empirical formula units make up a molecule:
The molecular formula is thus derived from the empirical formula by multiplying each of its subscripts by three:
Check Your LearningAcetylene, a fuel used welding torches, is composed of 92.3% C and 7.7% H by mass. Find the empirical formula. If 1.10 g of acetylene occupies of volume of 1.00 L at 1.15 atm and 59.5 °C, what is the molecular formula for acetylene?
Empirical formula, CH; Molecular formula, C2H2
Determining the Molar Mass of a Volatile Liquid The approximate molar mass of a volatile liquid can be determined by:
- Heating a sample of the liquid in a flask with a tiny hole at the top, which converts the liquid into gas that may escape through the hole
- Removing the flask from heat at the instant when the last bit of liquid becomes gas, at which time the flask will be filled with only gaseous sample at ambient pressure
- Sealing the flask and permitting the gaseous sample to condense to liquid, and then weighing the flask to determine the sample’s mass (see (Figure))

Using this procedure, a sample of chloroform gas weighing 0.494 g is collected in a flask with a volume of 129 cm3 at 99.6 °C when the atmospheric pressure is 742.1 mm Hg. What is the approximate molar mass of chloroform?
Solution Since \(\text{ℳ}=\phantom{\rule{0.2em}{0ex}}\frac{m}{n}\) and \(n=\phantom{\rule{0.2em}{0ex}}\frac{PV}{RT},\) substituting and rearranging gives \(\text{ℳ}=\phantom{\rule{0.2em}{0ex}}\frac{mRT}{PV},\)
then
Check Your Learning A sample of phosphorus that weighs 3.243 \(×\) 10−2 g exerts a pressure of 31.89 kPa in a 56.0-mL bulb at 550 °C. What are the molar mass and molecular formula of phosphorus vapor?
124 g/mol P4
The Pressure of a Mixture of Gases: Dalton’s Law
Unless they chemically react with each other, the individual gases in a mixture of gases do not affect each other’s pressure. Each individual gas in a mixture exerts the same pressure that it would exert if it were present alone in the container ((Figure)). The pressure exerted by each individual gas in a mixture is called its partial pressure. This observation is summarized by Dalton’s law of partial pressures: The total pressure of a mixture of ideal gases is equal to the sum of the partial pressures of the component gases:
In the equation PTotal is the total pressure of a mixture of gases, PA is the partial pressure of gas A; PB is the partial pressure of gas B; PC is the partial pressure of gas C; and so on.

The partial pressure of gas A is related to the total pressure of the gas mixture via its mole fraction (X), a unit of concentration defined as the number of moles of a component of a solution divided by the total number of moles of all components:
where PA, XA, and nA are the partial pressure, mole fraction, and number of moles of gas A, respectively, and nTotal is the number of moles of all components in the mixture.
The Pressure of a Mixture of Gases A 10.0-L vessel contains 2.50 \(×\) 10−3 mol of H2, 1.00 \(×\) 10−3 mol of He, and 3.00 \(×\) 10−4 mol of Ne at 35 °C.
(a) What are the partial pressures of each of the gases?
(b) What is the total pressure in atmospheres?
SolutionThe gases behave independently, so the partial pressure of each gas can be determined from the ideal gas equation, using \(P=\phantom{\rule{0.2em}{0ex}}\frac{nRT}{V}\):
The total pressure is given by the sum of the partial pressures:
Check Your Learning A 5.73-L flask at 25 °C contains 0.0388 mol of N2, 0.147 mol of CO, and 0.0803 mol of H2. What is the total pressure in the flask in atmospheres?
1.137 atm
Here is another example of this concept, but dealing with mole fraction calculations.
The Pressure of a Mixture of Gases A gas mixture used for anesthesia contains 2.83 mol oxygen, O2, and 8.41 mol nitrous oxide, N2O. The total pressure of the mixture is 192 kPa.
(a) What are the mole fractions of O2 and N2O?
(b) What are the partial pressures of O2 and N2O?
Solution The mole fraction is given by \({X}_{A}=\phantom{\rule{0.2em}{0ex}}\frac{{n}_{A}}{{n}_{Total}}\) and the partial pressure is PA = XA \(×\) PTotal.
For O2,
and \({P}_{{O}_{2}}={X}_{{O}_{2}}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{P}_{Total}=0.252\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\text{192 kPa}=\text{48.4 kPa}\)
For N2O,
and
\({P}_{{N}_{2}}={X}_{{N}_{2}}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{P}_{\mathrm{Total}}=0.748\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\text{192 kPa}=\text{143.6 kPa}\)
Check Your Learning What is the pressure of a mixture of 0.200 g of H2, 1.00 g of N2, and 0.820 g of Ar in a container with a volume of 2.00 L at 20 °C?
1.87 atm
Collection of Gases over Water
A simple way to collect gases that do not react with water is to capture them in a bottle that has been filled with water and inverted into a dish filled with water. The pressure of the gas inside the bottle can be made equal to the air pressure outside by raising or lowering the bottle. When the water level is the same both inside and outside the bottle ((Figure)), the pressure of the gas is equal to the atmospheric pressure, which can be measured with a barometer.

However, there is another factor we must consider when we measure the pressure of the gas by this method. Water evaporates and there is always gaseous water (water vapor) above a sample of liquid water. As a gas is collected over water, it becomes saturated with water vapor and the total pressure of the mixture equals the partial pressure of the gas plus the partial pressure of the water vapor. The pressure of the pure gas is therefore equal to the total pressure minus the pressure of the water vapor—this is referred to as the “dry” gas pressure, that is, the pressure of the gas only, without water vapor. The vapor pressure of water, which is the pressure exerted by water vapor in equilibrium with liquid water in a closed container, depends on the temperature ((Figure)); more detailed information on the temperature dependence of water vapor can be found in (Figure), and vapor pressure will be discussed in more detail in the chapter on liquids.

| Vapor Pressure of Ice and Water in Various Temperatures at Sea Level | |||||||
|---|---|---|---|---|---|---|---|
| Temperature (°C) | Pressure (torr) | Temperature (°C) | Pressure (torr) | Temperature (°C) | Pressure (torr) | ||
| –10 | 1.95 | 18 | 15.5 | 30 | 31.8 | ||
| –5 | 3.0 | 19 | 16.5 | 35 | 42.2 | ||
| –2 | 3.9 | 20 | 17.5 | 40 | 55.3 | ||
| 0 | 4.6 | 21 | 18.7 | 50 | 92.5 | ||
| 2 | 5.3 | 22 | 19.8 | 60 | 149.4 | ||
| 4 | 6.1 | 23 | 21.1 | 70 | 233.7 | ||
| 6 | 7.0 | 24 | 22.4 | 80 | 355.1 | ||
| 8 | 8.0 | 25 | 23.8 | 90 | 525.8 | ||
| 10 | 9.2 | 26 | 25.2 | 95 | 633.9 | ||
| 12 | 10.5 | 27 | 26.7 | 99 | 733.2 | ||
| 14 | 12.0 | 28 | 28.3 | 100.0 | 760.0 | ||
| 16 | 13.6 | 29 | 30.0 | 101.0 | 787.6 | ||
Pressure of a Gas Collected Over Water If 0.200 L of argon is collected over water at a temperature of 26 °C and a pressure of 750 torr in a system like that shown in (Figure), what is the partial pressure of argon?
Solution According to Dalton’s law, the total pressure in the bottle (750 torr) is the sum of the partial pressure of argon and the partial pressure of gaseous water:
Rearranging this equation to solve for the pressure of argon gives:
The pressure of water vapor above a sample of liquid water at 26 °C is 25.2 torr (Appendix E), so:
Check Your Learning A sample of oxygen collected over water at a temperature of 29.0 °C and a pressure of 764 torr has a volume of 0.560 L. What volume would the dry oxygen have under the same conditions of temperature and pressure?
0.583 L
Chemical Stoichiometry and Gases
Chemical stoichiometry describes the quantitative relationships between reactants and products in chemical reactions.
We have previously measured quantities of reactants and products using masses for solids and volumes in conjunction with the molarity for solutions; now we can also use gas volumes to indicate quantities. If we know the volume, pressure, and temperature of a gas, we can use the ideal gas equation to calculate how many moles of the gas are present. If we know how many moles of a gas are involved, we can calculate the volume of a gas at any temperature and pressure.
Avogadro’s Law Revisited
Sometimes we can take advantage of a simplifying feature of the stoichiometry of gases that solids and solutions do not exhibit: All gases that show ideal behavior contain the same number of molecules in the same volume (at the same temperature and pressure). Thus, the ratios of volumes of gases involved in a chemical reaction are given by the coefficients in the equation for the reaction, provided that the gas volumes are measured at the same temperature and pressure.
We can extend Avogadro’s law (that the volume of a gas is directly proportional to the number of moles of the gas) to chemical reactions with gases: Gases combine, or react, in definite and simple proportions by volume, provided that all gas volumes are measured at the same temperature and pressure. For example, since nitrogen and hydrogen gases react to produce ammonia gas according to \({\text{N}}_{2}\left(g\right)+3{\text{H}}_{2}\left(g\right)\phantom{\rule{0.4em}{0ex}}⟶\phantom{\rule{0.4em}{0ex}}2{\text{NH}}_{3}\left(g\right),\) a given volume of nitrogen gas reacts with three times that volume of hydrogen gas to produce two times that volume of ammonia gas, if pressure and temperature remain constant.
The explanation for this is illustrated in (Figure). According to Avogadro’s law, equal volumes of gaseous N2, H2, and NH3, at the same temperature and pressure, contain the same number of molecules. Because one molecule of N2 reacts with three molecules of H2 to produce two molecules of NH3, the volume of H2 required is three times the volume of N2, and the volume of NH3 produced is two times the volume of N2.<!-- no-selfclose -->

Reaction of Gases Propane, C3H8(g), is used in gas grills to provide the heat for cooking. What volume of O2(g) measured at 25 °C and 760 torr is required to react with 2.7 L of propane measured under the same conditions of temperature and pressure? Assume that the propane undergoes complete combustion.
Solution The ratio of the volumes of C3H8 and O2 will be equal to the ratio of their coefficients in the balanced equation for the reaction:
From the equation, we see that one volume of C3H8 will react with five volumes of O2:
A volume of 13.5 L of O2 will be required to react with 2.7 L of C3H8.
Check Your Learning An acetylene tank for an oxyacetylene welding torch provides 9340 L of acetylene gas, C2H2, at 0 °C and 1 atm. How many tanks of oxygen, each providing 7.00 \(×\) 103 L of O2 at 0 °C and 1 atm, will be required to burn the acetylene?
3.34 tanks (2.34 \(×\) 104 L)
Volumes of Reacting Gases Ammonia is an important fertilizer and industrial chemical. Suppose that a volume of 683 billion cubic feet of gaseous ammonia, measured at 25 °C and 1 atm, was manufactured. What volume of H2(g), measured under the same conditions, was required to prepare this amount of ammonia by reaction with N2?
Solution Because equal volumes of H2 and NH3 contain equal numbers of molecules and each three molecules of H2 that react produce two molecules of NH3, the ratio of the volumes of H2 and NH3 will be equal to 3:2. Two volumes of NH3, in this case in units of billion ft3, will be formed from three volumes of H2:
The manufacture of 683 billion ft3 of NH3 required 1020 billion ft3 of H2. (At 25 °C and 1 atm, this is the volume of a cube with an edge length of approximately 1.9 miles.)
Check Your Learning What volume of O2(g) measured at 25 °C and 760 torr is required to react with 17.0 L of ethylene, C2H4(g), measured under the same conditions of temperature and pressure? The products are CO2 and water vapor.
51.0 L
Volume of Gaseous Product What volume of hydrogen at 27 °C and 723 torr may be prepared by the reaction of 8.88 g of gallium with an excess of hydrochloric acid?
Solution
Convert the provided mass of the limiting reactant, Ga, to moles of hydrogen produced:
Convert the provided temperature and pressure values to appropriate units (K and atm, respectively), and then use the molar amount of hydrogen gas and the ideal gas equation to calculate the volume of gas:
Check Your Learning Sulfur dioxide is an intermediate in the preparation of sulfuric acid. What volume of SO2 at 343 °C and 1.21 atm is produced by burning l.00 kg of sulfur in excess oxygen?
1.30 \(×\) 103 L
The thin skin of our atmosphere keeps the earth from being an ice planet and makes it habitable. In fact, this is due to less than 0.5% of the air molecules. Of the energy from the sun that reaches the earth, almost \(\frac{1}{3}\) is reflected back into space, with the rest absorbed by the atmosphere and the surface of the earth. Some of the energy that the earth absorbs is re-emitted as infrared (IR) radiation, a portion of which passes back out through the atmosphere into space. Most of this IR radiation, however, is absorbed by certain atmospheric gases, effectively trapping heat within the atmosphere in a phenomenon known as the greenhouse effect. This effect maintains global temperatures within the range needed to sustain life on earth. Without our atmosphere, the earth's average temperature would be lower by more than 30 °C (nearly 60 °F). The major greenhouse gases (GHGs) are water vapor, carbon dioxide, methane, and ozone. Since the Industrial Revolution, human activity has been increasing the concentrations of GHGs, which have changed the energy balance and are significantly altering the earth’s climate ((Figure)).

There is strong evidence from multiple sources that higher atmospheric levels of CO2 are caused by human activity, with fossil fuel burning accounting for about \(\frac{3}{4}\) of the recent increase in CO2. Reliable data from ice cores reveals that CO2 concentration in the atmosphere is at the highest level in the past 800,000 years; other evidence indicates that it may be at its highest level in 20 million years. In recent years, the CO2 concentration has increased from preindustrial levels of ~280 ppm to more than 400 ppm today ((Figure)).

Click here to see a 2-minute video explaining greenhouse gases and global warming.
Atmospheric and climate scientist Susan Solomon ((Figure)) is the author of one of The New York Times books of the year (The Coldest March, 2001), one of Time magazine’s 100 most influential people in the world (2008), and a working group leader of the Intergovernmental Panel on Climate Change (IPCC), which was the recipient of the 2007 Nobel Peace Prize. She helped determine and explain the cause of the formation of the ozone hole over Antarctica, and has authored many important papers on climate change. She has been awarded the top scientific honors in the US and France (the National Medal of Science and the Grande Medaille, respectively), and is a member of the National Academy of Sciences, the Royal Society, the French Academy of Sciences, and the European Academy of Sciences. Formerly a professor at the University of Colorado, she is now at MIT, and continues to work at NOAA.
For more information, watch this video about Susan Solomon.

Key Concepts and Summary
The ideal gas law can be used to derive a number of convenient equations relating directly measured quantities to properties of interest for gaseous substances and mixtures. Appropriate rearrangement of the ideal gas equation may be made to permit the calculation of gas densities and molar masses. Dalton’s law of partial pressures may be used to relate measured gas pressures for gaseous mixtures to their compositions. Avogadro’s law may be used in stoichiometric computations for chemical reactions involving gaseous reactants or products.
Key Equations
- PTotal = PA + PB + PC + … = ƩiPi
- PA = XA PTotal
- \({X}_{A}=\phantom{\rule{0.2em}{0ex}}\frac{{n}_{A}}{{n}_{Total}}\)
Chemistry End of Chapter Exercises
What is the density of laughing gas, dinitrogen monoxide, N2O, at a temperature of 325 K and a pressure of 113.0 kPa?
Calculate the density of Freon 12, CF2Cl2, at 30.0 °C and 0.954 atm.
4.64 g L−1
Which is denser at the same temperature and pressure, dry air or air saturated with water vapor? Explain.
A cylinder of O2(g) used in breathing by patients with emphysema has a volume of 3.00 L at a pressure of 10.0 atm. If the temperature of the cylinder is 28.0 °C, what mass of oxygen is in the cylinder?
38.8 g
What is the molar mass of a gas if 0.0494 g of the gas occupies a volume of 0.100 L at a temperature 26 °C and a pressure of 307 torr?
What is the molar mass of a gas if 0.281 g of the gas occupies a volume of 125 mL at a temperature 126 °C and a pressure of 777 torr?
72.0 g mol−1
How could you show experimentally that the molecular formula of propene is C3H6, not CH2?
The density of a certain gaseous fluoride of phosphorus is 3.93 g/L at STP. Calculate the molar mass of this fluoride and determine its molecular formula.
88.1 g mol−1; PF3
Consider this question: What is the molecular formula of a compound that contains 39% C, 45% N, and 16% H if 0.157 g of the compound occupies 125 mL with a pressure of 99.5 kPa at 22 °C?
(a) Outline the steps necessary to answer the question.
(b) Answer the question.
A 36.0–L cylinder of a gas used for calibration of blood gas analyzers in medical laboratories contains 350 g CO2, 805 g O2, and 4,880 g N2. At 25 degrees C, what is the pressure in the cylinder in atmospheres?
141 atm
A cylinder of a gas mixture used for calibration of blood gas analyzers in medical laboratories contains 5.0% CO2, 12.0% O2, and the remainder N2 at a total pressure of 146 atm. What is the partial pressure of each component of this gas? (The percentages given indicate the percent of the total pressure that is due to each component.)
A sample of gas isolated from unrefined petroleum contains 90.0% CH4, 8.9% C2H6, and 1.1% C3H8 at a total pressure of 307.2 kPa. What is the partial pressure of each component of this gas? (The percentages given indicate the percent of the total pressure that is due to each component.)
CH4: 276 kPa; C2H6: 27 kPa; C3H8: 3.4 kPa
A mixture of 0.200 g of H2, 1.00 g of N2, and 0.820 g of Ar is stored in a closed container at STP. Find the volume of the container, assuming that the gases exhibit ideal behavior.
Most mixtures of hydrogen gas with oxygen gas are explosive. However, a mixture that contains less than 3.0 % O2 is not. If enough O2 is added to a cylinder of H2 at 33.2 atm to bring the total pressure to 34.5 atm, is the mixture explosive?
Yes
A commercial mercury vapor analyzer can detect, in air, concentrations of gaseous Hg atoms (which are poisonous) as low as 2 \(×\) 10−6 mg/L of air. At this concentration, what is the partial pressure of gaseous mercury if the atmospheric pressure is 733 torr at 26 °C?
A sample of carbon monoxide was collected over water at a total pressure of 756 torr and a temperature of 18 °C. What is the pressure of the carbon monoxide? (See (Figure) for the vapor pressure of water.)
740 torr
In an experiment in a general chemistry laboratory, a student collected a sample of a gas over water. The volume of the gas was 265 mL at a pressure of 753 torr and a temperature of 27 °C. The mass of the gas was 0.472 g. What was the molar mass of the gas?
Joseph Priestley first prepared pure oxygen by heating mercuric oxide, HgO:
\(2\text{HgO}\left(s\right)\phantom{\rule{0.2em}{0ex}}⟶\phantom{\rule{0.2em}{0ex}}2\text{Hg}\left(l\right)+{\text{O}}_{2}\left(g\right)\)
(a) Outline the steps necessary to answer the following question: What volume of O2 at 23 °C and 0.975 atm is produced by the decomposition of 5.36 g of HgO?
(b) Answer the question.
(a) Determine the moles of HgO that decompose; using the chemical equation, determine the moles of O2 produced by decomposition of this amount of HgO; and determine the volume of O2 from the moles of O2, temperature, and pressure. (b) 0.308 L
Cavendish prepared hydrogen in 1766 by the novel method of passing steam through a red-hot gun barrel:
\(4{\text{H}}_{2}\text{O}\left(g\right)+3\text{Fe}\left(s\right)\phantom{\rule{0.2em}{0ex}}⟶\phantom{\rule{0.2em}{0ex}}{\text{Fe}}_{3}{\text{O}}_{4}\left(s\right)+4{\text{H}}_{2}\left(g\right)\)
(a) Outline the steps necessary to answer the following question: What volume of H2 at a pressure of 745 torr and a temperature of 20 °C can be prepared from the reaction of 15.O g of H2O?
(b) Answer the question.
The chlorofluorocarbon CCl2F2 can be recycled into a different compound by reaction with hydrogen to produce CH2F2(g), a compound useful in chemical manufacturing:
\({\text{CCl}}_{2}{\text{F}}_{2}\left(g\right)+4{\text{H}}_{2}\left(g\right)\phantom{\rule{0.2em}{0ex}}⟶\phantom{\rule{0.2em}{0ex}}{\text{CH}}_{2}{\text{F}}_{2}\left(g\right)+2\text{HCl}\left(g\right)\)
(a) Outline the steps necessary to answer the following question: What volume of hydrogen at 225 atm and 35.5 °C would be required to react with 1 ton (1.000 \(×\) 103 kg) of CCl2F2?
(b) Answer the question.
(a) Determine the molar mass of CCl2F2. From the balanced equation, calculate the moles of H2 needed for the complete reaction. From the ideal gas law, convert moles of H2 into volume. (b) 3.72 \(×\) 103 L
Automobile air bags are inflated with nitrogen gas, which is formed by the decomposition of solid sodium azide (NaN3). The other product is sodium metal. Calculate the volume of nitrogen gas at 27 °C and 756 torr formed by the decomposition of 125 g of sodium azide.
Lime, CaO, is produced by heating calcium carbonate, CaCO3; carbon dioxide is the other product.
(a) Outline the steps necessary to answer the following question: What volume of carbon dioxide at 875° and 0.966 atm is produced by the decomposition of 1 ton (1.000 \(×\) 103 kg) of calcium carbonate?
(b) Answer the question.
(a) Balance the equation. Determine the grams of CO2 produced and the number of moles. From the ideal gas law, determine the volume of gas. (b) 7.43 \(×\) 105 L
Before small batteries were available, carbide lamps were used for bicycle lights. Acetylene gas, C2H2, and solid calcium hydroxide were formed by the reaction of calcium carbide, CaC2, with water. The ignition of the acetylene gas provided the light. Currently, the same lamps are used by some cavers, and calcium carbide is used to produce acetylene for carbide cannons.
(a) Outline the steps necessary to answer the following question: What volume of C2H2 at 1.005 atm and 12.2 °C is formed by the reaction of 15.48 g of CaC2 with water?
(b) Answer the question.
Calculate the volume of oxygen required to burn 12.00 L of ethane gas, C2H6, to produce carbon dioxide and water, if the volumes of C2H6 and O2 are measured under the same conditions of temperature and pressure.
42.00 L
What volume of O2 at STP is required to oxidize 8.0 L of NO at STP to NO2? What volume of NO2 is produced at STP?
Consider the following questions:
(a) What is the total volume of the CO2(g) and H2O(g) at 600 °C and 0.888 atm produced by the combustion of 1.00 L of C2H6(g) measured at STP?
(b) What is the partial pressure of H2O in the product gases?
(a) 18.0 L; (b) 0.533 atm
Methanol, CH3OH, is produced industrially by the following reaction:
\(\text{CO}\left(g\right)+2{\text{H}}_{2}\left(g\right)\phantom{\rule{0.2em}{0ex}}\stackrel{\phantom{\rule{2em}{0ex}}\text{copper catalyst 300 °C, 300 atm}\phantom{\rule{2em}{0ex}}}{\to }\phantom{\rule{0.2em}{0ex}}{\text{CH}}_{3}\text{OH}\left(g\right)\)
Assuming that the gases behave as ideal gases, find the ratio of the total volume of the reactants to the final volume.
What volume of oxygen at 423.0 K and a pressure of 127.4 kPa is produced by the decomposition of 129.7 g of BaO2 to BaO and O2?
10.57 L O2
A 2.50-L sample of a colorless gas at STP decomposed to give 2.50 L of N2 and 1.25 L of O2 at STP. What is the colorless gas?
Ethanol, C2H5OH, is produced industrially from ethylene, C2H4, by the following sequence of reactions:
\(3{\text{C}}_{2}{\text{H}}_{4}+2{\text{H}}_{2}{\text{SO}}_{4}\phantom{\rule{0.2em}{0ex}}⟶\phantom{\rule{0.2em}{0ex}}{\text{C}}_{2}{\text{H}}_{5}{\text{HSO}}_{4}+{\left({\text{C}}_{2}{\text{H}}_{5}\right)}_{2}{\text{SO}}_{4}\)
\({\text{C}}_{2}{\text{H}}_{5}{\text{HSO}}_{4}+{\left({\text{C}}_{2}{\text{H}}_{5}\right)}_{2}{\text{SO}}_{4}+3{\text{H}}_{2}\text{O}\phantom{\rule{0.2em}{0ex}}⟶\phantom{\rule{0.2em}{0ex}}3{\text{C}}_{2}{\text{H}}_{5}\text{OH}+2{\text{H}}_{2}{\text{SO}}_{4}\)
What volume of ethylene at STP is required to produce 1.000 metric ton (1000 kg) of ethanol if the overall yield of ethanol is 90.1%?
5.40 \(×\) 105 L
One molecule of hemoglobin will combine with four molecules of oxygen. If 1.0 g of hemoglobin combines with 1.53 mL of oxygen at body temperature (37 °C) and a pressure of 743 torr, what is the molar mass of hemoglobin?
A sample of a compound of xenon and fluorine was confined in a bulb with a pressure of 18 torr. Hydrogen was added to the bulb until the pressure was 72 torr. Passage of an electric spark through the mixture produced Xe and HF. After the HF was removed by reaction with solid KOH, the final pressure of xenon and unreacted hydrogen in the bulb was 36 torr. What is the empirical formula of the xenon fluoride in the original sample? (Note: Xenon fluorides contain only one xenon atom per molecule.)
XeF2
One method of analyzing amino acids is the van Slyke method. The characteristic amino groups (−NH2) in protein material are allowed to react with nitrous acid, HNO2, to form N2 gas. From the volume of the gas, the amount of amino acid can be determined. A 0.0604-g sample of a biological sample containing glycine, CH2(NH2)COOH, was analyzed by the van Slyke method and yielded 3.70 mL of N2 collected over water at a pressure of 735 torr and 29 °C. What was the percentage of glycine in the sample?
\({\text{CH}}_{2}\left({\text{NH}}_{2}\right){\text{CO}}_{2}\text{H}+{\text{HNO}}_{2}\phantom{\rule{0.2em}{0ex}}⟶\phantom{\rule{0.2em}{0ex}}{\text{CH}}_{2}\left(\text{OH}\right){\text{CO}}_{2}\text{H}+{\text{H}}_{2}\text{O}+{\text{N}}_{2}\)
Footnotes
- 1“Quotations by Joseph-Louis Lagrange,” last modified February 2006, accessed February 10, 2015, http://www-history.mcs.st-andrews.ac.uk/Quotations/Lagrange.html
Glossary
- Dalton’s law of partial pressures
- total pressure of a mixture of ideal gases is equal to the sum of the partial pressures of the component gases.
- mole fraction (X)
- concentration unit defined as the ratio of the molar amount of a mixture component to the total number of moles of all mixture components
- partial pressure
- pressure exerted by an individual gas in a mixture
- vapor pressure of water
- pressure exerted by water vapor in equilibrium with liquid water in a closed container at a specific temperature
[latexpage]
Learning Objectives
By the end of this section, you will be able to:
- Describe the formation of covalent bonds
- Define electronegativity and assess the polarity of covalent bonds
Ionic bonding results from the electrostatic attraction of oppositely charged ions that are typically produced by the transfer of electrons between metallic and nonmetallic atoms. A different type of bonding results from the mutual attraction of atoms for a “shared” pair of electrons. Such bonds are called covalent bonds. Covalent bonds are formed between two atoms when both have similar tendencies to attract electrons to themselves (i.e., when both atoms have identical or fairly similar ionization energies and electron affinities). For example, two hydrogen atoms bond covalently to form an H2 molecule; each hydrogen atom in the H2 molecule has two electrons stabilizing it, giving each atom the same number of valence electrons as the noble gas He.
Compounds that contain covalent bonds exhibit different physical properties than ionic compounds. Because the attraction between molecules, which are electrically neutral, is weaker than that between electrically charged ions, covalent compounds generally have much lower melting and boiling points than ionic compounds. In fact, many covalent compounds are liquids or gases at room temperature, and, in their solid states, they are typically much softer than ionic solids. Furthermore, whereas ionic compounds are good conductors of electricity when dissolved in water, most covalent compounds are insoluble in water; since they are electrically neutral, they are poor conductors of electricity in any state.
Formation of Covalent Bonds
Nonmetal atoms frequently form covalent bonds with other nonmetal atoms. For example, the hydrogen molecule, H2, contains a covalent bond between its two hydrogen atoms. (Figure) illustrates why this bond is formed. Starting on the far right, we have two separate hydrogen atoms with a particular potential energy, indicated by the red line. Along the x-axis is the distance between the two atoms. As the two atoms approach each other (moving left along the x-axis), their valence orbitals (1s) begin to overlap. The single electrons on each hydrogen atom then interact with both atomic nuclei, occupying the space around both atoms. The strong attraction of each shared electron to both nuclei stabilizes the system, and the potential energy decreases as the bond distance decreases. If the atoms continue to approach each other, the positive charges in the two nuclei begin to repel each other, and the potential energy increases. The bond length is determined by the distance at which the lowest potential energy is achieved.
It is essential to remember that energy must be added to break chemical bonds (an endothermic process), whereas forming chemical bonds releases energy (an exothermic process). In the case of H2, the covalent bond is very strong; a large amount of energy, 436 kJ, must be added to break the bonds in one mole of hydrogen molecules and cause the atoms to separate:
Conversely, the same amount of energy is released when one mole of H2 molecules forms from two moles of H atoms:
Pure vs. Polar Covalent Bonds
If the atoms that form a covalent bond are identical, as in H2, Cl2, and other diatomic molecules, then the electrons in the bond must be shared equally. We refer to this as a pure covalent bond. Electrons shared in pure covalent bonds have an equal probability of being near each nucleus.
In the case of Cl2, each atom starts off with seven valence electrons, and each Cl shares one electron with the other, forming one covalent bond:
The total number of electrons around each individual atom consists of six nonbonding electrons and two shared (i.e., bonding) electrons for eight total electrons, matching the number of valence electrons in the noble gas argon. Since the bonding atoms are identical, Cl2 also features a pure covalent bond.
When the atoms linked by a covalent bond are different, the bonding electrons are shared, but no longer equally. Instead, the bonding electrons are more attracted to one atom than the other, giving rise to a shift of electron density toward that atom. This unequal distribution of electrons is known as a polar covalent bond, characterized by a partial positive charge on one atom and a partial negative charge on the other. The atom that attracts the electrons more strongly acquires the partial negative charge and vice versa. For example, the electrons in the H–Cl bond of a hydrogen chloride molecule spend more time near the chlorine atom than near the hydrogen atom. Thus, in an HCl molecule, the chlorine atom carries a partial negative charge and the hydrogen atom has a partial positive charge. (Figure) shows the distribution of electrons in the H–Cl bond. Note that the shaded area around Cl is much larger than it is around H. Compare this to (Figure), which shows the even distribution of electrons in the H2 nonpolar bond.
We sometimes designate the positive and negative atoms in a polar covalent bond using a lowercase Greek letter “delta,” δ, with a plus sign or minus sign to indicate whether the atom has a partial positive charge (δ+) or a partial negative charge (δ–). This symbolism is shown for the H–Cl molecule in (Figure).
Electronegativity
Whether a bond is nonpolar or polar covalent is determined by a property of the bonding atoms called electronegativity. Electronegativity is a measure of the tendency of an atom to attract electrons (or electron density) towards itself. It determines how the shared electrons are distributed between the two atoms in a bond. The more strongly an atom attracts the electrons in its bonds, the larger its electronegativity. Electrons in a polar covalent bond are shifted toward the more electronegative atom; thus, the more electronegative atom is the one with the partial negative charge. The greater the difference in electronegativity, the more polarized the electron distribution and the larger the partial charges of the atoms.
(Figure) shows the electronegativity values of the elements as proposed by one of the most famous chemists of the twentieth century: Linus Pauling ((Figure)). In general, electronegativity increases from left to right across a period in the periodic table and decreases down a group. Thus, the nonmetals, which lie in the upper right, tend to have the highest electronegativities, with fluorine the most electronegative element of all (EN = 4.0). Metals tend to be less electronegative elements, and the group 1 metals have the lowest electronegativities. Note that noble gases are excluded from this figure because these atoms usually do not share electrons with others atoms since they have a full valence shell. (While noble gas compounds such as XeO2 do exist, they can only be formed under extreme conditions, and thus they do not fit neatly into the general model of electronegativity.)
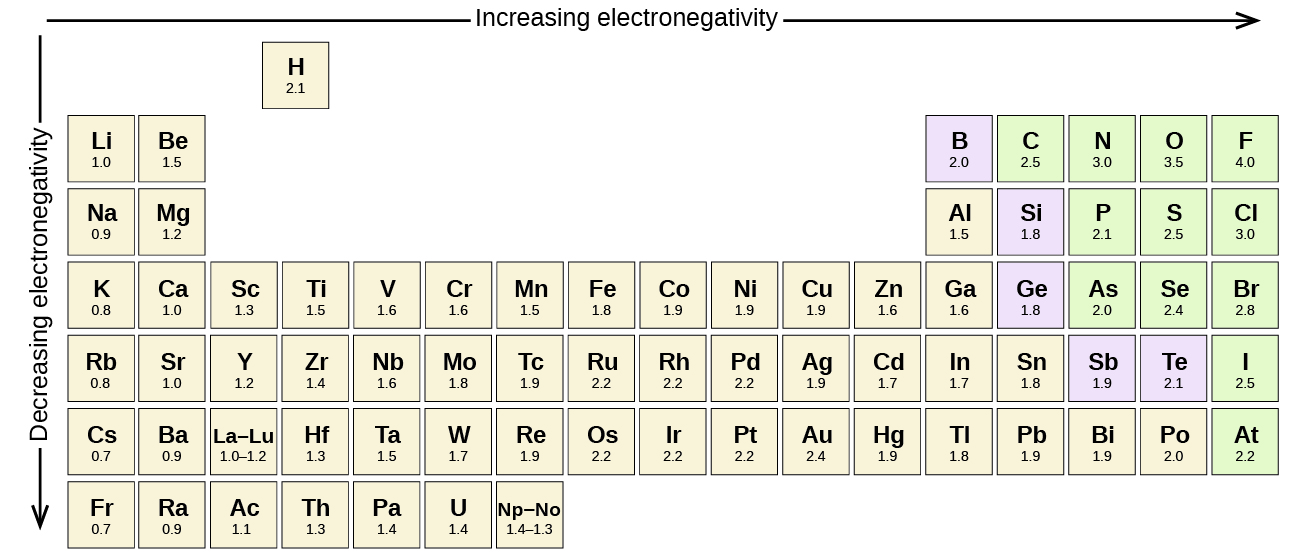
Electronegativity versus Electron Affinity
We must be careful not to confuse electronegativity and electron affinity. The electron affinity of an element is a measurable physical quantity, namely, the energy released or absorbed when an isolated gas-phase atom acquires an electron, measured in kJ/mol. Electronegativity, on the other hand, describes how tightly an atom attracts electrons in a bond. It is a dimensionless quantity that is calculated, not measured. Pauling derived the first electronegativity values by comparing the amounts of energy required to break different types of bonds. He chose an arbitrary relative scale ranging from 0 to 4.
Linus Pauling, shown in (Figure), is the only person to have received two unshared (individual) Nobel Prizes: one for chemistry in 1954 for his work on the nature of chemical bonds and one for peace in 1962 for his opposition to weapons of mass destruction. He developed many of the theories and concepts that are foundational to our current understanding of chemistry, including electronegativity and resonance structures.

Pauling also contributed to many other fields besides chemistry. His research on sickle cell anemia revealed the cause of the disease—the presence of a genetically inherited abnormal protein in the blood—and paved the way for the field of molecular genetics. His work was also pivotal in curbing the testing of nuclear weapons; he proved that radioactive fallout from nuclear testing posed a public health risk.
Electronegativity and Bond Type
The absolute value of the difference in electronegativity (ΔEN) of two bonded atoms provides a rough measure of the polarity to be expected in the bond and, thus, the bond type. When the difference is very small or zero, the bond is covalent and nonpolar. When it is large, the bond is polar covalent or ionic. The absolute values of the electronegativity differences between the atoms in the bonds H–H, H–Cl, and Na–Cl are 0 (nonpolar), 0.9 (polar covalent), and 2.1 (ionic), respectively. The degree to which electrons are shared between atoms varies from completely equal (pure covalent bonding) to not at all (ionic bonding). (Figure) shows the relationship between electronegativity difference and bond type.
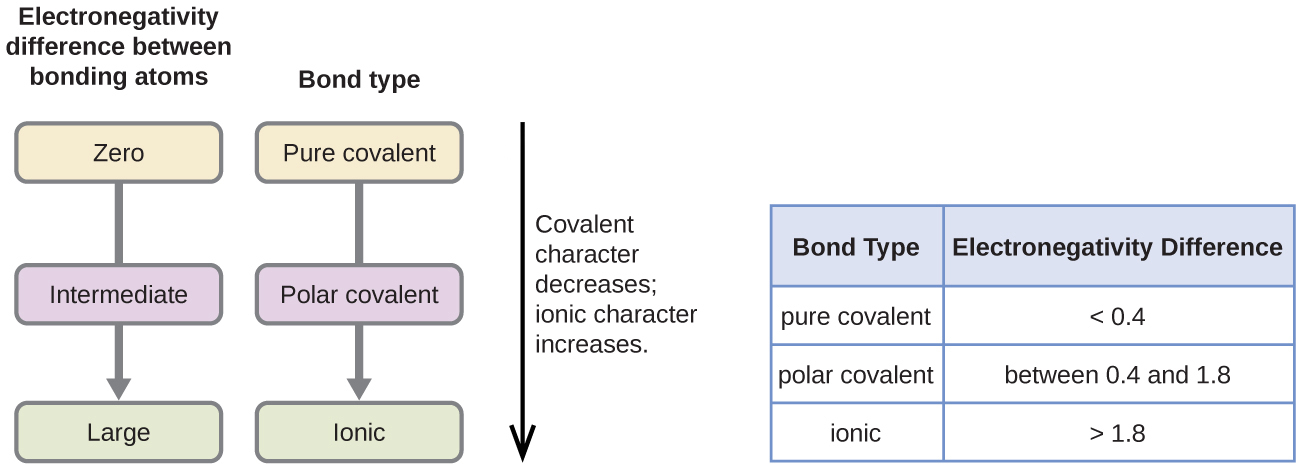
A rough approximation of the electronegativity differences associated with covalent, polar covalent, and ionic bonds is shown in (Figure). This table is just a general guide, however, with many exceptions. For example, the H and F atoms in HF have an electronegativity difference of 1.9, and the N and H atoms in NH3 a difference of 0.9, yet both of these compounds form bonds that are considered polar covalent. Likewise, the Na and Cl atoms in NaCl have an electronegativity difference of 2.1, and the Mn and I atoms in MnI2 have a difference of 1.0, yet both of these substances form ionic compounds.
The best guide to the covalent or ionic character of a bond is to consider the types of atoms involved and their relative positions in the periodic table. Bonds between two nonmetals are generally covalent; bonding between a metal and a nonmetal is often ionic.
Some compounds contain both covalent and ionic bonds. The atoms in polyatomic ions, such as OH–, \({\text{NO}}_{3}{}^{\text{−}},\) and \({\text{NH}}_{4}{}^{\text{+}},\) are held together by polar covalent bonds. However, these polyatomic ions form ionic compounds by combining with ions of opposite charge. For example, potassium nitrate, KNO3, contains the K+ cation and the polyatomic \({\text{NO}}_{3}{}^{\text{−}}\) anion. Thus, bonding in potassium nitrate is ionic, resulting from the electrostatic attraction between the ions K+ and \({\text{NO}}_{3}{}^{\text{−}},\) as well as covalent between the nitrogen and oxygen atoms in \({\text{NO}}_{3}{}^{\text{−}}.\)
Electronegativity and Bond Polarity Bond polarities play an important role in determining the structure of proteins. Using the electronegativity values in (Figure), arrange the following covalent bonds—all commonly found in amino acids—in order of increasing polarity. Then designate the positive and negative atoms using the symbols δ+ and δ–:
C–H, C–N, C–O, N–H, O–H, S–H
Solution The polarity of these bonds increases as the absolute value of the electronegativity difference increases. The atom with the δ– designation is the more electronegative of the two. (Figure) shows these bonds in order of increasing polarity.
| Bond Polarity and Electronegativity Difference | ||
|---|---|---|
| Bond | ΔEN | Polarity |
| C–H | 0.4 | \(\stackrel{\delta \text{−}}{\text{C}}\text{−}\stackrel{\delta \text{+}}{\text{H}}\) |
| S–H | 0.4 | \(\stackrel{\delta \text{−}}{\text{S}}\text{−}\stackrel{\delta \text{+}}{\text{H}}\) |
| C–N | 0.5 | \(\stackrel{\delta \text{+}}{\text{C}}\text{−}\stackrel{\delta \text{−}}{\text{N}}\) |
| N–H | 0.9 | \(\stackrel{\delta \text{−}}{\text{N}}\text{−}\stackrel{\delta \text{+}}{\text{H}}\) |
| C–O | 1.0 | \(\stackrel{\delta \text{+}}{\text{C}}\text{−}\stackrel{\delta \text{−}}{\text{O}}\) |
| O–H | 1.4 | \(\stackrel{\delta \text{−}}{\text{O}}\text{−}\stackrel{\delta \text{+}}{\text{H}}\) |
Check Your Learning Silicones are polymeric compounds containing, among others, the following types of covalent bonds: Si–O, Si–C, C–H, and C–C. Using the electronegativity values in (Figure), arrange the bonds in order of increasing polarity and designate the positive and negative atoms using the symbols δ+ and δ–.
| Bond | Electronegativity Difference | Polarity |
|---|---|---|
| C–C | 0.0 | nonpolar |
| C–H | 0.4 | \(\stackrel{\delta \text{−}}{\text{C}}\text{−}\stackrel{\delta \text{+}}{\text{H}}\) |
| Si–C | 0.7 | \(\stackrel{\delta \text{+}}{\text{Si}}\text{−}\stackrel{\delta \text{−}}{\text{C}}\) |
| Si–O | 1.7 | \(\stackrel{\delta \text{+}}{\text{Si}}\text{−}\stackrel{\delta \text{−}}{\text{O}}\) |
Key Concepts and Summary
Covalent bonds form when electrons are shared between atoms and are attracted by the nuclei of both atoms. In pure covalent bonds, the electrons are shared equally. In polar covalent bonds, the electrons are shared unequally, as one atom exerts a stronger force of attraction on the electrons than the other. The ability of an atom to attract a pair of electrons in a chemical bond is called its electronegativity. The difference in electronegativity between two atoms determines how polar a bond will be. In a diatomic molecule with two identical atoms, there is no difference in electronegativity, so the bond is nonpolar or pure covalent. When the electronegativity difference is very large, as is the case between metals and nonmetals, the bonding is characterized as ionic.
Chemistry End of Chapter Exercises
Why is it incorrect to speak of a molecule of solid NaCl?
NaCl consists of discrete ions arranged in a crystal lattice, not covalently bonded molecules.
What information can you use to predict whether a bond between two atoms is covalent or ionic?
Predict which of the following compounds are ionic and which are covalent, based on the location of their constituent atoms in the periodic table:
(a) Cl2CO
(b) MnO
(c) NCl3
(d) CoBr2
(e) K2S
(f) CO
(g) CaF2
(h) HI
(i) CaO
(j) IBr
(k) CO2
ionic: (b), (d), (e), (g), and (i); covalent: (a), (c), (f), (h), (j), and (k)
Explain the difference between a nonpolar covalent bond, a polar covalent bond, and an ionic bond.
From its position in the periodic table, determine which atom in each pair is more electronegative:
(a) Br or Cl
(b) N or O
(c) S or O
(d) P or S
(e) Si or N
(f) Ba or P
(g) N or K
(a) Cl; (b) O; (c) O; (d) S; (e) N; (f) P; (g) N
From its position in the periodic table, determine which atom in each pair is more electronegative:
(a) N or P
(b) N or Ge
(c) S or F
(d) Cl or S
(e) H or C
(f) Se or P
(g) C or Si
From their positions in the periodic table, arrange the atoms in each of the following series in order of increasing electronegativity:
(a) C, F, H, N, O
(b) Br, Cl, F, H, I
(c) F, H, O, P, S
(d) Al, H, Na, O, P
(e) Ba, H, N, O, As
(a) H, C, N, O, F; (b) H, I, Br, Cl, F; (c) H, P, S, O, F; (d) Na, Al, H, P, O; (e) Ba, H, As, N, O
From their positions in the periodic table, arrange the atoms in each of the following series in order of increasing electronegativity:
(a) As, H, N, P, Sb
(b) Cl, H, P, S, Si
(c) Br, Cl, Ge, H, Sr
(d) Ca, H, K, N, Si
(e) Cl, Cs, Ge, H, Sr
Which atoms can bond to sulfur so as to produce a positive partial charge on the sulfur atom?
N, O, F, and Cl
Which is the most polar bond?
(a) C–C
(b) C–H
(c) N–H
(d) O–H
(e) Se–H
Identify the more polar bond in each of the following pairs of bonds:
(a) HF or HCl
(b) NO or CO
(c) SH or OH
(d) PCl or SCl
(e) CH or NH
(f) SO or PO
(g) CN or NN
(a) HF; (b) CO; (c) OH; (d) PCl; (e) NH; (f) PO; (g) CN
Which of the following molecules or ions contain polar bonds?
(a) O3
(b) S8
(c) \({\text{O}}_{2}{}^{\text{2−}}\)
(d) \({\text{NO}}_{3}{}^{\text{−}}\)
(e) CO2
(f) H2S
(g) \({\text{BH}}_{4}{}^{\text{−}}\)
Glossary
- bond length
- distance between the nuclei of two bonded atoms at which the lowest potential energy is achieved
- covalent bond
- bond formed when electrons are shared between atoms
- electronegativity
- tendency of an atom to attract electrons in a bond to itself
- polar covalent bond
- covalent bond between atoms of different electronegativities; a covalent bond with a positive end and a negative end
- pure covalent bond
- (also, nonpolar covalent bond) covalent bond between atoms of identical electronegativities
[latexpage]
Learning Objectives
By the end of this section, you will be able to:
- Define and explain effusion and diffusion
- State Graham’s law and use it to compute relevant gas properties
If you have ever been in a room when a piping hot pizza was delivered, you have been made aware of the fact that gaseous molecules can quickly spread throughout a room, as evidenced by the pleasant aroma that soon reaches your nose. Although gaseous molecules travel at tremendous speeds (hundreds of meters per second), they collide with other gaseous molecules and travel in many different directions before reaching the desired target. At room temperature, a gaseous molecule will experience billions of collisions per second. The mean free path is the average distance a molecule travels between collisions. The mean free path increases with decreasing pressure; in general, the mean free path for a gaseous molecule will be hundreds of times the diameter of the molecule
In general, we know that when a sample of gas is introduced to one part of a closed container, its molecules very quickly disperse throughout the container; this process by which molecules disperse in space in response to differences in concentration is called diffusion (shown in (Figure)). The gaseous atoms or molecules are, of course, unaware of any concentration gradient, they simply move randomly—regions of higher concentration have more particles than regions of lower concentrations, and so a net movement of species from high to low concentration areas takes place. In a closed environment, diffusion will ultimately result in equal concentrations of gas throughout, as depicted in (Figure). The gaseous atoms and molecules continue to move, but since their concentrations are the same in both bulbs, the rates of transfer between the bulbs are equal (no net transfer of molecules occurs).
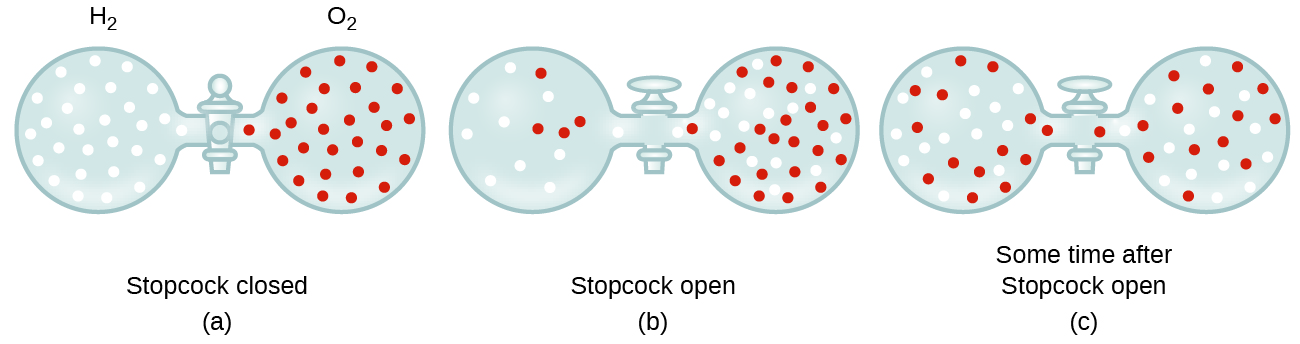
We are often interested in the rate of diffusion, the amount of gas passing through some area per unit time:
The diffusion rate depends on several factors: the concentration gradient (the increase or decrease in concentration from one point to another); the amount of surface area available for diffusion; and the distance the gas particles must travel. Note also that the time required for diffusion to occur is inversely proportional to the rate of diffusion, as shown in the rate of diffusion equation.
A process involving movement of gaseous species similar to diffusion is effusion, the escape of gas molecules through a tiny hole such as a pinhole in a balloon into a vacuum ((Figure)). Although diffusion and effusion rates both depend on the molar mass of the gas involved, their rates are not equal; however, the ratios of their rates are the same.
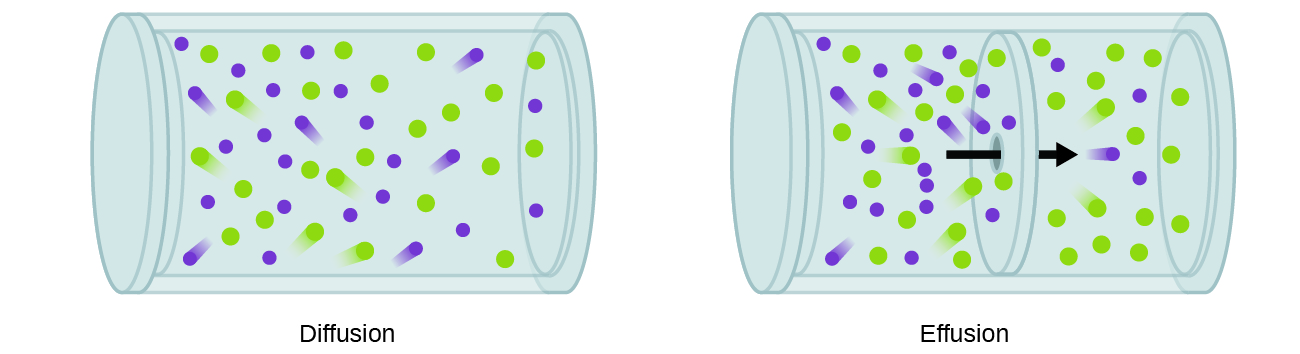
If a mixture of gases is placed in a container with porous walls, the gases effuse through the small openings in the walls. The lighter gases pass through the small openings more rapidly (at a higher rate) than the heavier ones ((Figure)). In 1832, Thomas Graham studied the rates of effusion of different gases and formulated Graham’s law of effusion: The rate of effusion of a gas is inversely proportional to the square root of the mass of its particles:
This means that if two gases A and B are at the same temperature and pressure, the ratio of their effusion rates is inversely proportional to the ratio of the square roots of the masses of their particles:

Applying Graham’s Law to Rates of Effusion Calculate the ratio of the rate of effusion of hydrogen to the rate of effusion of oxygen.
Solution From Graham’s law, we have:
Hydrogen effuses four times as rapidly as oxygen.
Check Your Learning At a particular pressure and temperature, nitrogen gas effuses at the rate of 79 mL/s. Under the same conditions, at what rate will sulfur dioxide effuse?
52 mL/s
Effusion Time Calculations It takes 243 s for 4.46 \(×\) 10−5 mol Xe to effuse through a tiny hole. Under the same conditions, how long will it take 4.46 \(×\) 10−5 mol Ne to effuse?
Solution It is important to resist the temptation to use the times directly, and to remember how rate relates to time as well as how it relates to mass. Recall the definition of rate of effusion:
and combine it with Graham’s law:
To get:
Noting that amount of A = amount of B, and solving for time for Ne:
and substitute values:
Finally, solve for the desired quantity:
Note that this answer is reasonable: Since Ne is lighter than Xe, the effusion rate for Ne will be larger than that for Xe, which means the time of effusion for Ne will be smaller than that for Xe.
Check Your Learning A party balloon filled with helium deflates to \(\frac{2}{3}\) of its original volume in 8.0 hours. How long will it take an identical balloon filled with the same number of moles of air (ℳ = 28.2 g/mol) to deflate to \(\frac{1}{2}\) of its original volume?
32 h
Determining Molar Mass Using Graham’s Law An unknown gas effuses 1.66 times more rapidly than CO2. What is the molar mass of the unknown gas? Can you make a reasonable guess as to its identity?
Solution From Graham’s law, we have:
Plug in known data:
Solve:
The gas could well be CH4, the only gas with this molar mass.
Check Your Learning Hydrogen gas effuses through a porous container 8.97-times faster than an unknown gas. Estimate the molar mass of the unknown gas.
163 g/mol
Gaseous diffusion has been used to produce enriched uranium for use in nuclear power plants and weapons. Naturally occurring uranium contains only 0.72% of 235U, the kind of uranium that is “fissile,” that is, capable of sustaining a nuclear fission chain reaction. Nuclear reactors require fuel that is 2–5% 235U, and nuclear bombs need even higher concentrations. One way to enrich uranium to the desired levels is to take advantage of Graham’s law. In a gaseous diffusion enrichment plant, uranium hexafluoride (UF6, the only uranium compound that is volatile enough to work) is slowly pumped through large cylindrical vessels called diffusers, which contain porous barriers with microscopic openings. The process is one of diffusion because the other side of the barrier is not evacuated. The 235UF6 molecules have a higher average speed and diffuse through the barrier a little faster than the heavier 238UF6 molecules. The gas that has passed through the barrier is slightly enriched in 235UF6 and the residual gas is slightly depleted. The small difference in molecular weights between 235UF6 and 238UF6 only about 0.4% enrichment, is achieved in one diffuser ((Figure)). But by connecting many diffusers in a sequence of stages (called a cascade), the desired level of enrichment can be attained.
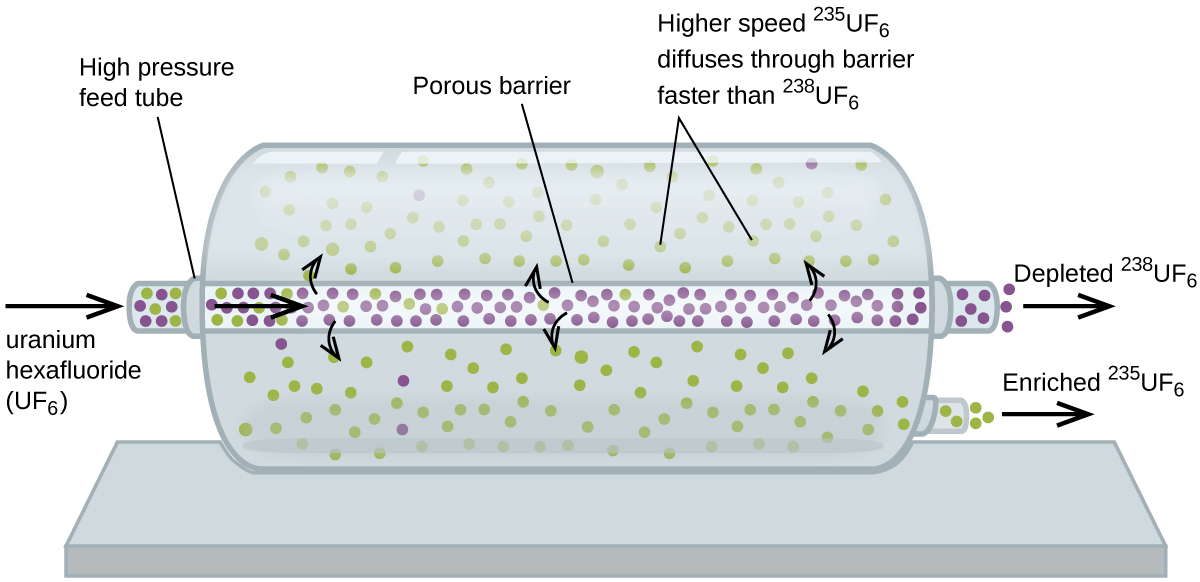
The large scale separation of gaseous 235UF6 from 238UF6 was first done during the World War II, at the atomic energy installation in Oak Ridge, Tennessee, as part of the Manhattan Project (the development of the first atomic bomb). Although the theory is simple, this required surmounting many daunting technical challenges to make it work in practice. The barrier must have tiny, uniform holes (about 10–6 cm in diameter) and be porous enough to produce high flow rates. All materials (the barrier, tubing, surface coatings, lubricants, and gaskets) need to be able to contain, but not react with, the highly reactive and corrosive UF6.
Because gaseous diffusion plants require very large amounts of energy (to compress the gas to the high pressures required and drive it through the diffuser cascade, to remove the heat produced during compression, and so on), it is now being replaced by gas centrifuge technology, which requires far less energy. A current hot political issue is how to deny this technology to Iran, to prevent it from producing enough enriched uranium for them to use to make nuclear weapons.
Key Concepts and Summary
Gaseous atoms and molecules move freely and randomly through space. Diffusion is the process whereby gaseous atoms and molecules are transferred from regions of relatively high concentration to regions of relatively low concentration. Effusion is a similar process in which gaseous species pass from a container to a vacuum through very small orifices. The rates of effusion of gases are inversely proportional to the square roots of their densities or to the square roots of their atoms/molecules’ masses (Graham’s law).
Key Equations
- \(\text{rate of diffusion}=\phantom{\rule{0.2em}{0ex}}\frac{\text{amount of gas passing through an area}}{\text{unit of time}}\)
- \(\frac{\text{rate of effusion of gas A}}{\text{rate of effusion of gas B}}\phantom{\rule{0.2em}{0ex}}=\phantom{\rule{0.2em}{0ex}}\frac{\sqrt{{m}_{B}}}{\sqrt{{m}_{A}}}\phantom{\rule{0.2em}{0ex}}=\phantom{\rule{0.2em}{0ex}}\frac{\sqrt{{\text{ℳ}}_{B}}}{\sqrt{{\text{ℳ}}_{A}}}\)
Chemistry End of Chapter Exercises
A balloon filled with helium gas takes 6 hours to deflate to 50% of its original volume. How long will it take for an identical balloon filled with the same volume of hydrogen gas (instead of helium) to decrease its volume by 50%?
4.2 hours
Explain why the numbers of molecules are not identical in the left- and right-hand bulbs shown in the center illustration of (Figure).
Starting with the definition of rate of effusion and Graham’s finding relating rate and molar mass, show how to derive the Graham’s law equation, relating the relative rates of effusion for two gases to their molecular masses.
Effusion can be defined as the process by which a gas escapes through a pinhole into a vacuum. Graham’s law states that with a mixture of two gases A and B: \(\left(\frac{\text{rate A}}{\text{rate B}}\right)={\left(\frac{\text{molar mass of B}}{\text{molar mass of A}}\right)}^{1\text{/}2}.\) Both A and B are in the same container at the same temperature, and therefore will have the same kinetic energy:
\({\text{KE}}_{\text{A}}={\text{KE}}_{\text{B}}\text{KE}=\phantom{\rule{0.2em}{0ex}}\frac{1}{2}\phantom{\rule{0.2em}{0ex}}m{v}^{2}\)
Therefore, \(\frac{1}{2}{m}_{\text{A}}{v}_{\text{A}}^{2}=\frac{1}{2}{m}_{\text{B}}{v}_{\text{B}}^{2}\)
\(\frac{{v}_{\text{A}}^{2}}{{v}_{\text{B}}^{2}}\phantom{\rule{0.2em}{0ex}}=\phantom{\rule{0.2em}{0ex}}\frac{{m}_{\text{B}}}{{m}_{\text{A}}}\)
\({\left(\frac{{v}_{\text{A}}^{2}}{{v}_{\text{B}}^{2}}\right)}^{1\text{/}2}={\left(\frac{{m}_{\text{B}}}{{m}_{\text{A}}}\right)}^{1\text{/}2}\)
\(\frac{{v}_{\text{A}}}{{v}_{\text{B}}}\phantom{\rule{0.2em}{0ex}}={\left(\frac{{m}_{\text{B}}}{{m}_{\text{A}}}\right)}^{1\text{/}2}\)
Heavy water, D2O (molar mass = 20.03 g mol–1), can be separated from ordinary water, H2O (molar mass = 18.01), as a result of the difference in the relative rates of diffusion of the molecules in the gas phase. Calculate the relative rates of diffusion of H2O and D2O.
Which of the following gases diffuse more slowly than oxygen? F2, Ne, N2O, C2H2, NO, Cl2, H2S
F2, N2O, Cl2, H2S
During the discussion of gaseous diffusion for enriching uranium, it was claimed that 235UF6 diffuses 0.4% faster than 238UF6. Show the calculation that supports this value. The molar mass of 235UF6 = 235.043930 + 6 \(×\) 18.998403 = 349.034348 g/mol, and the molar mass of 238UF6 = 238.050788 + 6 \(×\) 18.998403 = 352.041206 g/mol.
Calculate the relative rate of diffusion of 1H2 (molar mass 2.0 g/mol) compared with 2H2 (molar mass 4.0 g/mol) and the relative rate of diffusion of O2 (molar mass 32 g/mol) compared with O3 (molar mass 48 g/mol).
1.4; 1.2
A gas of unknown identity diffuses at a rate of 83.3 mL/s in a diffusion apparatus in which carbon dioxide diffuses at the rate of 102 mL/s. Calculate the molecular mass of the unknown gas.
When two cotton plugs, one moistened with ammonia and the other with hydrochloric acid, are simultaneously inserted into opposite ends of a glass tube that is 87.0 cm long, a white ring of NH4Cl forms where gaseous NH3 and gaseous HCl first come into contact. \({\text{NH}}_{3}\left(g\right)+\text{HCl}\left(g\right)\phantom{\rule{0.2em}{0ex}}⟶\phantom{\rule{0.2em}{0ex}}{\text{NH}}_{4}\text{Cl}\left(s\right)\) At approximately what distance from the ammonia moistened plug does this occur? (Hint: Calculate the rates of diffusion for both NH3 and HCl, and find out how much faster NH3 diffuses than HCl.)
51.7 cm
Glossary
- diffusion
- movement of an atom or molecule from a region of relatively high concentration to one of relatively low concentration (discussed in this chapter with regard to gaseous species, but applicable to species in any phase)
- effusion
- transfer of gaseous atoms or molecules from a container to a vacuum through very small openings
- Graham’s law of effusion
- rates of diffusion and effusion of gases are inversely proportional to the square roots of their molecular masses
- mean free path
- average distance a molecule travels between collisions
- rate of diffusion
- amount of gas diffusing through a given area over a given time
[latexpage]
Learning Objectives
By the end of this section, you will be able to:
- Define and explain effusion and diffusion
- State Graham’s law and use it to compute relevant gas properties
If you have ever been in a room when a piping hot pizza was delivered, you have been made aware of the fact that gaseous molecules can quickly spread throughout a room, as evidenced by the pleasant aroma that soon reaches your nose. Although gaseous molecules travel at tremendous speeds (hundreds of meters per second), they collide with other gaseous molecules and travel in many different directions before reaching the desired target. At room temperature, a gaseous molecule will experience billions of collisions per second. The mean free path is the average distance a molecule travels between collisions. The mean free path increases with decreasing pressure; in general, the mean free path for a gaseous molecule will be hundreds of times the diameter of the molecule
In general, we know that when a sample of gas is introduced to one part of a closed container, its molecules very quickly disperse throughout the container; this process by which molecules disperse in space in response to differences in concentration is called diffusion (shown in (Figure)). The gaseous atoms or molecules are, of course, unaware of any concentration gradient, they simply move randomly—regions of higher concentration have more particles than regions of lower concentrations, and so a net movement of species from high to low concentration areas takes place. In a closed environment, diffusion will ultimately result in equal concentrations of gas throughout, as depicted in (Figure). The gaseous atoms and molecules continue to move, but since their concentrations are the same in both bulbs, the rates of transfer between the bulbs are equal (no net transfer of molecules occurs).

We are often interested in the rate of diffusion, the amount of gas passing through some area per unit time:
The diffusion rate depends on several factors: the concentration gradient (the increase or decrease in concentration from one point to another); the amount of surface area available for diffusion; and the distance the gas particles must travel. Note also that the time required for diffusion to occur is inversely proportional to the rate of diffusion, as shown in the rate of diffusion equation.
A process involving movement of gaseous species similar to diffusion is effusion, the escape of gas molecules through a tiny hole such as a pinhole in a balloon into a vacuum ((Figure)). Although diffusion and effusion rates both depend on the molar mass of the gas involved, their rates are not equal; however, the ratios of their rates are the same.

If a mixture of gases is placed in a container with porous walls, the gases effuse through the small openings in the walls. The lighter gases pass through the small openings more rapidly (at a higher rate) than the heavier ones ((Figure)). In 1832, Thomas Graham studied the rates of effusion of different gases and formulated Graham’s law of effusion: The rate of effusion of a gas is inversely proportional to the square root of the mass of its particles:
This means that if two gases A and B are at the same temperature and pressure, the ratio of their effusion rates is inversely proportional to the ratio of the square roots of the masses of their particles:

Applying Graham’s Law to Rates of Effusion Calculate the ratio of the rate of effusion of hydrogen to the rate of effusion of oxygen.
Solution From Graham’s law, we have:
Hydrogen effuses four times as rapidly as oxygen.
Check Your Learning At a particular pressure and temperature, nitrogen gas effuses at the rate of 79 mL/s. Under the same conditions, at what rate will sulfur dioxide effuse?
52 mL/s
Effusion Time Calculations It takes 243 s for 4.46 \(×\) 10−5 mol Xe to effuse through a tiny hole. Under the same conditions, how long will it take 4.46 \(×\) 10−5 mol Ne to effuse?
Solution It is important to resist the temptation to use the times directly, and to remember how rate relates to time as well as how it relates to mass. Recall the definition of rate of effusion:
and combine it with Graham’s law:
To get:
Noting that amount of A = amount of B, and solving for time for Ne:
and substitute values:
Finally, solve for the desired quantity:
Note that this answer is reasonable: Since Ne is lighter than Xe, the effusion rate for Ne will be larger than that for Xe, which means the time of effusion for Ne will be smaller than that for Xe.
Check Your Learning A party balloon filled with helium deflates to \(\frac{2}{3}\) of its original volume in 8.0 hours. How long will it take an identical balloon filled with the same number of moles of air (ℳ = 28.2 g/mol) to deflate to \(\frac{1}{2}\) of its original volume?
32 h
Determining Molar Mass Using Graham’s Law An unknown gas effuses 1.66 times more rapidly than CO2. What is the molar mass of the unknown gas? Can you make a reasonable guess as to its identity?
Solution From Graham’s law, we have:
Plug in known data:
Solve:
The gas could well be CH4, the only gas with this molar mass.
Check Your Learning Hydrogen gas effuses through a porous container 8.97-times faster than an unknown gas. Estimate the molar mass of the unknown gas.
163 g/mol
Gaseous diffusion has been used to produce enriched uranium for use in nuclear power plants and weapons. Naturally occurring uranium contains only 0.72% of 235U, the kind of uranium that is “fissile,” that is, capable of sustaining a nuclear fission chain reaction. Nuclear reactors require fuel that is 2–5% 235U, and nuclear bombs need even higher concentrations. One way to enrich uranium to the desired levels is to take advantage of Graham’s law. In a gaseous diffusion enrichment plant, uranium hexafluoride (UF6, the only uranium compound that is volatile enough to work) is slowly pumped through large cylindrical vessels called diffusers, which contain porous barriers with microscopic openings. The process is one of diffusion because the other side of the barrier is not evacuated. The 235UF6 molecules have a higher average speed and diffuse through the barrier a little faster than the heavier 238UF6 molecules. The gas that has passed through the barrier is slightly enriched in 235UF6 and the residual gas is slightly depleted. The small difference in molecular weights between 235UF6 and 238UF6 only about 0.4% enrichment, is achieved in one diffuser ((Figure)). But by connecting many diffusers in a sequence of stages (called a cascade), the desired level of enrichment can be attained.

The large scale separation of gaseous 235UF6 from 238UF6 was first done during the World War II, at the atomic energy installation in Oak Ridge, Tennessee, as part of the Manhattan Project (the development of the first atomic bomb). Although the theory is simple, this required surmounting many daunting technical challenges to make it work in practice. The barrier must have tiny, uniform holes (about 10–6 cm in diameter) and be porous enough to produce high flow rates. All materials (the barrier, tubing, surface coatings, lubricants, and gaskets) need to be able to contain, but not react with, the highly reactive and corrosive UF6.
Because gaseous diffusion plants require very large amounts of energy (to compress the gas to the high pressures required and drive it through the diffuser cascade, to remove the heat produced during compression, and so on), it is now being replaced by gas centrifuge technology, which requires far less energy. A current hot political issue is how to deny this technology to Iran, to prevent it from producing enough enriched uranium for them to use to make nuclear weapons.
Key Concepts and Summary
Gaseous atoms and molecules move freely and randomly through space. Diffusion is the process whereby gaseous atoms and molecules are transferred from regions of relatively high concentration to regions of relatively low concentration. Effusion is a similar process in which gaseous species pass from a container to a vacuum through very small orifices. The rates of effusion of gases are inversely proportional to the square roots of their densities or to the square roots of their atoms/molecules’ masses (Graham’s law).
Key Equations
- \(\text{rate of diffusion}=\phantom{\rule{0.2em}{0ex}}\frac{\text{amount of gas passing through an area}}{\text{unit of time}}\)
- \(\frac{\text{rate of effusion of gas A}}{\text{rate of effusion of gas B}}\phantom{\rule{0.2em}{0ex}}=\phantom{\rule{0.2em}{0ex}}\frac{\sqrt{{m}_{B}}}{\sqrt{{m}_{A}}}\phantom{\rule{0.2em}{0ex}}=\phantom{\rule{0.2em}{0ex}}\frac{\sqrt{{\text{ℳ}}_{B}}}{\sqrt{{\text{ℳ}}_{A}}}\)
Chemistry End of Chapter Exercises
A balloon filled with helium gas takes 6 hours to deflate to 50% of its original volume. How long will it take for an identical balloon filled with the same volume of hydrogen gas (instead of helium) to decrease its volume by 50%?
4.2 hours
Explain why the numbers of molecules are not identical in the left- and right-hand bulbs shown in the center illustration of (Figure).
Starting with the definition of rate of effusion and Graham’s finding relating rate and molar mass, show how to derive the Graham’s law equation, relating the relative rates of effusion for two gases to their molecular masses.
Effusion can be defined as the process by which a gas escapes through a pinhole into a vacuum. Graham’s law states that with a mixture of two gases A and B: \(\left(\frac{\text{rate A}}{\text{rate B}}\right)={\left(\frac{\text{molar mass of B}}{\text{molar mass of A}}\right)}^{1\text{/}2}.\) Both A and B are in the same container at the same temperature, and therefore will have the same kinetic energy:
\({\text{KE}}_{\text{A}}={\text{KE}}_{\text{B}}\text{KE}=\phantom{\rule{0.2em}{0ex}}\frac{1}{2}\phantom{\rule{0.2em}{0ex}}m{v}^{2}\)
Therefore, \(\frac{1}{2}{m}_{\text{A}}{v}_{\text{A}}^{2}=\frac{1}{2}{m}_{\text{B}}{v}_{\text{B}}^{2}\)
\(\frac{{v}_{\text{A}}^{2}}{{v}_{\text{B}}^{2}}\phantom{\rule{0.2em}{0ex}}=\phantom{\rule{0.2em}{0ex}}\frac{{m}_{\text{B}}}{{m}_{\text{A}}}\)
\({\left(\frac{{v}_{\text{A}}^{2}}{{v}_{\text{B}}^{2}}\right)}^{1\text{/}2}={\left(\frac{{m}_{\text{B}}}{{m}_{\text{A}}}\right)}^{1\text{/}2}\)
\(\frac{{v}_{\text{A}}}{{v}_{\text{B}}}\phantom{\rule{0.2em}{0ex}}={\left(\frac{{m}_{\text{B}}}{{m}_{\text{A}}}\right)}^{1\text{/}2}\)
Heavy water, D2O (molar mass = 20.03 g mol–1), can be separated from ordinary water, H2O (molar mass = 18.01), as a result of the difference in the relative rates of diffusion of the molecules in the gas phase. Calculate the relative rates of diffusion of H2O and D2O.
Which of the following gases diffuse more slowly than oxygen? F2, Ne, N2O, C2H2, NO, Cl2, H2S
F2, N2O, Cl2, H2S
During the discussion of gaseous diffusion for enriching uranium, it was claimed that 235UF6 diffuses 0.4% faster than 238UF6. Show the calculation that supports this value. The molar mass of 235UF6 = 235.043930 + 6 \(×\) 18.998403 = 349.034348 g/mol, and the molar mass of 238UF6 = 238.050788 + 6 \(×\) 18.998403 = 352.041206 g/mol.
Calculate the relative rate of diffusion of 1H2 (molar mass 2.0 g/mol) compared with 2H2 (molar mass 4.0 g/mol) and the relative rate of diffusion of O2 (molar mass 32 g/mol) compared with O3 (molar mass 48 g/mol).
1.4; 1.2
A gas of unknown identity diffuses at a rate of 83.3 mL/s in a diffusion apparatus in which carbon dioxide diffuses at the rate of 102 mL/s. Calculate the molecular mass of the unknown gas.
When two cotton plugs, one moistened with ammonia and the other with hydrochloric acid, are simultaneously inserted into opposite ends of a glass tube that is 87.0 cm long, a white ring of NH4Cl forms where gaseous NH3 and gaseous HCl first come into contact. \({\text{NH}}_{3}\left(g\right)+\text{HCl}\left(g\right)\phantom{\rule{0.2em}{0ex}}⟶\phantom{\rule{0.2em}{0ex}}{\text{NH}}_{4}\text{Cl}\left(s\right)\) At approximately what distance from the ammonia moistened plug does this occur? (Hint: Calculate the rates of diffusion for both NH3 and HCl, and find out how much faster NH3 diffuses than HCl.)
51.7 cm
Glossary
- diffusion
- movement of an atom or molecule from a region of relatively high concentration to one of relatively low concentration (discussed in this chapter with regard to gaseous species, but applicable to species in any phase)
- effusion
- transfer of gaseous atoms or molecules from a container to a vacuum through very small openings
- Graham’s law of effusion
- rates of diffusion and effusion of gases are inversely proportional to the square roots of their molecular masses
- mean free path
- average distance a molecule travels between collisions
- rate of diffusion
- amount of gas diffusing through a given area over a given time
[latexpage]
Learning Objectives
- State the postulates of the kinetic-molecular theory
- Use this theory’s postulates to explain the gas laws
The gas laws that we have seen to this point, as well as the ideal gas equation, are empirical, that is, they have been derived from experimental observations. The mathematical forms of these laws closely describe the macroscopic behavior of most gases at pressures less than about 1 or 2 atm. Although the gas laws describe relationships that have been verified by many experiments, they do not tell us why gases follow these relationships.
The kinetic molecular theory (KMT) is a simple microscopic model that effectively explains the gas laws described in previous modules of this chapter. This theory is based on the following five postulates described here. (Note: The term “molecule” will be used to refer to the individual chemical species that compose the gas, although some gases are composed of atomic species, for example, the noble gases.)
- Gases are composed of molecules that are in continuous motion, travelling in straight lines and changing direction only when they collide with other molecules or with the walls of a container.
- The molecules composing the gas are negligibly small compared to the distances between them.
- The pressure exerted by a gas in a container results from collisions between the gas molecules and the container walls.
- Gas molecules exert no attractive or repulsive forces on each other or the container walls; therefore, their collisions are elastic (do not involve a loss of energy).
- The average kinetic energy of the gas molecules is proportional to the kelvin temperature of the gas.
The test of the KMT and its postulates is its ability to explain and describe the behavior of a gas. The various gas laws can be derived from the assumptions of the KMT, which have led chemists to believe that the assumptions of the theory accurately represent the properties of gas molecules. We will first look at the individual gas laws (Boyle’s, Charles’s, Amontons’s, Avogadro’s, and Dalton’s laws) conceptually to see how the KMT explains them. Then, we will more carefully consider the relationships between molecular masses, speeds, and kinetic energies with temperature, and explain Graham’s law.
The Kinetic-Molecular Theory Explains the Behavior of Gases, Part I
Recalling that gas pressure is exerted by rapidly moving gas molecules and depends directly on the number of molecules hitting a unit area of the wall per unit of time, we see that the KMT conceptually explains the behavior of a gas as follows:
- Amontons’s law. If the temperature is increased, the average speed and kinetic energy of the gas molecules increase. If the volume is held constant, the increased speed of the gas molecules results in more frequent and more forceful collisions with the walls of the container, therefore increasing the pressure ((Figure)).
- Charles’s law. If the temperature of a gas is increased, a constant pressure may be maintained only if the volume occupied by the gas increases. This will result in greater average distances traveled by the molecules to reach the container walls, as well as increased wall surface area. These conditions will decrease the both the frequency of molecule-wall collisions and the number of collisions per unit area, the combined effects of which balance the effect of increased collision forces due to the greater kinetic energy at the higher temperature.
- Boyle’s law. If the gas volume is decreased, the container wall area decreases and the molecule-wall collision frequency increases, both of which increase the pressure exerted by the gas ((Figure)).
- Avogadro’s law. At constant pressure and temperature, the frequency and force of molecule-wall collisions are constant. Under such conditions, increasing the number of gaseous molecules will require a proportional increase in the container volume in order to yield a decrease in the number of collisions per unit area to compensate for the increased frequency of collisions ((Figure)).
- Dalton’s Law. Because of the large distances between them, the molecules of one gas in a mixture bombard the container walls with the same frequency whether other gases are present or not, and the total pressure of a gas mixture equals the sum of the (partial) pressures of the individual gases.
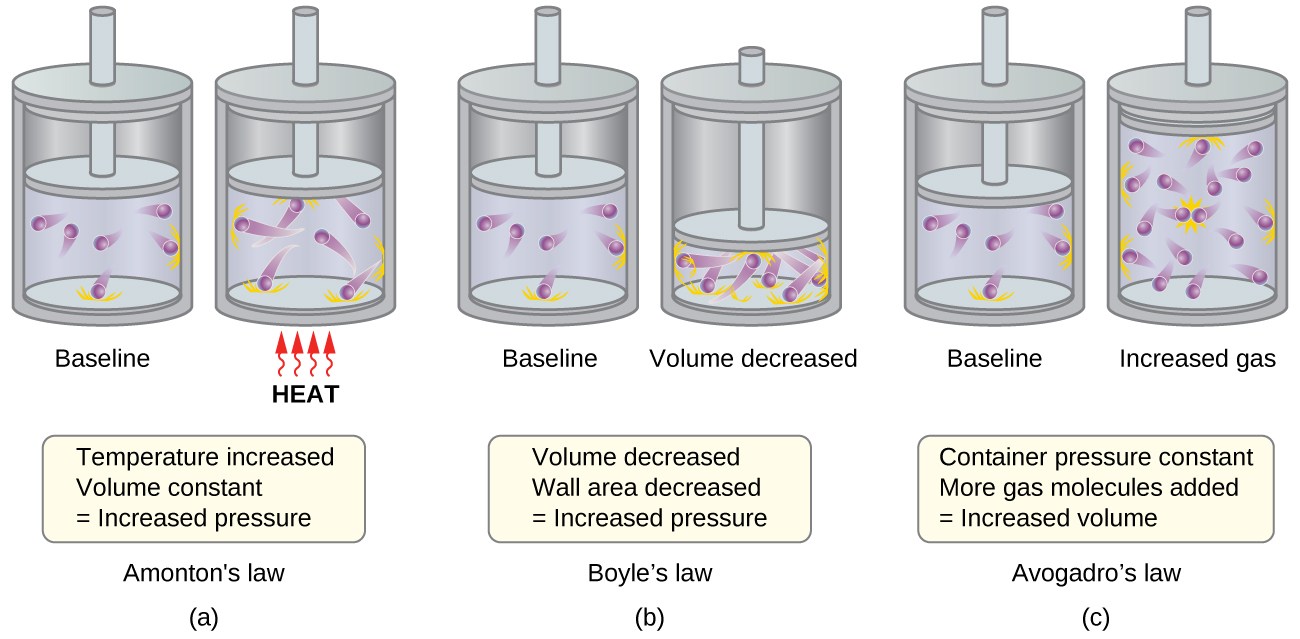
Molecular Velocities and Kinetic Energy
The previous discussion showed that the KMT qualitatively explains the behaviors described by the various gas laws. The postulates of this theory may be applied in a more quantitative fashion to derive these individual laws. To do this, we must first look at velocities and kinetic energies of gas molecules, and the temperature of a gas sample.
In a gas sample, individual molecules have widely varying speeds; however, because of the vast number of molecules and collisions involved, the molecular speed distribution and average speed are constant. This molecular speed distribution is known as a Maxwell-Boltzmann distribution, and it depicts the relative numbers of molecules in a bulk sample of gas that possesses a given speed ((Figure)).
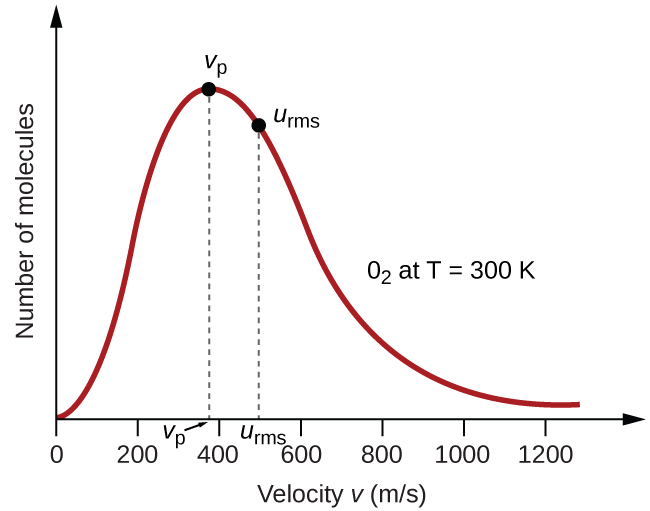
The kinetic energy (KE) of a particle of mass (m) and speed (u) is given by:
Expressing mass in kilograms and speed in meters per second will yield energy values in units of joules (J = kg m2 s–2). To deal with a large number of gas molecules, we use averages for both speed and kinetic energy. In the KMT, the root mean square velocity of a particle, urms, is defined as the square root of the average of the squares of the velocities with n = the number of particles:
The average kinetic energy for a mole of particles, KEavg, is then equal to:
where M is the molar mass expressed in units of kg/mol. The KEavg of a mole of gas molecules is also directly proportional to the temperature of the gas and may be described by the equation:
where R is the gas constant and T is the kelvin temperature. When used in this equation, the appropriate form of the gas constant is 8.314 J/mol⋅K (8.314 kg m2s–2mol–1K–1). These two separate equations for KEavg may be combined and rearranged to yield a relation between molecular speed and temperature:
Calculation of urmsCalculate the root-mean-square velocity for a nitrogen molecule at 30 °C.
Solution Convert the temperature into Kelvin:
Determine the molar mass of nitrogen in kilograms:
Replace the variables and constants in the root-mean-square velocity equation, replacing Joules with the equivalent kg m2s–2:
Check Your LearningCalculate the root-mean-square velocity for a mole of oxygen molecules at –23 °C.
441 m/s
If the temperature of a gas increases, its KEavg increases, more molecules have higher speeds and fewer molecules have lower speeds, and the distribution shifts toward higher speeds overall, that is, to the right. If temperature decreases, KEavg decreases, more molecules have lower speeds and fewer molecules have higher speeds, and the distribution shifts toward lower speeds overall, that is, to the left. This behavior is illustrated for nitrogen gas in (Figure).
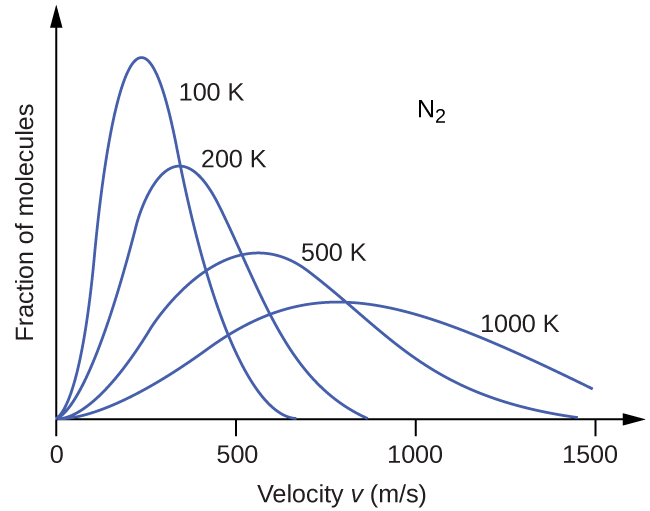
At a given temperature, all gases have the same KEavg for their molecules. Gases composed of lighter molecules have more high-speed particles and a higher urms, with a speed distribution that peaks at relatively higher velocities. Gases consisting of heavier molecules have more low-speed particles, a lower urms, and a speed distribution that peaks at relatively lower velocities. This trend is demonstrated by the data for a series of noble gases shown in (Figure).
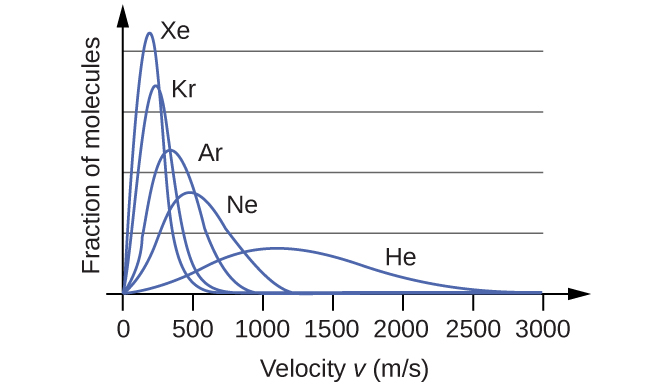
The gas simulator may be used to examine the effect of temperature on molecular velocities. Examine the simulator’s “energy histograms” (molecular speed distributions) and “species information” (which gives average speed values) for molecules of different masses at various temperatures.
The Kinetic-Molecular Theory Explains the Behavior of Gases, Part II
According to Graham’s law, the molecules of a gas are in rapid motion and the molecules themselves are small. The average distance between the molecules of a gas is large compared to the size of the molecules. As a consequence, gas molecules can move past each other easily and diffuse at relatively fast rates.
The rate of effusion of a gas depends directly on the (average) speed of its molecules:
Using this relation, and the equation relating molecular speed to mass, Graham’s law may be easily derived as shown here:
The ratio of the rates of effusion is thus derived to be inversely proportional to the ratio of the square roots of their masses. This is the same relation observed experimentally and expressed as Graham’s law.
Key Concepts and Summary
The kinetic molecular theory is a simple but very effective model that effectively explains ideal gas behavior. The theory assumes that gases consist of widely separated molecules of negligible volume that are in constant motion, colliding elastically with one another and the walls of their container with average velocities determined by their absolute temperatures. The individual molecules of a gas exhibit a range of velocities, the distribution of these velocities being dependent on the temperature of the gas and the mass of its molecules.
Key Equations
- \({u}_{\text{r}\text{m}\text{s}}=\sqrt{\overline{{u}^{2}}}=\sqrt{\frac{{u}_{1}^{2}+{u}_{2}^{2}+{u}_{3}^{2}+{u}_{4}^{2}+\dots }{n}}\)
- \({\text{KE}}_{\text{avg}}=\phantom{\rule{0.2em}{0ex}}\frac{3}{2}RT\)
- \({u}_{\text{rms}}=\sqrt{\frac{3RT}{M}}\)
Chemistry End of Chapter Exercises
Using the postulates of the kinetic molecular theory, explain why a gas uniformly fills a container of any shape.
Can the speed of a given molecule in a gas double at constant temperature? Explain your answer.
Yes. At any given instant, there are a range of values of molecular speeds in a sample of gas. Any single molecule can speed up or slow down as it collides with other molecules. The average velocity of all the molecules is constant at constant temperature.
Describe what happens to the average kinetic energy of ideal gas molecules when the conditions are changed as follows:
(a) The pressure of the gas is increased by reducing the volume at constant temperature.
(b) The pressure of the gas is increased by increasing the temperature at constant volume.
(c) The average velocity of the molecules is increased by a factor of 2.
The distribution of molecular velocities in a sample of helium is shown in (Figure). If the sample is cooled, will the distribution of velocities look more like that of H2 or of H2O? Explain your answer.
H2O. Cooling slows the velocities of the He atoms, causing them to behave as though they were heavier.
What is the ratio of the average kinetic energy of a SO2 molecule to that of an O2 molecule in a mixture of two gases? What is the ratio of the root mean square speeds, urms, of the two gases?
A 1-L sample of CO initially at STP is heated to 546 K, and its volume is increased to 2 L.
(a) What effect do these changes have on the number of collisions of the molecules of the gas per unit area of the container wall?
(b) What is the effect on the average kinetic energy of the molecules?
(c) What is the effect on the root mean square speed of the molecules?
(a) The number of collisions per unit area of the container wall is constant. (b) The average kinetic energy doubles. (c) The root mean square speed increases to \(\sqrt{2}\) times its initial value; urms is proportional to \(\sqrt{{\text{KE}}_{\text{avg}}}.\)
The root mean square speed of H2 molecules at 25 °C is about 1.6 km/s. What is the root mean square speed of a N2 molecule at 25 °C?
Answer the following questions:
(a) Is the pressure of the gas in the hot-air balloon shown at the opening of this chapter greater than, less than, or equal to that of the atmosphere outside the balloon?
(b) Is the density of the gas in the hot-air balloon shown at the opening of this chapter greater than, less than, or equal to that of the atmosphere outside the balloon?
(c) At a pressure of 1 atm and a temperature of 20 °C, dry air has a density of 1.2256 g/L. What is the (average) molar mass of dry air?
(d) The average temperature of the gas in a hot-air balloon is 1.30 \(×\) 102 °F. Calculate its density, assuming the molar mass equals that of dry air.
(e) The lifting capacity of a hot-air balloon is equal to the difference in the mass of the cool air displaced by the balloon and the mass of the gas in the balloon. What is the difference in the mass of 1.00 L of the cool air in part (c) and the hot air in part (d)?
(f) An average balloon has a diameter of 60 feet and a volume of 1.1 \(×\) 105 ft3. What is the lifting power of such a balloon? If the weight of the balloon and its rigging is 500 pounds, what is its capacity for carrying passengers and cargo?
(g) A balloon carries 40.0 gallons of liquid propane (density 0.5005 g/L). What volume of CO2 and H2O gas is produced by the combustion of this propane?
(h) A balloon flight can last about 90 minutes. If all of the fuel is burned during this time, what is the approximate rate of heat loss (in kJ/min) from the hot air in the bag during the flight?
(a) equal; (b) less than; (c) 29.48 g mol−1; (d) 1.0966 g L−1; (e) 0.129 g/L; (f) 4.01 \(×\) 105 g; net lifting capacity = 384 lb; (g) 270 L; (h) 39.1 kJ min−1
Show that the ratio of the rate of diffusion of Gas 1 to the rate of diffusion of Gas 2, \(\frac{{R}_{1}}{{R}_{2}},\) is the same at 0 °C and 100 °C.
Glossary
- kinetic molecular theory
- theory based on simple principles and assumptions that effectively explains ideal gas behavior
- root mean square velocity (urms)
- measure of average velocity for a group of particles calculated as the square root of the average squared velocity
[latexpage]
Learning Objectives
- State the postulates of the kinetic-molecular theory
- Use this theory’s postulates to explain the gas laws
The gas laws that we have seen to this point, as well as the ideal gas equation, are empirical, that is, they have been derived from experimental observations. The mathematical forms of these laws closely describe the macroscopic behavior of most gases at pressures less than about 1 or 2 atm. Although the gas laws describe relationships that have been verified by many experiments, they do not tell us why gases follow these relationships.
The kinetic molecular theory (KMT) is a simple microscopic model that effectively explains the gas laws described in previous modules of this chapter. This theory is based on the following five postulates described here. (Note: The term “molecule” will be used to refer to the individual chemical species that compose the gas, although some gases are composed of atomic species, for example, the noble gases.)
- Gases are composed of molecules that are in continuous motion, travelling in straight lines and changing direction only when they collide with other molecules or with the walls of a container.
- The molecules composing the gas are negligibly small compared to the distances between them.
- The pressure exerted by a gas in a container results from collisions between the gas molecules and the container walls.
- Gas molecules exert no attractive or repulsive forces on each other or the container walls; therefore, their collisions are elastic (do not involve a loss of energy).
- The average kinetic energy of the gas molecules is proportional to the kelvin temperature of the gas.
The test of the KMT and its postulates is its ability to explain and describe the behavior of a gas. The various gas laws can be derived from the assumptions of the KMT, which have led chemists to believe that the assumptions of the theory accurately represent the properties of gas molecules. We will first look at the individual gas laws (Boyle’s, Charles’s, Amontons’s, Avogadro’s, and Dalton’s laws) conceptually to see how the KMT explains them. Then, we will more carefully consider the relationships between molecular masses, speeds, and kinetic energies with temperature, and explain Graham’s law.
The Kinetic-Molecular Theory Explains the Behavior of Gases, Part I
Recalling that gas pressure is exerted by rapidly moving gas molecules and depends directly on the number of molecules hitting a unit area of the wall per unit of time, we see that the KMT conceptually explains the behavior of a gas as follows:
- Amontons’s law. If the temperature is increased, the average speed and kinetic energy of the gas molecules increase. If the volume is held constant, the increased speed of the gas molecules results in more frequent and more forceful collisions with the walls of the container, therefore increasing the pressure ((Figure)).
- Charles’s law. If the temperature of a gas is increased, a constant pressure may be maintained only if the volume occupied by the gas increases. This will result in greater average distances traveled by the molecules to reach the container walls, as well as increased wall surface area. These conditions will decrease the both the frequency of molecule-wall collisions and the number of collisions per unit area, the combined effects of which balance the effect of increased collision forces due to the greater kinetic energy at the higher temperature.
- Boyle’s law. If the gas volume is decreased, the container wall area decreases and the molecule-wall collision frequency increases, both of which increase the pressure exerted by the gas ((Figure)).
- Avogadro’s law. At constant pressure and temperature, the frequency and force of molecule-wall collisions are constant. Under such conditions, increasing the number of gaseous molecules will require a proportional increase in the container volume in order to yield a decrease in the number of collisions per unit area to compensate for the increased frequency of collisions ((Figure)).
- Dalton’s Law. Because of the large distances between them, the molecules of one gas in a mixture bombard the container walls with the same frequency whether other gases are present or not, and the total pressure of a gas mixture equals the sum of the (partial) pressures of the individual gases.

Molecular Velocities and Kinetic Energy
The previous discussion showed that the KMT qualitatively explains the behaviors described by the various gas laws. The postulates of this theory may be applied in a more quantitative fashion to derive these individual laws. To do this, we must first look at velocities and kinetic energies of gas molecules, and the temperature of a gas sample.
In a gas sample, individual molecules have widely varying speeds; however, because of the vast number of molecules and collisions involved, the molecular speed distribution and average speed are constant. This molecular speed distribution is known as a Maxwell-Boltzmann distribution, and it depicts the relative numbers of molecules in a bulk sample of gas that possesses a given speed ((Figure)).

The kinetic energy (KE) of a particle of mass (m) and speed (u) is given by:
Expressing mass in kilograms and speed in meters per second will yield energy values in units of joules (J = kg m2 s–2). To deal with a large number of gas molecules, we use averages for both speed and kinetic energy. In the KMT, the root mean square velocity of a particle, urms, is defined as the square root of the average of the squares of the velocities with n = the number of particles:
The average kinetic energy for a mole of particles, KEavg, is then equal to:
where M is the molar mass expressed in units of kg/mol. The KEavg of a mole of gas molecules is also directly proportional to the temperature of the gas and may be described by the equation:
where R is the gas constant and T is the kelvin temperature. When used in this equation, the appropriate form of the gas constant is 8.314 J/mol⋅K (8.314 kg m2s–2mol–1K–1). These two separate equations for KEavg may be combined and rearranged to yield a relation between molecular speed and temperature:
Calculation of urmsCalculate the root-mean-square velocity for a nitrogen molecule at 30 °C.
Solution Convert the temperature into Kelvin:
Determine the molar mass of nitrogen in kilograms:
Replace the variables and constants in the root-mean-square velocity equation, replacing Joules with the equivalent kg m2s–2:
Check Your LearningCalculate the root-mean-square velocity for a mole of oxygen molecules at –23 °C.
441 m/s
If the temperature of a gas increases, its KEavg increases, more molecules have higher speeds and fewer molecules have lower speeds, and the distribution shifts toward higher speeds overall, that is, to the right. If temperature decreases, KEavg decreases, more molecules have lower speeds and fewer molecules have higher speeds, and the distribution shifts toward lower speeds overall, that is, to the left. This behavior is illustrated for nitrogen gas in (Figure).

At a given temperature, all gases have the same KEavg for their molecules. Gases composed of lighter molecules have more high-speed particles and a higher urms, with a speed distribution that peaks at relatively higher velocities. Gases consisting of heavier molecules have more low-speed particles, a lower urms, and a speed distribution that peaks at relatively lower velocities. This trend is demonstrated by the data for a series of noble gases shown in (Figure).

The gas simulator may be used to examine the effect of temperature on molecular velocities. Examine the simulator’s “energy histograms” (molecular speed distributions) and “species information” (which gives average speed values) for molecules of different masses at various temperatures.
The Kinetic-Molecular Theory Explains the Behavior of Gases, Part II
According to Graham’s law, the molecules of a gas are in rapid motion and the molecules themselves are small. The average distance between the molecules of a gas is large compared to the size of the molecules. As a consequence, gas molecules can move past each other easily and diffuse at relatively fast rates.
The rate of effusion of a gas depends directly on the (average) speed of its molecules:
Using this relation, and the equation relating molecular speed to mass, Graham’s law may be easily derived as shown here:
The ratio of the rates of effusion is thus derived to be inversely proportional to the ratio of the square roots of their masses. This is the same relation observed experimentally and expressed as Graham’s law.
Key Concepts and Summary
The kinetic molecular theory is a simple but very effective model that effectively explains ideal gas behavior. The theory assumes that gases consist of widely separated molecules of negligible volume that are in constant motion, colliding elastically with one another and the walls of their container with average velocities determined by their absolute temperatures. The individual molecules of a gas exhibit a range of velocities, the distribution of these velocities being dependent on the temperature of the gas and the mass of its molecules.
Key Equations
- \({u}_{\text{r}\text{m}\text{s}}=\sqrt{\overline{{u}^{2}}}=\sqrt{\frac{{u}_{1}^{2}+{u}_{2}^{2}+{u}_{3}^{2}+{u}_{4}^{2}+\dots }{n}}\)
- \({\text{KE}}_{\text{avg}}=\phantom{\rule{0.2em}{0ex}}\frac{3}{2}RT\)
- \({u}_{\text{rms}}=\sqrt{\frac{3RT}{M}}\)
Chemistry End of Chapter Exercises
Using the postulates of the kinetic molecular theory, explain why a gas uniformly fills a container of any shape.
Can the speed of a given molecule in a gas double at constant temperature? Explain your answer.
Yes. At any given instant, there are a range of values of molecular speeds in a sample of gas. Any single molecule can speed up or slow down as it collides with other molecules. The average velocity of all the molecules is constant at constant temperature.
Describe what happens to the average kinetic energy of ideal gas molecules when the conditions are changed as follows:
(a) The pressure of the gas is increased by reducing the volume at constant temperature.
(b) The pressure of the gas is increased by increasing the temperature at constant volume.
(c) The average velocity of the molecules is increased by a factor of 2.
The distribution of molecular velocities in a sample of helium is shown in (Figure). If the sample is cooled, will the distribution of velocities look more like that of H2 or of H2O? Explain your answer.
H2O. Cooling slows the velocities of the He atoms, causing them to behave as though they were heavier.
What is the ratio of the average kinetic energy of a SO2 molecule to that of an O2 molecule in a mixture of two gases? What is the ratio of the root mean square speeds, urms, of the two gases?
A 1-L sample of CO initially at STP is heated to 546 K, and its volume is increased to 2 L.
(a) What effect do these changes have on the number of collisions of the molecules of the gas per unit area of the container wall?
(b) What is the effect on the average kinetic energy of the molecules?
(c) What is the effect on the root mean square speed of the molecules?
(a) The number of collisions per unit area of the container wall is constant. (b) The average kinetic energy doubles. (c) The root mean square speed increases to \(\sqrt{2}\) times its initial value; urms is proportional to \(\sqrt{{\text{KE}}_{\text{avg}}}.\)
The root mean square speed of H2 molecules at 25 °C is about 1.6 km/s. What is the root mean square speed of a N2 molecule at 25 °C?
Answer the following questions:
(a) Is the pressure of the gas in the hot-air balloon shown at the opening of this chapter greater than, less than, or equal to that of the atmosphere outside the balloon?
(b) Is the density of the gas in the hot-air balloon shown at the opening of this chapter greater than, less than, or equal to that of the atmosphere outside the balloon?
(c) At a pressure of 1 atm and a temperature of 20 °C, dry air has a density of 1.2256 g/L. What is the (average) molar mass of dry air?
(d) The average temperature of the gas in a hot-air balloon is 1.30 \(×\) 102 °F. Calculate its density, assuming the molar mass equals that of dry air.
(e) The lifting capacity of a hot-air balloon is equal to the difference in the mass of the cool air displaced by the balloon and the mass of the gas in the balloon. What is the difference in the mass of 1.00 L of the cool air in part (c) and the hot air in part (d)?
(f) An average balloon has a diameter of 60 feet and a volume of 1.1 \(×\) 105 ft3. What is the lifting power of such a balloon? If the weight of the balloon and its rigging is 500 pounds, what is its capacity for carrying passengers and cargo?
(g) A balloon carries 40.0 gallons of liquid propane (density 0.5005 g/L). What volume of CO2 and H2O gas is produced by the combustion of this propane?
(h) A balloon flight can last about 90 minutes. If all of the fuel is burned during this time, what is the approximate rate of heat loss (in kJ/min) from the hot air in the bag during the flight?
(a) equal; (b) less than; (c) 29.48 g mol−1; (d) 1.0966 g L−1; (e) 0.129 g/L; (f) 4.01 \(×\) 105 g; net lifting capacity = 384 lb; (g) 270 L; (h) 39.1 kJ min−1
Show that the ratio of the rate of diffusion of Gas 1 to the rate of diffusion of Gas 2, \(\frac{{R}_{1}}{{R}_{2}},\) is the same at 0 °C and 100 °C.
Glossary
- kinetic molecular theory
- theory based on simple principles and assumptions that effectively explains ideal gas behavior
- root mean square velocity (urms)
- measure of average velocity for a group of particles calculated as the square root of the average squared velocity
[latexpage]
Learning Objectives
By the end of this section, you will be able to:
- Describe the physical factors that lead to deviations from ideal gas behavior
- Explain how these factors are represented in the van der Waals equation
- Define compressibility (Z) and describe how its variation with pressure reflects non-ideal behavior
- Quantify non-ideal behavior by comparing computations of gas properties using the ideal gas law and the van der Waals equation
Thus far, the ideal gas law, PV = nRT, has been applied to a variety of different types of problems, ranging from reaction stoichiometry and empirical and molecular formula problems to determining the density and molar mass of a gas. As mentioned in the previous modules of this chapter, however, the behavior of a gas is often non-ideal, meaning that the observed relationships between its pressure, volume, and temperature are not accurately described by the gas laws. In this section, the reasons for these deviations from ideal gas behavior are considered.
One way in which the accuracy of PV = nRT can be judged is by comparing the actual volume of 1 mole of gas (its molar volume, Vm) to the molar volume of an ideal gas at the same temperature and pressure. This ratio is called the compressibility factor (Z) with:
Ideal gas behavior is therefore indicated when this ratio is equal to 1, and any deviation from 1 is an indication of non-ideal behavior. (Figure) shows plots of Z over a large pressure range for several common gases.
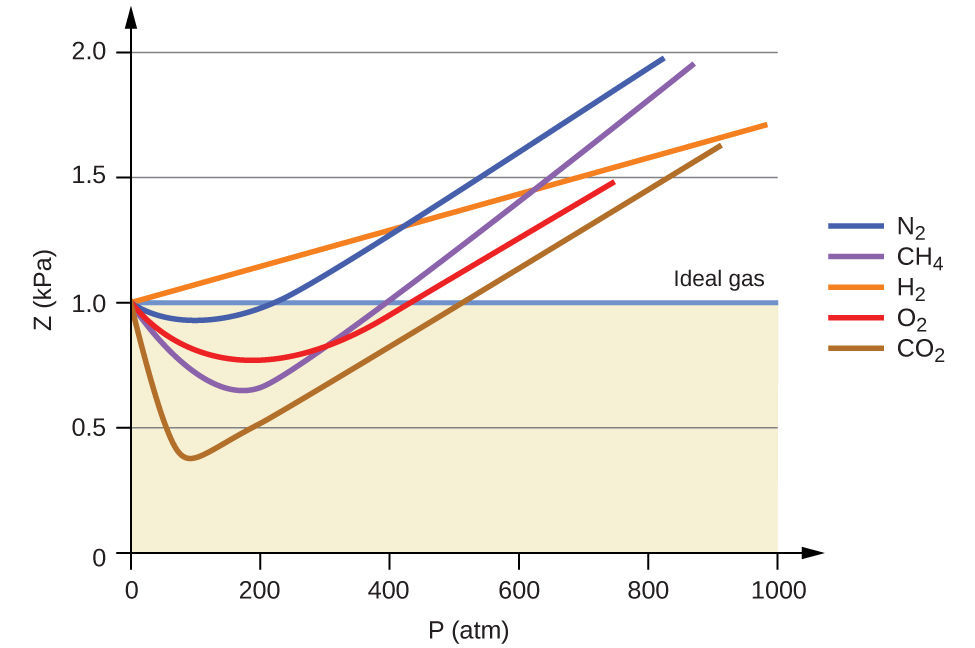
As is apparent from (Figure), the ideal gas law does not describe gas behavior well at relatively high pressures. To determine why this is, consider the differences between real gas properties and what is expected of a hypothetical ideal gas.
Particles of a hypothetical ideal gas have no significant volume and do not attract or repel each other. In general, real gases approximate this behavior at relatively low pressures and high temperatures. However, at high pressures, the molecules of a gas are crowded closer together, and the amount of empty space between the molecules is reduced. At these higher pressures, the volume of the gas molecules themselves becomes appreciable relative to the total volume occupied by the gas. The gas therefore becomes less compressible at these high pressures, and although its volume continues to decrease with increasing pressure, this decrease is not proportional as predicted by Boyle’s law.
At relatively low pressures, gas molecules have practically no attraction for one another because they are (on average) so far apart, and they behave almost like particles of an ideal gas. At higher pressures, however, the force of attraction is also no longer insignificant. This force pulls the molecules a little closer together, slightly decreasing the pressure (if the volume is constant) or decreasing the volume (at constant pressure) ((Figure)). This change is more pronounced at low temperatures because the molecules have lower KE relative to the attractive forces, and so they are less effective in overcoming these attractions after colliding with one another.
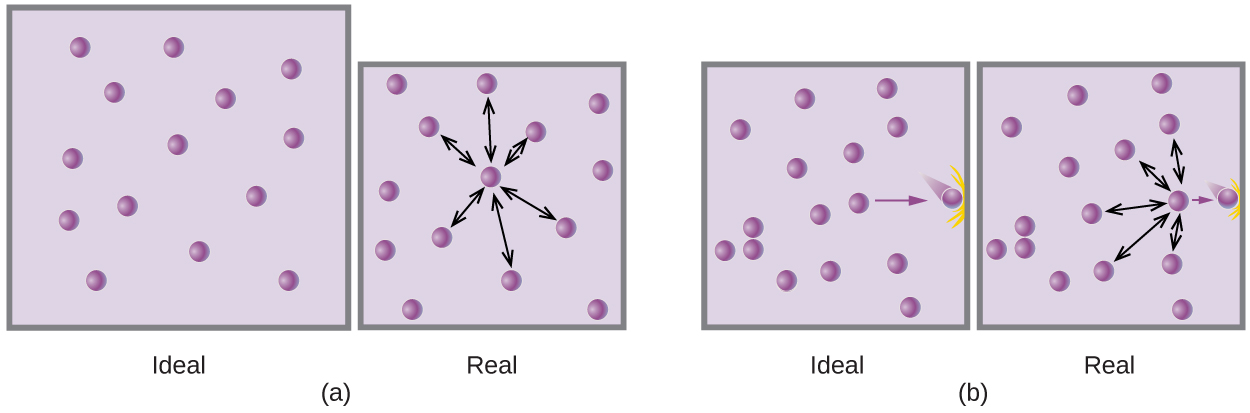
There are several different equations that better approximate gas behavior than does the ideal gas law. The first, and simplest, of these was developed by the Dutch scientist Johannes van der Waals in 1879. The van der Waals equation improves upon the ideal gas law by adding two terms: one to account for the volume of the gas molecules and another for the attractive forces between them.

The constant a corresponds to the strength of the attraction between molecules of a particular gas, and the constant b corresponds to the size of the molecules of a particular gas. The “correction” to the pressure term in the ideal gas law is \(\frac{{n}^{2}a}{{V}^{2}},\) and the “correction” to the volume is nb. Note that when V is relatively large and n is relatively small, both of these correction terms become negligible, and the van der Waals equation reduces to the ideal gas law, PV = nRT. Such a condition corresponds to a gas in which a relatively low number of molecules is occupying a relatively large volume, that is, a gas at a relatively low pressure. Experimental values for the van der Waals constants of some common gases are given in (Figure).
| Values of van der Waals Constants for Some Common Gases | ||
|---|---|---|
| Gas | a (L2 atm/mol2) | b (L/mol) |
| N2 | 1.39 | 0.0391 |
| O2 | 1.36 | 0.0318 |
| CO2 | 3.59 | 0.0427 |
| H2O | 5.46 | 0.0305 |
| He | 0.0342 | 0.0237 |
| CCl4 | 20.4 | 0.1383 |
At low pressures, the correction for intermolecular attraction, a, is more important than the one for molecular volume, b. At high pressures and small volumes, the correction for the volume of the molecules becomes important because the molecules themselves are incompressible and constitute an appreciable fraction of the total volume. At some intermediate pressure, the two corrections have opposing influences and the gas appears to follow the relationship given by PV = nRT over a small range of pressures. This behavior is reflected by the “dips” in several of the compressibility curves shown in (Figure). The attractive force between molecules initially makes the gas more compressible than an ideal gas, as pressure is raised (Z decreases with increasing P). At very high pressures, the gas becomes less compressible (Z increases with P), as the gas molecules begin to occupy an increasingly significant fraction of the total gas volume.
Strictly speaking, the ideal gas equation functions well when intermolecular attractions between gas molecules are negligible and the gas molecules themselves do not occupy an appreciable part of the whole volume. These criteria are satisfied under conditions of low pressure and high temperature. Under such conditions, the gas is said to behave ideally, and deviations from the gas laws are small enough that they may be disregarded—this is, however, very often not the case.
Comparison of Ideal Gas Law and van der Waals Equation A 4.25-L flask contains 3.46 mol CO2 at 229 °C. Calculate the pressure of this sample of CO2:
(a) from the ideal gas law
(b) from the van der Waals equation
(c) Explain the reason(s) for the difference.
Solution (a) From the ideal gas law:
(b) From the van der Waals equation:
This finally yields P = 32.4 atm.
(c) This is not very different from the value from the ideal gas law because the pressure is not very high and the temperature is not very low. The value is somewhat different because CO2 molecules do have some volume and attractions between molecules, and the ideal gas law assumes they do not have volume or attractions.
Check your Learning A 560-mL flask contains 21.3 g N2 at 145 °C. Calculate the pressure of N2:
(a) from the ideal gas law
(b) from the van der Waals equation
(c) Explain the reason(s) for the difference.
(a) 46.562 atm; (b) 46.594 atm; (c) The van der Waals equation takes into account the volume of the gas molecules themselves as well as intermolecular attractions.
Key Concepts and Summary
Gas molecules possess a finite volume and experience forces of attraction for one another. Consequently, gas behavior is not necessarily described well by the ideal gas law. Under conditions of low pressure and high temperature, these factors are negligible, the ideal gas equation is an accurate description of gas behavior, and the gas is said to exhibit ideal behavior. However, at lower temperatures and higher pressures, corrections for molecular volume and molecular attractions are required to account for finite molecular size and attractive forces. The van der Waals equation is a modified version of the ideal gas law that can be used to account for the non-ideal behavior of gases under these conditions.
Key Equations
- \(\text{Z}=\phantom{\rule{0.2em}{0ex}}\frac{\text{molar}\phantom{\rule{0.2em}{0ex}}\text{volume of gas at same}\phantom{\rule{0.2em}{0ex}}T\phantom{\rule{0.2em}{0ex}}\text{and}\phantom{\rule{0.2em}{0ex}}P}{\text{molar volume of ideal gas at same}\phantom{\rule{0.2em}{0ex}}T\phantom{\rule{0.2em}{0ex}}\text{and}\phantom{\rule{0.2em}{0ex}}P}\phantom{\rule{0.2em}{0ex}}={\left(\frac{P\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{V}_{m}}{R\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}T}\right)}_{\text{measured}}\)
- \(\left(P+\frac{{n}^{2}a}{{V}^{2}}\right)\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\left(V-nb\right)=nRT\)
Chemistry End of Chapter Exercises
Graphs showing the behavior of several different gases follow. Which of these gases exhibit behavior significantly different from that expected for ideal gases?
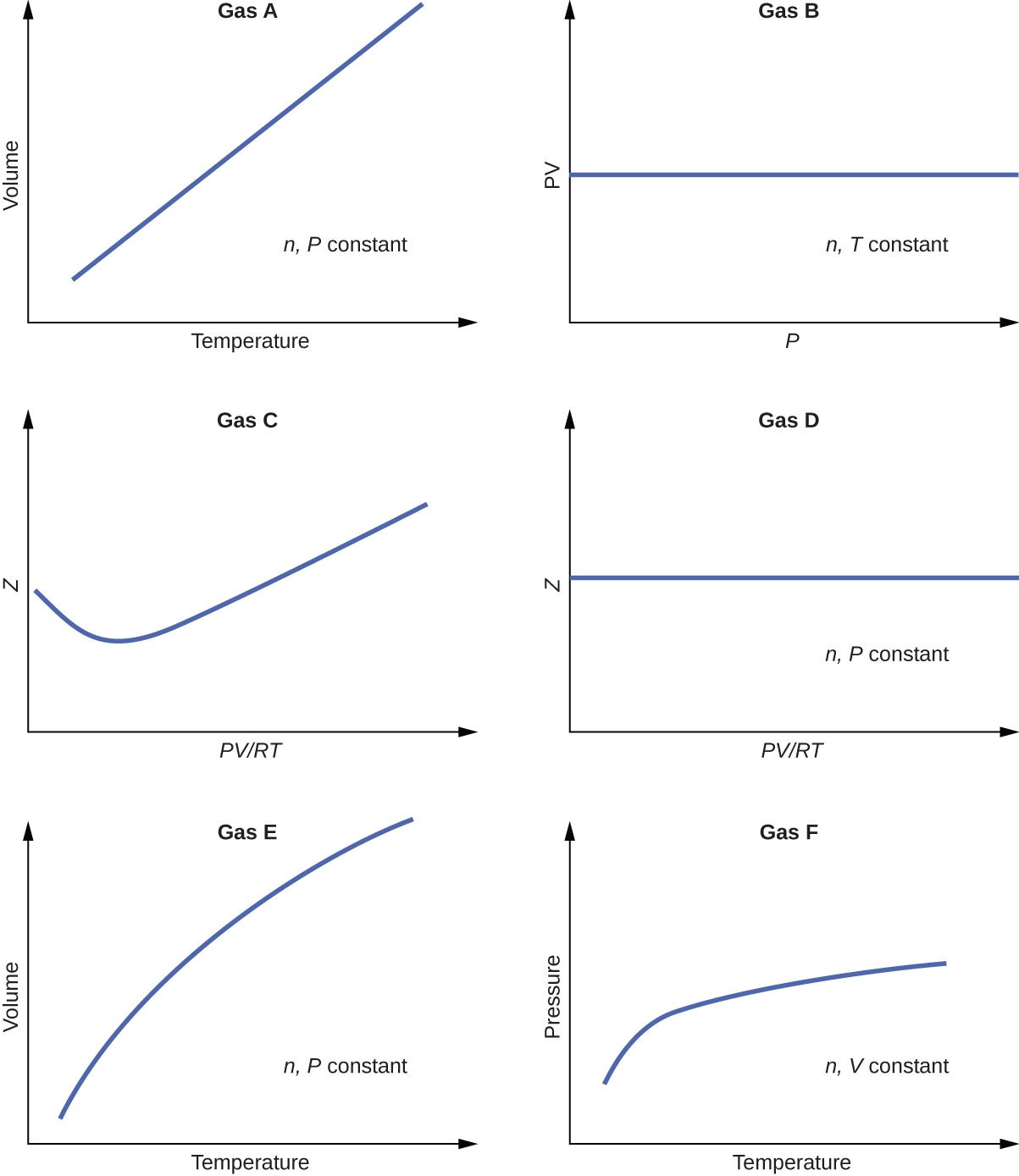
Gases C, E, and F
Explain why the plot of PV for CO2 differs from that of an ideal gas.
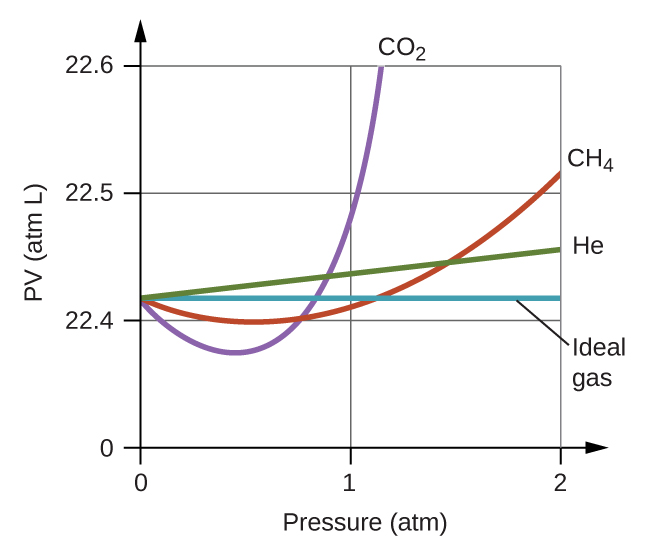
Under which of the following sets of conditions does a real gas behave most like an ideal gas, and for which conditions is a real gas expected to deviate from ideal behavior? Explain.
(a) high pressure, small volume
(b) high temperature, low pressure
(c) low temperature, high pressure
The gas behavior most like an ideal gas will occur under the conditions in (b). Molecules have high speeds and move through greater distances between collision; they also have shorter contact times and interactions are less likely. Deviations occur with the conditions described in (a) and (c). Under conditions of (a), some gases may liquefy. Under conditions of (c), most gases will liquefy.
Describe the factors responsible for the deviation of the behavior of real gases from that of an
ideal gas.
For which of the following gases should the correction for the molecular volume be largest:
CO, CO2, H2, He, NH3, SF6?
SF6
A 0.245-L flask contains 0.467 mol CO2 at 159 °C. Calculate the pressure:
(a) using the ideal gas law
(b) using the van der Waals equation
(c) Explain the reason for the difference.
(d) Identify which correction (that for P or V) is dominant and why.
Answer the following questions:
(a) If XX behaved as an ideal gas, what would its graph of Z vs. P look like?
(b) For most of this chapter, we performed calculations treating gases as ideal. Was this justified?
(c) What is the effect of the volume of gas molecules on Z? Under what conditions is this effect small? When is it large? Explain using an appropriate diagram.
(d) What is the effect of intermolecular attractions on the value of Z? Under what conditions is this effect small? When is it large? Explain using an appropriate diagram.
(e) In general, under what temperature conditions would you expect Z to have the largest deviations from the Z for an ideal gas?
(a) A straight horizontal line at 1.0; (b) When real gases are at low pressures and high temperatures, they behave close enough to ideal gases that they are approximated as such; however, in some cases, we see that at a high pressure and temperature, the ideal gas approximation breaks down and is significantly different from the pressure calculated by the ideal gas equation. (c) The greater the compressibility, the more the volume matters. At low pressures, the correction factor for intermolecular attractions is more significant, and the effect of the volume of the gas molecules on Z would be a small lowering compressibility. At higher pressures, the effect of the volume of the gas molecules themselves on Z would increase compressibility (see (Figure)). (d) Once again, at low pressures, the effect of intermolecular attractions on Z would be more important than the correction factor for the volume of the gas molecules themselves, though perhaps still small. At higher pressures and low temperatures, the effect of intermolecular attractions would be larger. See (Figure). (e) Low temperatures
Glossary
- compressibility factor (Z)
- ratio of the experimentally measured molar volume for a gas to its molar volume as computed from the ideal gas equation
- van der Waals equation
- modified version of the ideal gas equation containing additional terms to account for non-ideal gas behavior
[latexpage]
Learning Objectives
By the end of this section, you will be able to:
- Describe the physical factors that lead to deviations from ideal gas behavior
- Explain how these factors are represented in the van der Waals equation
- Define compressibility (Z) and describe how its variation with pressure reflects non-ideal behavior
- Quantify non-ideal behavior by comparing computations of gas properties using the ideal gas law and the van der Waals equation
Thus far, the ideal gas law, PV = nRT, has been applied to a variety of different types of problems, ranging from reaction stoichiometry and empirical and molecular formula problems to determining the density and molar mass of a gas. As mentioned in the previous modules of this chapter, however, the behavior of a gas is often non-ideal, meaning that the observed relationships between its pressure, volume, and temperature are not accurately described by the gas laws. In this section, the reasons for these deviations from ideal gas behavior are considered.
One way in which the accuracy of PV = nRT can be judged is by comparing the actual volume of 1 mole of gas (its molar volume, Vm) to the molar volume of an ideal gas at the same temperature and pressure. This ratio is called the compressibility factor (Z) with:
Ideal gas behavior is therefore indicated when this ratio is equal to 1, and any deviation from 1 is an indication of non-ideal behavior. (Figure) shows plots of Z over a large pressure range for several common gases.

As is apparent from (Figure), the ideal gas law does not describe gas behavior well at relatively high pressures. To determine why this is, consider the differences between real gas properties and what is expected of a hypothetical ideal gas.
Particles of a hypothetical ideal gas have no significant volume and do not attract or repel each other. In general, real gases approximate this behavior at relatively low pressures and high temperatures. However, at high pressures, the molecules of a gas are crowded closer together, and the amount of empty space between the molecules is reduced. At these higher pressures, the volume of the gas molecules themselves becomes appreciable relative to the total volume occupied by the gas. The gas therefore becomes less compressible at these high pressures, and although its volume continues to decrease with increasing pressure, this decrease is not proportional as predicted by Boyle’s law.
At relatively low pressures, gas molecules have practically no attraction for one another because they are (on average) so far apart, and they behave almost like particles of an ideal gas. At higher pressures, however, the force of attraction is also no longer insignificant. This force pulls the molecules a little closer together, slightly decreasing the pressure (if the volume is constant) or decreasing the volume (at constant pressure) ((Figure)). This change is more pronounced at low temperatures because the molecules have lower KE relative to the attractive forces, and so they are less effective in overcoming these attractions after colliding with one another.

There are several different equations that better approximate gas behavior than does the ideal gas law. The first, and simplest, of these was developed by the Dutch scientist Johannes van der Waals in 1879. The van der Waals equation improves upon the ideal gas law by adding two terms: one to account for the volume of the gas molecules and another for the attractive forces between them.

The constant a corresponds to the strength of the attraction between molecules of a particular gas, and the constant b corresponds to the size of the molecules of a particular gas. The “correction” to the pressure term in the ideal gas law is \(\frac{{n}^{2}a}{{V}^{2}},\) and the “correction” to the volume is nb. Note that when V is relatively large and n is relatively small, both of these correction terms become negligible, and the van der Waals equation reduces to the ideal gas law, PV = nRT. Such a condition corresponds to a gas in which a relatively low number of molecules is occupying a relatively large volume, that is, a gas at a relatively low pressure. Experimental values for the van der Waals constants of some common gases are given in (Figure).
| Values of van der Waals Constants for Some Common Gases | ||
|---|---|---|
| Gas | a (L2 atm/mol2) | b (L/mol) |
| N2 | 1.39 | 0.0391 |
| O2 | 1.36 | 0.0318 |
| CO2 | 3.59 | 0.0427 |
| H2O | 5.46 | 0.0305 |
| He | 0.0342 | 0.0237 |
| CCl4 | 20.4 | 0.1383 |
At low pressures, the correction for intermolecular attraction, a, is more important than the one for molecular volume, b. At high pressures and small volumes, the correction for the volume of the molecules becomes important because the molecules themselves are incompressible and constitute an appreciable fraction of the total volume. At some intermediate pressure, the two corrections have opposing influences and the gas appears to follow the relationship given by PV = nRT over a small range of pressures. This behavior is reflected by the “dips” in several of the compressibility curves shown in (Figure). The attractive force between molecules initially makes the gas more compressible than an ideal gas, as pressure is raised (Z decreases with increasing P). At very high pressures, the gas becomes less compressible (Z increases with P), as the gas molecules begin to occupy an increasingly significant fraction of the total gas volume.
Strictly speaking, the ideal gas equation functions well when intermolecular attractions between gas molecules are negligible and the gas molecules themselves do not occupy an appreciable part of the whole volume. These criteria are satisfied under conditions of low pressure and high temperature. Under such conditions, the gas is said to behave ideally, and deviations from the gas laws are small enough that they may be disregarded—this is, however, very often not the case.
Comparison of Ideal Gas Law and van der Waals Equation A 4.25-L flask contains 3.46 mol CO2 at 229 °C. Calculate the pressure of this sample of CO2:
(a) from the ideal gas law
(b) from the van der Waals equation
(c) Explain the reason(s) for the difference.
Solution (a) From the ideal gas law:
(b) From the van der Waals equation:
This finally yields P = 32.4 atm.
(c) This is not very different from the value from the ideal gas law because the pressure is not very high and the temperature is not very low. The value is somewhat different because CO2 molecules do have some volume and attractions between molecules, and the ideal gas law assumes they do not have volume or attractions.
Check your Learning A 560-mL flask contains 21.3 g N2 at 145 °C. Calculate the pressure of N2:
(a) from the ideal gas law
(b) from the van der Waals equation
(c) Explain the reason(s) for the difference.
(a) 46.562 atm; (b) 46.594 atm; (c) The van der Waals equation takes into account the volume of the gas molecules themselves as well as intermolecular attractions.
Key Concepts and Summary
Gas molecules possess a finite volume and experience forces of attraction for one another. Consequently, gas behavior is not necessarily described well by the ideal gas law. Under conditions of low pressure and high temperature, these factors are negligible, the ideal gas equation is an accurate description of gas behavior, and the gas is said to exhibit ideal behavior. However, at lower temperatures and higher pressures, corrections for molecular volume and molecular attractions are required to account for finite molecular size and attractive forces. The van der Waals equation is a modified version of the ideal gas law that can be used to account for the non-ideal behavior of gases under these conditions.
Key Equations
- \(\text{Z}=\phantom{\rule{0.2em}{0ex}}\frac{\text{molar}\phantom{\rule{0.2em}{0ex}}\text{volume of gas at same}\phantom{\rule{0.2em}{0ex}}T\phantom{\rule{0.2em}{0ex}}\text{and}\phantom{\rule{0.2em}{0ex}}P}{\text{molar volume of ideal gas at same}\phantom{\rule{0.2em}{0ex}}T\phantom{\rule{0.2em}{0ex}}\text{and}\phantom{\rule{0.2em}{0ex}}P}\phantom{\rule{0.2em}{0ex}}={\left(\frac{P\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{V}_{m}}{R\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}T}\right)}_{\text{measured}}\)
- \(\left(P+\frac{{n}^{2}a}{{V}^{2}}\right)\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\left(V-nb\right)=nRT\)
Chemistry End of Chapter Exercises
Graphs showing the behavior of several different gases follow. Which of these gases exhibit behavior significantly different from that expected for ideal gases?

Gases C, E, and F
Explain why the plot of PV for CO2 differs from that of an ideal gas.

Under which of the following sets of conditions does a real gas behave most like an ideal gas, and for which conditions is a real gas expected to deviate from ideal behavior? Explain.
(a) high pressure, small volume
(b) high temperature, low pressure
(c) low temperature, high pressure
The gas behavior most like an ideal gas will occur under the conditions in (b). Molecules have high speeds and move through greater distances between collision; they also have shorter contact times and interactions are less likely. Deviations occur with the conditions described in (a) and (c). Under conditions of (a), some gases may liquefy. Under conditions of (c), most gases will liquefy.
Describe the factors responsible for the deviation of the behavior of real gases from that of an
ideal gas.
For which of the following gases should the correction for the molecular volume be largest:
CO, CO2, H2, He, NH3, SF6?
SF6
A 0.245-L flask contains 0.467 mol CO2 at 159 °C. Calculate the pressure:
(a) using the ideal gas law
(b) using the van der Waals equation
(c) Explain the reason for the difference.
(d) Identify which correction (that for P or V) is dominant and why.
Answer the following questions:
(a) If XX behaved as an ideal gas, what would its graph of Z vs. P look like?
(b) For most of this chapter, we performed calculations treating gases as ideal. Was this justified?
(c) What is the effect of the volume of gas molecules on Z? Under what conditions is this effect small? When is it large? Explain using an appropriate diagram.
(d) What is the effect of intermolecular attractions on the value of Z? Under what conditions is this effect small? When is it large? Explain using an appropriate diagram.
(e) In general, under what temperature conditions would you expect Z to have the largest deviations from the Z for an ideal gas?
(a) A straight horizontal line at 1.0; (b) When real gases are at low pressures and high temperatures, they behave close enough to ideal gases that they are approximated as such; however, in some cases, we see that at a high pressure and temperature, the ideal gas approximation breaks down and is significantly different from the pressure calculated by the ideal gas equation. (c) The greater the compressibility, the more the volume matters. At low pressures, the correction factor for intermolecular attractions is more significant, and the effect of the volume of the gas molecules on Z would be a small lowering compressibility. At higher pressures, the effect of the volume of the gas molecules themselves on Z would increase compressibility (see (Figure)). (d) Once again, at low pressures, the effect of intermolecular attractions on Z would be more important than the correction factor for the volume of the gas molecules themselves, though perhaps still small. At higher pressures and low temperatures, the effect of intermolecular attractions would be larger. See (Figure). (e) Low temperatures
Glossary
- compressibility factor (Z)
- ratio of the experimentally measured molar volume for a gas to its molar volume as computed from the ideal gas equation
- van der Waals equation
- modified version of the ideal gas equation containing additional terms to account for non-ideal gas behavior
[latexpage]
Learning Objectives
- Energy Basics
- Calorimetry
- Enthalpy

Chemical reactions, such as those that occur when you light a match, involve changes in energy as well as matter. Societies at all levels of development could not function without the energy released by chemical reactions. In 2012, about 85% of US energy consumption came from the combustion of petroleum products, coal, wood, and garbage. We use this energy to produce electricity (38%); to transport food, raw materials, manufactured goods, and people (27%); for industrial production (21%); and to heat and power our homes and businesses (10%).1 While these combustion reactions help us meet our essential energy needs, they are also recognized by the majority of the scientific community as a major contributor to global climate change.
Useful forms of energy are also available from a variety of chemical reactions other than combustion. For example, the energy produced by the batteries in a cell phone, car, or flashlight results from chemical reactions. This chapter introduces many of the basic ideas necessary to explore the relationships between chemical changes and energy, with a focus on thermal energy.
The chemical bond is simply another form of energy, and its strength is indicated in exactly the same units as any other process involving energy. That is, in joules (or kilojoules) – or, when taken on a molar basis, in J/mol or kJ/mol. All of the principles involving energy we have examined up to now apply to bond energies exactly as they do to all other forms of energy: that is, energy may change form or be absorbed or released, but it cannot be created or destroyed. The energy of a chemical bond is indicated by the bond enthalpy, taken by convention to be the energy required to break a chemical bond. The same energy is released when a chemical bond is formed. Given that a chemical reaction involves breaking some bonds and making others, the enthalpy change for a chemical reaction can be estimated by analyzing the bonds broken and formed during the reaction. While this procedure gives an estimate of the overall enthalpy, it should be noted that a far more precise method involves using enthalpies of formation; the fact that there can be significant differences between, for example, an O–H bond in water and that in acetic acid accounts for the discrepancy in calculating ΔH using bond enthalpies versus the use of enthalpies of formation.
Footnotes
- 1 US Energy Information Administration, Primary Energy Consumption by Source and Sector, 2012, http://www.eia.gov/totalenergy/data/monthly/pdf/flow/css_2012_energy.pdf. Data derived from US Energy Information Administration, Monthly Energy Review (January 2014).
[latexpage]
Learning Objectives
- Energy Basics
- Calorimetry
- Enthalpy

Chemical reactions, such as those that occur when you light a match, involve changes in energy as well as matter. Societies at all levels of development could not function without the energy released by chemical reactions. In 2012, about 85% of US energy consumption came from the combustion of petroleum products, coal, wood, and garbage. We use this energy to produce electricity (38%); to transport food, raw materials, manufactured goods, and people (27%); for industrial production (21%); and to heat and power our homes and businesses (10%).1 While these combustion reactions help us meet our essential energy needs, they are also recognized by the majority of the scientific community as a major contributor to global climate change.
Useful forms of energy are also available from a variety of chemical reactions other than combustion. For example, the energy produced by the batteries in a cell phone, car, or flashlight results from chemical reactions. This chapter introduces many of the basic ideas necessary to explore the relationships between chemical changes and energy, with a focus on thermal energy.
The chemical bond is simply another form of energy, and its strength is indicated in exactly the same units as any other process involving energy. That is, in joules (or kilojoules) – or, when taken on a molar basis, in J/mol or kJ/mol. All of the principles involving energy we have examined up to now apply to bond energies exactly as they do to all other forms of energy: that is, energy may change form or be absorbed or released, but it cannot be created or destroyed. The energy of a chemical bond is indicated by the bond enthalpy, taken by convention to be the energy required to break a chemical bond. The same energy is released when a chemical bond is formed. Given that a chemical reaction involves breaking some bonds and making others, the enthalpy change for a chemical reaction can be estimated by analyzing the bonds broken and formed during the reaction. While this procedure gives an estimate of the overall enthalpy, it should be noted that a far more precise method involves using enthalpies of formation; the fact that there can be significant differences between, for example, an O–H bond in water and that in acetic acid accounts for the discrepancy in calculating ΔH using bond enthalpies versus the use of enthalpies of formation.
Footnotes
- 1 US Energy Information Administration, Primary Energy Consumption by Source and Sector, 2012, http://www.eia.gov/totalenergy/data/monthly/pdf/flow/css_2012_energy.pdf. Data derived from US Energy Information Administration, Monthly Energy Review (January 2014).

